State Board Commerce (XI-XII) - Test Papers
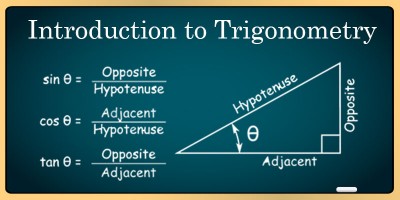
NCERT Solutions for Class 10 Maths – Introduction to Trigonometry
Exercise 8.1
Q1) In △ABC , 90∘ at B, AB=24cm, BC = 7cm.
Determine:
(i)sin(A), cos(A)
(ii) sin(C), cos(C)
Ans.) In △ABC , ∠B=90∘
By Applying Pythagoras theorem, we get
AC2=AB2+BC2
(24)2+72 =(576+49)
AC2 = 625cm2
à AC = 25cm
(i) sin(A) = BC/AC = 7/25
Cos(A) = AB/AC = 24/25
(ii) sin(C) = AB/AC =24/25
cos(C) = BC/AC = 7/25
Q2) In the given figure find tan(P) – cot(R)
Ans.) PR = 13cm,PQ = 12cm and QR = 5cm
According to Pythagorean theorem,
132=QR2+122 169=QR2+144 QR2=169−144=25 QR=25−−√=5
tan(P) = oppositesideadjacentside=QRPQ=512
cot(P) = adjacentsideoppositeside = PQQR = 512
tan(P) – cot(R) = 512−512=0
Therefore ,tan(P) – cot(R) = 0
Q3) If sin(A) = 3/4, calculate cos(A) and tan(A)
Ans.) Let △ABC , be a right-angled triangle, right-angled at B.
We know that sin(A) = BC/AC = 3/4
Let BC be 3k and AC will be 4k where k is a positive real number.
By Pythagoras theorem we get,
AC2=AB2+BC2
(4k)2=AB2+(3k)2
16k2−9k2=AB2
AB2=7k2
AB=7–√k
cos(A) = AB/AC = 7–√k/4k=7–√/4
tan(A) = BC/AB =3k/7–√=3/7–√
Q4) In question given below 15cot(A) = 8 ,find sin A and sec A.
Ans.) Let △ABC be a right angled triangle, right-angled at B.
We know that cot(A) = AB/BC = 8/15
Given
Let AB side be 8k and BC side 15k
Where k is positive real number
By Pythagoras theorem we get,
AC2=AB2+BC2
AC2=(8k)2+(15k)2
AC2=64k2+225k2
AC2=289k2
AC = 17k
sin(A) = BC/AC = 15k/17k = 15/7
sec(A) =AC/AB =17k/8k = 17/8
Q5) Given sec Ѳ =13/12, calculate all other trigonometric ratios.
Ans.) Let △ABC be right-angled triangle, right-angled at B.
We know that sec Ѳ =OP/OM =13/12(Given)
Let side OP be 13k and side OM will be 12k where k is positive real number.
By Pythagoras theorem we get,
OP2=OM2+MP2
(13k)2=(12k)2+MP2
169(k)2−144(k)2=MP2
MP2=25k2
MP = 5
Now,
sin Ѳ = MP/OP = 5k/13k =5/13
cos Ѳ = OM/OP = 12k/13k = 12/13
tan Ѳ = MP/OM = 5k/12k = 5/12
cot Ѳ = OM/MP = 12k/5k = 12/5
cosec Ѳ = OP/MP = 13k/5k = 13/5
Q6) If ∠A and ∠B are acute angles such that
cos(A) = cos(B), then show ∠A =∠B .
Ans.) Let △ABC in which CD⊥AB .
A/q,
cos(A) = cos(B)
à AD/AC = BD/BC
à AD/BD = AC/BC
Let AD/BD =AC/BC =k
AD =kBD …. (i)
AC=kBC …. (ii)
By applying Pythagoras theorem in △CAD and △CBD we get,
CD2=AC2−AD2 ….(iv)
From the equations (iii) and (iv) we get,
AC2−AD2=BC2−BD2 AC2−AD2=BC2−BD2 k2(BC2−BD2)=BC2−BD2 k2=1
Putting this value in equation (ii) , we obtain
AC = BC
∠A=∠B (Angles opposite to equal side are equal-isosceles triangle)
Q7) If cot Ѳ = 7/8, evaluate :
(i) (1+sin Ѳ)(1-sin Ѳ) / (1+cos Ѳ)(1-cos Ѳ)
(ii) cot2Θ
Ans.) Let △ABC in which ∠B=90∘
and ∠C=Θ
A/q,
cot Ѳ =BC/AB = 7/8
Let BC = 7k and AB = 8k, where k is a positive real number
According to Pythagoras theorem in △ABC we get.
AC2=AB2+BC2
AC2=(8k)2+(7k)2
AC2=64k2+49k2
AC2=113k2
AC=113−−−√k
sin Ѳ = AB/AC = 8k/113−−−√k=8/113−−−√
and cos Ѳ = BC/AC = 7k/113−−−√k=7/113−−−√
(i) (1+sin Ѳ)(1-sinѲ)/(1+cosѲ)(1-cos Ѳ) = (1−sin2Θ)/(1−cos2Θ)
= 1−(8/113−−−√)2/1−(7/113−−−√)2
= {1-(64/113)}/{1-(49/113)} = {(113-64)/113}/{(113-49)/113} = 49/64
(ii) cot2Θ=(7/8)2=49/64
Q8) If 3cot(A) = 4/3, check whether (1−tan2A)/(1+tan2A)=cos2A−sin2A or not.
Ans.) Let △ABC in which ∠B=90∘
A/q,
cot(A) = AB/BC = 4/3
Let AB = 4k an BC =3k, where k is a positive real number.
AC2=AB2+BC2
AC2=(4k)2+(3k)2
AC2=16k2+9k2
AC2=25k2
AC=5k
tan(A) = BC/AB = 3/4
sin(A) = BC/AC = 3/5
cos(A) = AB/AC = 4/5
L.H.S. = (1−tan2A)(1+tan2A)=1−(3/4)2/1+(3/4)2=(1−9/16)/(1+9/16)=(16−9)/(16+9)=7/25
R.H.S. =cos2A−sin2A=(4/5)2−(3/4)2=(16/25)−(9/25)=7/25
R.H.S. =L.H.S.
Hence, (1−tan2A)/(1+tan2A)=cos2A−sin2A
Q9) In triangle EFG, right-angled at F, if tan E =1/√3 find the value of:
(i) sin EcosG + cosE sin G
(ii) cosEcosG – sin E sin G
Answer
LetΔEFG in which ∠F=90∘, E/q
tanE=FCEF tanE=FCEF=13√
Where k is the positive real number of the problem
By Pythagoras theorem in ΔEFG we get:
EG2=EF2+FG2 EG2=(3k−−√2))+K2 EG2=3k2+K2 EG2=4k2 EG=2K
sinE = FG/EG = 1/2
cosE = EF/EG = 3√2 ,
sin G = EF/EG = 3√2 cosE = FG/EG = 1/2
(i) sin EcosG + cosE sin G = (1/2\ast1/2) + (3√2∗3√2)= 1/4+3/4 = 4/4 = 1
(ii) cosEcosG – sin E sin C = (3√2∗12)−(3√2∗12)= (3√4)−(3√4)= 0
Q10)In Δ MNO, right-angled at N, MO + NO = 25 cm and MN = 5 cm. Determine the values of sin M, cos M and tan M.
Answer
Given that, MO + NO = 25 , MN = 5
Let MO be x. ∴ NO = 25 – x
By Pythagoras theorem ,
MO2=MN2+NO2
X2=52+(25−x)2
50x = 650
x = 13
∴ MO = 13 cm
NO = (25 – 13) cm = 12 cm
sinM = NO/MO = 12/13
cosM = MN/MO = 5/13
tanM = NO/MN = 12/5
Q11) State whether the following are true or false. Justify your answer.
(i) The value of tan M is always less than 1.
(ii) secM = 12/5 for some value of angle M.
(iii) cosM is the abbreviation used for the cosecant of angle M.
(iv) cot M is the product of cot and M.
(v) sin θ = 4/3 for some angle θ.
Answer
(i) False.
In ΔMNC in which ∠N = 90∘,
MN = 3, NC = 4 and MC = 5
Value of tan M = 4/3 which is greater than.
The triangle can be formed with sides equal to 3, 4 and hypotenuse = 5 as
it will follow the Pythagoras theorem.
MC2=MN2+NC2
52=32+42
25 = 9 + 16
25 = 25
(ii) True.
Let a ΔMNC in which ∠N = 90º,MC be 12k and MB be 5k, where k is a positive real number.
By Pythagoras theorem we get,
MC2=MN2+NC2
(12k)2=(5k)2+NC2
NC2+25k2=144K2
NC2=119k2
Such a triangle is possible as it will follow the Pythagoras theorem.
(iii) False.
Abbreviation used for cosecant of angle M is cosec M.cosM is the abbreviation used for cosine of angle M.
(iv) False.
cotM is not the product of cot and M. It is the cotangent of ∠M.
(v) False.
sinΘ = Height/Hypotenuse
We know that in a right angled triangle, Hypotenuse is the longest side.
∴ sinΘwill always less than 1 and it can never be 4/3 for any value of Θ.
Exercise 8.2
1) Calculate the following:
- sin60∘cos30∘+sin30∘cos60∘
- 2tan245∘+co230∘−sin260∘
- cos45∘(sec30∘+cosec30∘)
- (sin30∘+tan45∘−cosec60∘)(sec30∘+cos60∘+cot45∘)
- (5cos260∘+4sec230∘−tan245∘)(sin230∘+cos230∘)
Ans.- (i) sin60∘cos30∘+sin30∘cos60∘
= (3√2×3√2)+(12×12)=34+14=44=1
(ii) 2tan245∘+co230∘−sin260∘
=2×(1)2+(3√2)2−(3√2)2=2
(iii) cos45∘(sec30∘+cosec30∘)
= 12√23√+2=12√(2+23√)3√
= 3√2√×(2+23√)=3√22√+26√
= √3(26√−22√)(26√+22√)(26√−22√)
= 23√(6√−2√)(26√2 −(22√)2)
23√(6√−2√)24−8=23√(6√−2√)16
3√(6√−2√)8=(18√−6√)8=(32√−6√)8
(iv) (sin 30° + tan 45° – cosec 60°)/(sec 30° + cos 60° + cot 45°)
= (12+1–23√23√+12+1)
= (32–23√32+23√)
= (33√–4)2(33√)2–42
= (27+16–243√)(27–16)
= (43–243√)11
(v) (5cos260° + 4sec230° – tan245°)/(sin230° + cos230°)
= 5(12)2+4(23√)2–12(12)2+(3√2)2
= (54+163–1)(14+34)
= (15+64–12)1244
=6712
2) Find the correct answer and explain your choice:
(i) 2tan30∘1+tan230∘ =
(A) sin 60∘ (B) cos 60∘ (C) tan 60∘ (D) sin 30∘
(ii) 1−tan245∘1+tan230∘ =
tan 90∘ (B) 1 (C) sin 45∘ (D) 0
(iii) sin 2P = 2 sin P is true when P =
0∘ (B) 30∘ (C) 45∘ (D) 60∘
(iv) 2tan30∘1−tan230∘ =
cos 60∘ (B) sin 60∘ (C) tan 60∘ (D) sin 30∘
Ans.-
(i) (A) IS correct.
2tan30∘1+tan230∘ = 2(1)3√1+(13√)2
(23√)1+13=(23√)43 =643√=3√2=sin60∘
(ii)(D) is correct
1−tan245∘1+tan230∘
= (1–12)(1+12)=02=0
(iii) (A) is correct
sin 2P = 2 sin P is true when
P = sin 2P = sin 0° = 0
2 sin P = 2sin 0° = 2×0 = 0
or,
sin 2P = 2sin PcosP
=>2sin PcosP = 2 sin P
=>2cos P = 2 =>cosP = 1
=>P = 0°
(iv) (C) is correct
2tan30∘1–tan230∘=2(13√1–(13√)2)
(23√)1–13=23√23=3–√=tan60∘
3) If tan (P + Q) = 3–√ and tan ( P – Q) = 13√;00<P+Q<=90∘;P>Q
, calculate P and Q
Ans:- tan (P + Q) = 3–√
=>tan (P + Q) = tan 60°
=> (P + Q) = 60° … (i)
=>tan (P – Q) = 13√
=>tan (P – Q) = 30°
=> (P – Q) = 30° … (ii)
Adding (i) and (ii), we get
P + Q + P – Q = 60° + 30°
2P = 90°
=> P = 45°
Putting the value of P in equation (i)
45° + Q = 60°
=> Q = 60° – 45° = 15°
Hence, P = 45° and Q = 15°
4) Check whether the given statements are true or false, also give a reason for your answer:
(i) sin (P + Q) = sin P + sin Q.
(ii) The value of sin θ increases as θ increases.
(iii) The value of cos θ increases as θ increases.
(iv) sin θ = cos θ for all values of θ.
(v) cotP is not defined for P = 0°.
Ans:-
(i) False
Let P = 30° and Q = 60°, then
sin (P + Q) = sin (30° + 60°) = sin 90° = 1 and,
sin P + sin Q = sin 30° + sin 60°
= 12+3√2=1+3√2
(ii) True
Sin 0° = 0
Sin 30° = 12
Sin 45° = 12√
Sin 60° = 3√2
Sin 90° = 1
Thus, the value of sinθ increases as θ increases
(iii) False
Cos 0° = 1
Cos 30° = 3√2
Cos 45° = 12√
Cos 60° = 12
Cos 90° = 0
Thus, the value of Cosθ decreases as θ increases.
(iv) True
cotP=cosPSinP cot0∘=cos0∘Sin0∘=10=notdefined
Exercise 8.3
1) Calculate:
(i) sin18∘cos72∘
(ii) tan26∘cot64∘
(iii) cos 48° – sin 42°
(iv) cosec 31° – sec 59°
Ans:-
(i) sin18∘cos72∘
= sin(90∘–18∘)cos72∘
= cos72∘cos72∘=1
(ii) tan26∘cot64∘
= tan(90∘–36∘)cot64∘
= cot64∘cot64∘=1
(iii) cos 48° – sin42°
= cos (90° – 42°) – sin 42°
= sin 42° – sin 42° = 0
(iv) cosec 31° – sec 59°
= cosec (90° – 59°) – sec 59°
= sec 59° – sec 59° = 0
2) Show that :
(i) tan 48° tan 23° tan 42° tan 67° = 1
(ii) cos 38° cos 52° – sin 38° sin 52° = 0
Ans:-
(i)tan 48° tan 23° tan 42° tan 67°
= tan (90° – 42°) tan (90° – 67°) tan 42° tan 67°
= cot 42° cot 67° tan 42° tan 67°
= (cot 42° tan 42°) (cot 67° tan 67°) = 1×1 = 1
(ii) cos 38° cos 52° – sin 38° sin 52°
= cos (90° – 52°) cos (90°-38°) – sin 38° sin 52°
= sin 52° sin 38° – sin 38° sin 52° = 0
3) We have 2P = cot ( P – 18 ° ), where 2P is an acute angle, calculate the value of P.
Ans:- According to question,
tan 2P = cot (P- 18°)
=>cot (90° – 2P) = cot (P -18°)
Equating angles,
=>90° – 2P = P- 18°
=>108° = 3P
=> P = 36
4) If tan P = cot Q, prove that P + Q = 90°.
AnswerAccording to question,
tanP = cot Q
=>tan P = tan (90° – Q)
=>P = 90° – Q
=>P + Q = 90°
5) If the value of sec 4P = cosec (P – 20°), in which 4P is an acute angle, find the value of P.
Ans:-According to question
sec 4P = cosec (P – 20°)
=> cosec (90° – 4P) = cosec (P – 20°)
Equating angles,
=> 90° – 4P= P- 20°
=> 110° = 5P
=> P = 22°
Q6) If X,Y and Z are interior angles of a triangle XYZ, then show that
sin (Y+Z/2) = cos X2
Answer
In a triangle, sum of all the interior angles
X + Y + Z = 180∘
⇒ Y + Z = 180∘ – X
⇒ Y+Z2 = (180∘−X)2
⇒ Y+Z2 = (90∘−X2)
⇒ sin (Y+Z2) = sin (90∘−X2)
⇒ sin (Y+Z2) = cosX2
Q7) Express sin 67∘ + cos 75∘ in terms of trigonometric ratios of angles between 0∘ and 45∘.
Answer
sin 67∘ + cos 75∘
= sin (90∘ – 23∘) + cos (90∘ – 15∘)
= cos 23∘ + sin 15∘
Excercise 8.4
Q1) Express the trigonometric ratios sin A, sec A and tan A in terms of cot A.
Answer
cosec2A−cot2A=1
⇒ cosec2A = 1 + cot2A
⇒ 1sin2A = 1 + cot2A
⇒ sin2A = 1/(1+cot2A)
⇒ sin A= ±11+cot2A√
Now,
sin2A=11+cot2A
⇒1−cos2A=11+cot2A
⇒ cos2A = 1−11+cot2A
⇒cos2A = (1−1+cot2A)(1+cot2A)
⇒1sec2A = (cot2A)(1+cotA)
⇒secA = (1+cotA)(cot2A)
⇒secA=±1+cot2A√cotA
also,
tan A = sinAcosAand cot A = cosAsinA
⇒ tan A = 1cotA
Q2) Write all the other trigonometric ratios of ∠A in terms of sec A.
Answer
We know that,
sec A = 1cosA
⇒cos A = 1secA
also,
cos2A + sin2A = 1
⇒ sin2A = 1 – cos2A
⇒ sin2A = 1 – (1sec2A)
⇒ sin2A = (sec2A−1)sec2A
⇒ sin A=±sec2A−1√secA
also,
sin A = 1cosecA
⇒ cosec A = 1sinA
⇒cosec A=±secAsec2A−1√
Now,
sec2A – tan2A = 1
⇒ tan2A = sec2A + 1
⇒ tan A=sec2A+1−−−−−−−−√
also,
tan A = 1cotA
⇒ cot A = 1tanA
⇒ cot A=±1sec2A+1√
Q3 Evaluate :
(i) (sin263∘+sin227∘)(cos217∘+cos273∘)
(ii) sin25∘cos65+∘+cos25∘sin65∘
Answer
(i) (sin263∘+sin227∘)(cos217∘+cos273∘)
= [sin2(90∘–27∘)+sin227∘][cos2(90∘–73∘)+cos273∘]
=(cos227∘+sin227∘)(sin227∘+cos273∘)
= 11 =1 ( becausesin2A+cos2A=1)
(ii) sin25∘cos65+∘+cos25∘sin65∘
=sin(90∘−25∘)cos65∘+cos(90∘−65∘)sin65∘
=cos65∘cos65∘+sin65∘sin65∘
= cos65∘+sin65∘=1
4) Choose the correct option. Justify your choice.
(i) 9 sec2A – 9 tan2A =
(A) 1 (B) 9 (C) 8 (D) 0
(ii) (1 + tan Θ + sec Θ) (1 + cot Θ – cosec Θ)
(A) 0 (B) 1 (C) 2 (D) – 1
(iii) (secA + tanA) (1 – sinA) =
(A) secA (B) sinA (C) cosecA (D) cosA
(iv) 1+tan2A1+cot2A=
(A) sec2A
(B) -1
(C) cot2A
(D) tan2A
Answer
(i) (B) is correct.
9 sec2A– 9 tan2A
= 9 (sec2A– tan2A )
= 9×1 = 9 ( because sec2A– tan2A = 1)
(ii) (C) is correct
(1 + tan θ + sec θ) (1 + cot θ – cosec θ)
= (1 + sin θ/cos θ + 1/cos θ) (1 + cos θ/sin θ – 1/sin θ)
= (cosθ+sin θ+1)/cos θ × (sin θ+cos θ-1)/sin θ
= (cosθ+sin θ)2-12/(cos θ sin θ)
= (cos2θ + sin2θ + 2cos θ sin θ -1)/(cos θ sin θ)
= (1+ 2cos θ sin θ -1)/(cos θ sin θ)
= (2cos θ sin θ)/(cos θ sin θ) = 2
(iii) (D) is correct.
(secA + tanA) (1 – sinA)
= (1/cos A + sin A/cos A) (1 – sinA)
= (1+sin A/cos A) (1 – sinA)
= (1 – sin2A)/cos A
= cos2A/cos A = cos A
(iv) (D) is correct.
1+tan2A/1+cot2A
= (1+1/cot2A)/1+cot2A
= (cot2A+1/cot2A)×(1/1+cot2A)
= 1/cot2A = tan2A
Q5) Prove the following identities, where the angles involved are acute angles for which theexpressions are defined.
(i) (cosec θ – cot θ)2 = (1-cos θ)/(1+cos θ)
(ii) cos A/(1+sin A) + (1+sin A)/cos A = 2 sec A
(iii) tan θ/(1-cot θ) + cot θ/(1-tan θ) = 1 + sec θ cosec θ
[Hint : Write the expression in terms of sin θ and cos θ]
(iv) (1 + sec A)/sec A = sin2A/(1-cos A)
[Hint : Simplify LHS and RHS separately]
(v) (cos A–sin A+1)/(cosA+sin A–1) = cosec A + cot A,using the identity cosec2A = 1+cot2A.
(vi)1+sinA1−sinA−−−−−√=secA+tanA
(vii) (sin θ – 2sin3θ)/(2cos3θ-cos θ) = tan θ
(viii) (sin A + cosec A)2 + (cos A + sec A)2 = 7+tan2A+cot2A
(ix) (cosec A – sin A)(sec A – cos A) = 1/(tan A+cotA)
[Hint : Simplify LHS and RHS separately]
(x) (1+tan2A/1+cot2A) = (1-tan A/1-cot A)2 = tan2A
Answer
(i) (cosecΘ−cotΘ)2 = (1-cos θ)/(1+cos θ)
L.H.S. = (cosecΘ−cotΘ)2
=(cosec2Θ+cot2Θ−2cosecΘcotΘ)
=(1sin2Θ+cos2Θsin2Θ−2cosΘsin2Θ)
= (1 + cos2Θ – 2cos θ)/(1 – cos2Θ)
= (1−cosΘ)2 /(1 – cosθ)(1+cos θ)
= (1-cos θ)/(1+cos θ) = R.H.S.
(ii) cos A/(1+sin A) + (1+sin A)/cos A = 2 sec A
L.H.S. = cos A/(1+sin A) + (1+sin A)/cos A
= [cos2A +(1+sinA)2]/(1+sin A)cos A
= (cos2A + sin2A + 1 + 2sin A)/(1+sin A)cos A
= (1 + 1 + 2sin A)/(1+sin A)cos A
= (2+ 2sin A)/(1+sin A)cos A
= 2(1+sin A)/(1+sin A)cos A
= 2/cos A = 2 sec A = R.H.S.
(iii) tan θ/(1-cot θ) + cot θ/(1-tan θ) = 1 + sec θ cosec θ
L.H.S. = tan θ/(1-cot θ) + cot θ/(1-tan θ)
= [(sin θ/cos θ)/1-(cos θ/sin θ)] + [(cos θ/sin θ)/1-(sin θ/cos θ)]
= [(sin θ/cos θ)/(sin θ-cos θ)/sin θ] + [(cos θ/sin θ)/(cosθ-sin θ)/cos θ]
= sin2Θ /[cos θ(sin θ-cos θ)] + cos2Θ /[sin θ(cos θ-sin θ)]
= sin2Θ /[cos θ(sin θ-cos θ)] – cos2Θ /[sin θ(sin θ-cos θ)]
= 1/(sin θ-cos θ) [(sin2Θ /cos θ) – (cos2Θ /sin θ)]
= 1/(sin θ-cos θ) × [(sin3Θ – cos3Θ)/sin θ cos θ]
= [(sin θ-cos θ)(sin2Θ +cos2Θ +sin θ cos θ)]/[(sin θ-cos θ)sin θ cos θ]
= (1 + sin θ cos θ)/sin θ cos θ)
= 1/sin θ cos θ + 1
= 1 + sec θ cosec θ = R.H.S.
(iv) (1 + sec A)/sec A = sin2Θ /(1-cos A)
L.H.S. = (1 + sec A)/sec A
= (1 + 1/cos A)/1/cos A
= (cos A + 1)/cos A/1/cos A
= cos A + 1
R.H.S. = sin2Θ /(1-cos A)
= (1 –cos2Θ)/(1-cos A)
= (1-cos A)(1+cos A)/(1-cos A)
= cos A + 1
L.H.S. = R.H.S.
(v) (cos A–sin A+1)/(cosA+sin A–1) = cosec A + cot A,using the identity cosec2A = 1+cot2A.
L.H.S. = (cos A–sin A+1)/(cosA+sin A–1)
Dividing Numerator and Denominator by sin A,
= (cos A–sin A+1)/sin A/(cosA+sin A–1)/sin A
= (cot A – 1 + cosec A)/(cot A+ 1 – cosec A)
= (cot A – cosec2A + cot2A + cosec A)/(cot A+ 1 – cosec A) (using cosec2A – cot2A = 1)
= [(cot A + cosec A) – (cosec2A – cot2A)]/(cot A+ 1 – cosec A)
= [(cot A + cosec A) – (cosec A + cot A)(cosec A – cot A)]/(1 – cosec A + cot A)
= (cot A + cosec A)(1 – cosec A + cot A)/(1 – cosec A + cot A)
= cot A + cosec A = R.H.S.
(vi)1+sinA1−sinA−−−−−√=secA+tanA
Dividing Numerator and Denominator of L.H.S. by cos A,
= 1cosA+sinAcosA√1cosA−sinAcosA√
= secA+tanA√secA−tanA√
= secA+tanA√secA−tanA√XsecA+tanA√secA+tanA√
=(secA+tanA)2√sec2A−tan2A√
=secA+tanA1
= sec A + tan A = R.H.S.
(vii) (sin θ – 2sin3Θ)/(2cos3Θ -cos θ) = tan θ
L.H.S. = (sin θ – 2sin3Θ)/(2cos3Θ – cos θ)
= [sin θ(1 – 2sin2Θ)]/[cos θ(2cos2Θ – 1)]
= sin θ[1 – 2(1-cos2Θ)]/[cosθ(2cos2Θ-1)]
= [sin θ(2cos2Θ -1)]/[cos θ(2cos2Θ -1)]
= tan θ = R.H.S.
(viii) (sinA+cosecA)2 + (cosA+secA)2 = 7+tan2A +cot2A
L.H.S. = (sinA+cosecA)2 + (cosA+secA)2
= (sin2A + cosec2A + 2 sin A cosec A) + (tcos2A + sec2A + 2 cos A sec A)
= (sin2A + cos2A) + 2 sin A(1/sin A) + 2 cos A(1/cos A) + 1 + tan2A + 1 + cot2A
= 1 + 2 + 2 + 2 + tan2A + cot2A
= 7+tan2A+cot2A = R.H.S.
(ix) (cosec A – sin A)(sec A – cos A) = 1/(tan A+cotA)
L.H.S. = (cosec A – sin A)(sec A – cos A)
= (1/sin A – sin A)(1/cos A – cos A)
= [(1-sin2A)/sin A][(1-cos2A)/cos A]
= (cos2A/sin A)×(sin2A/cos A)
= cos A sin A
R.H.S. = 1/(tan A+cotA)
= 1/(sin A/cos A +cos A/sin A)
= 1/[(sin2A+cos2A)/sin A cos A]
= cos A sin A
L.H.S. = R.H.S.
(x) (1+tan2A/1+cot2A) = (1−tanA1−cotA)2 =tan2A
L.H.S. = (1+tan2A/1+cot2A)
= (1+tan2A/1+1/tan2A)
= 1+tan2A/[(1+tan2A)/tan2A]
= tan2A
Related Links
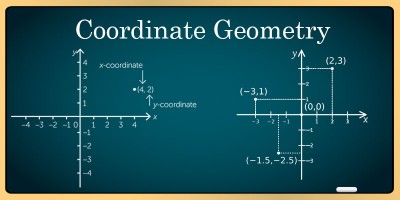
NCERT Solutions for Class 10 Maths – Coordinate Geometry
1. If the point A (x , y) is equidistant from B (4 , 2) and C (-2 , 4). Find the relation between x and y.
|AB| = (x−5)2+(y−1)2−−−−−−−−−−−−−−−√
= x2+16−8x+y2+4−4y−−−−−−−−−−−−−−−−−−−−−√
|AC| = (x+2)2+(y−5)2−−−−−−−−−−−−−−−√
= x2+4+4x+y2+16−8y−−−−−−−−−−−−−−−−−−−−−√
Since, |AB| = |AC|
⇒ x2+y2−8x−4y+20 = x2+y2+4x−8y+20
⇒ 8y – 4y = 4x + 8x
⇒ y = 3x
2. Find the perimeter of a triangle with vertices (0, 8), (0, 0) and (6, 0)
Let the vertices of the △ be P(0, 8), Q(0, 0) and R(6, 0)
∴ PQ = (0)2+(−8)2−−−−−−−−−−√=64−−√=8
∴ QR = (6)2+(0)2−−−−−−−−−√=36−−√=6
∴ RP = (−6)2+(8)2−−−−−−−−−−√=100−−−√=10
∴ Perimeter of △ = 8 + 6 + 10 = 24 units
3.Find the coordinates of the perpendicular bisector of the line segment joining the points P (1, 4) and Q (2, 3), cuts the y-axis.
Here, O (0, y), P (1, 4) and Q (2, 3)
AO = BO
⇒ (0−1)2+(y−4)2−−−−−−−−−−−−−−−√ = (0−2)2+(y−3)2−−−−−−−−−−−−−−−√
⇒ 1+y2+16−8y=4+y2+9−6y
⇒ 8y – 6y = 17 -13
⇒ 2y = 4
y = 2
∴The required point is (0, 2)
4. The points which divides the line segment joining the points (5, 8) and (1, 2) in ratio 1:2 internally lies in which quadrant?
Now C(1+103,2+163)
∴ C(113,6)

Since, C(113,6) lies in IV quadrant.
5. If A (x2,3) is the mid-point of the line segment joining the points B(4,1) and C(8,7). Find the value of x.
∴4+82=a2
⇒ 24 = 2a
⇒ a = 12
6. A line intersects the y-axis at point A and B respectively. If (3, 4) is the mid-point of AB. Find the coordinates of A and B.
Here, x+02=3 ⇒x=6
0+y2=4 ⇒x=8

∴ The coordinates of A and B are (0, 8) and (6, 0).
7. Find the fourth vertex S of a parallelogram PQRS whose three vertices are P (-3, 8), Q (5, 4) and R (6, 2).
Here, −3+62=5+x2⇒x=−1
8+22=4+x2⇒x=3
∴The fourth vertices S of a parallelogram PQRS is S(-1, 3)
8. Find the coordinates of the point which is equidistant from the three vertices of the
△POQ.

Point equidistant from the three vertices of a right angle triangle is the mid-point of hypotenuse.
⇒(4x+02,0+4y2)⇒(2x,2y)
9. Find the area of a triangle with vertices (x, y + z), (y, z + x) and (z, x + y).
Area of the required
△=12|x(z+x−x−y)+y(x+y−y−z)+(y+z−z−x)|
= 12|x(z−y)+y(x−z)+z(y−a)|
= 12|0| = 0.
10. Find the area of a triangle with vertices P (2, 0), Q (6, 0) and R (5, 4)
Area of the required △PQR
= 12|2(0–2)+6(4–0)+5(0–0)|
= 12|24−4|=10 Sq. units.
11. If the points O (0, 0), P (3, 4), Q (x, y) are collinear, then write the relation between x and y.
Since, O (0, 0), P (3, 4), Q (x, y) are collinear.
⇒ Area of a triangle formed by these points vanishes
∴ 12|0(4–y)+3(y−0)+x(0−4)|=0
⇒y−2x=0⇒2x=y
12. Find the coordinates of the point O dividing the line segment joining the point P (6, 5)
and Q(2, 10) in the ratio 2:1.

x=2(2)+1(6)5=4+65=2
and y=2(10)+1(5)5=20+55=5
∴ O(2, 5)
13. The line joining A(4, 3) and B(-3, 6) meets y-axis at C. At what ratio dose C divides the line segment AB?
Since,R lies on y-axis, let C be (0, y)

Suppose that the required ratio be z:1
∴x=−3z+4z+1⇒0=−3z+4⇒z=43
∴ The required ratio is 4 : 3.
Related Links
NCERT Solutions for Class 10 Maths – Triangles
Exercise 1
Question 1:
Fill in the blanks
A: All circles are ________ (Similar, Congruent)
Solution: All circles are similar.
B: All squares are ________ (Congruent, similar)
Solution: All squares are similar.
C: All _________ triangles are similar. (Isosceles, equilateral)
Solution: All equilateral triangles are similar.
D: Two polygons having the same number of sides are similar, if
(i) Their corresponding angles are _______ and
(ii) Their corresponding sides are __________. (Proportional, equal)
Solution: Two polygons having the same number of sides are similar, if (i) their corresponding angles are equal and (ii) their corresponding sides are proportional
Question 2:
Give examples for the following, two of each.
A: Similar figures
B: Non-similar figures
Solution:
A: Two ten dollar notes and two ten cent coins.
B: One 5 cent coin and 10 cent coin and one 5 dollar note and ten dollar note.
Question 3:
Find out whether the quadrilaterals that are given below are similar.

Solution:
Since, the corresponding angles of both the figures are not equal, the figures given are not similar.
Exercise 2
Question 1:
From the given figures , we get that ST ǁ QR. Find TR in (i) and PS in (ii).

Solution:
In the triangle, PQR, ST ǁ QR (given)
Therefore, PS/SQ = PT/TR [Using the basic Proportionality theorem]
=> 1.5/3 = 1/ TR
=> ∑ TR = 3/1.5
TR = 3 x 10/15 = 2cm
Hence, TR = 2cm
(ii) In triangle PQR, ST ǁ QR (given)
Therefore, PS/ SQ = PT / TR [Using the basic proportionality theorem]
= > PS/7.2 = 1.8/5.4
= > PS = 1.8 x 7.2/5.4 = 18/10 x 72/10 x 10/54 = 24/10
= > PS = 2.4
Hence, PS = 2.4 cm
Question 2:
A and B are two points on the sides of XY and XZ respectively of a XYZ triangle. State whether AB ǁ YZ for the following given cases:
1: XA = 3.9 cm, AY = 3 cm XB = 3.6 cm and BZ= 2.4 cm
2: XA = 4 cm, YA = 4.5 cm, XB = 8 cm and ZB = 9 cm
3: XY = 1.28 cm, XZ = 2.56 cm, XA = 0.18 cm and XB = 0.63 cm

Solution:
In triangle XYZ, A and B are the two points on sides XY and XZ respectively.
1: XA = 3.9 cm and AY = 3 cm (given)
XB = 3.6 cm, BZ = 2.4 cm (Given)
Therefore, XA/AY = 3.9/3 = 39/30 = 13/10 = 1.3 [Using the basic proportionality theorem]
And, XB/BZ = 3.6/2.4 = 36/24 = 3/2 = 1.5
So, XA/AY ≠ XB/BZ
Hence, AB is not parallel to YZ.
2: XA = 4cm, YA = 4.5 cm, XB = 8cm, ZB= 9cm
Therefore, XA/YA = 4/4.5 = 40/45 = 8/9 [Using the basic proportionality theorem]
And, XB/ZB = 8/9
So, XA/YA = XB/ZB
Hence, AB is parallel to YZ
3: XZ = 1.28 cm, XZ = 2.56 cm, XA= 0.18 cm, XB= 0.36 cm (Given)
Here, AY = XY – XA = 1.28-0.18 = 1.10 cm
And, BZ = XZ – XB = 2.56 – 0.36 = 2.20 cm
So, XA/AY = 0.18/1.10 = 18/110 = 9/55 – – – – – – – – – – – – (1)
And, XA/BZ = 0.36/2.20 = 36/220 = 9/55 – – – – – – – – – – – (2)
Therefore, XA/AY = XB/BZ
Hence, AB is parallel to XY.
Question 3:
From the given figure, we see that AB ǁ RQ and AC ǁ RS, prove that PB/BQ = PC/PS

Solution:
From the given figure, we get AB ǁ RQ
By using the basic proportionality theorem, we get,
PB/BQ = PA/ PR – – – – – – – – (1)
Similarly, AC ǁ RS
Therefore, PC/PS = PA/PR – – – – (2)
From (1) and (2) we get,
PB/BQ = PC/PS
Question 4:
From the figure we get, ST ǁ PR and SU ǁ PT. Prove that QU/UT = QT/TR

Solution:
In triangle PQR, ST ǁ PR (Given)
Therefore, QS/SP= QT/TR – – – – – – – (1) [Using the proportionality Theorem]
In triangle PQR, SU ǁ PT (Given)
Therefore, QS/SP = QU/UT – – – – – – (2) [Using the basic proportionality theorem]
From equation (1) and (2) we get,
QT/TR = QU/UT
Question 5:
From the following figure we get, XZ ǁ AC and XZ ǁ AD, show that YZ ǁ CD.

Solution:
In triangle BCA, XY ǁ AC (Given)
Therefore, BX/XA = BY/YC – – – – – – – (1) [Using the basic proportionality theorem]
In triangle BCA, XY ǁ AC (Given)
Therefore, BX/XA = BZ/ZD – – – – – – – (2) [Using the basic proportionality theorem]
From the equation (1) and (2) we get,
BY/YC = BZ/ZD
In triangle BCA, YZ ǁ CD [By converse of the basic proportionality theorem]
Question 6:
From the figure, three points X, Y and Z are points on AB, AC and AD respectively such that XY ǁ BC and XZ ǁ BD. Show that YZ ǁ CD

Solution:
In triangle ABC, XY ǁ BC (Given)
Therefore, AX/XB = AY/YC – – – – – – – (1) [Using the basic proportionality theorem]
In triangle ABC, XZ ǁ BD (Given)
Therefore, AX/XB = AZ/ ZD – – – – – – – (2) [Using the basic proportionality theorem]
From the equations (1) and (2), we get
AY/ YC = AZ/ZD
In triangle ACD, YZ ǁ CD [By the converse of basic proportionality theorem]
Question 7:
Using the basic proportionality theorem, prove that a line drawn through the midpoints of one side of a triangle is parallel to the other side that bisects the third side.
Solution:

From the given diagram we get,
The triangle PQR in which S is the midpoint of P and Q such that PS=SQ
A line parallel to QR intersects PR at T such that ST ǁ QR
To prove: T is the midpoint of PR
Proof: S is the midpoint of PQ
Therefore, PS=SQ
=>PS/QS = 1 – – – – – – – (1)
In triangle PQR, ST ǁ QR,
Therefore, PS/SQ = PT / TR [Using the basic proportionality theorem]
=>1 = PT/ TR [from equation (1)]
Therefore, PT = TR
Hence, T is the midpoint of PR
Question 8:
Prove that the line joining the midpoints of any two sides of a triangle is parallel to the third side by using the converse of basic proportionality theorem.
Solution:

Given:
From the triangle PQR in which ST are the midpoints of PQ and PR respectively such that PS = SQ and PT= TR
To prove: ST ǁ QR
Proof: S is the midpoint of PQ (Given)
Therefore, PS = SQ
=>PS/QS = 1 – – – – – – – – – (1)
Also, T is the midpoint of PR (Given)
Therefore, PT= TR
=>PT/TR = 1 [from equation (1)]
From equation (1) and (2) we get,
PS/ QS = PT/ TR
Hence, ST ǁ QR [By the converse of the basic proportionality theorem]
Question 9:
PQRS is a trapezium in which PQ ǁ SR and its diagonals intersect each other at a point A. show that PA/QA = RA/SA
Solution:

Given:
PQRS is a trapezium in which PQ ǁ RS in which the diagonals PR and QS intersect each other at A.
To prove: PA/QA = RA/ SA
Construction: Through A, draw TA ǁ SR ǁ PQ
Proof: In triangle PSR, we have
AT ǁ SR (By construction)
Therefore, PT/TS = PA/RA – – – – – – – (1) [Using the basic proportionality theorem]
In triangle PQS, we have
AT ǁ PQ (by construction)
Therefore, ST/TP = SA/QA – – – – – – – – – (2) [Using the basic proportionality theorem]
From the equations (1) and (2) we get,
PA/RA = QA / SA
=>PA / QA = RA/SA
Question 10:
The diagonals of a quadrilateral PQRS intersect each other at the point A such that PA/QA = RA/SA. Show that PQRS is a trapezium.

Solution:
Given:
Quadrilateral PQRS in which diagonals PR and QS intersect each other at A such that PA/ QA = RA/SA
To prove: PQRS is a trapezium
Construction: Through A, draw line TA, where TA ǁ PQ, which meets PS at T
Proof: In triangle SPQ, we have
TA ǁ PQ
Therefore, ST/TP = SA/AQ – – – – – – (1) [Using the basic proportionality theorem]
Also, PA/QA = RA/SA (Given)
=>PA/ RA = QA/ SA
=> RA/PA = QA/SA
=> SA/AQ = RA/PA – – – – – – – – (2)
From the equations (1) and (2) we get,
ST / TP = RA/ PA
Therefore, By using converse of Basic proportionality theorem,
TA ǁ SR also TA ǁ PQ
=>PQ ǁ SR
Hence, quadrilateral PQRS is a trapezium with PQ ǁ RS
Exercise 3
Question 1:
Which of the following triangle pairs are similar? State the similarity criterion you used to determine the similarity of the triangles.

Solution:
(i)For ΔABC and ΔPQR:
∠A=∠P = 60o (Given)
∠B =∠Q = 80o(Given)
∠C =∠R = 40o(given)
∴ Δ ABC ~ ΔPQR (AAA similarity criteria)
(ii)For ΔJKL and ΔZXC
JK/XC = KL/CZ = JL/XZ
∴ ΔJKL~ ΔZXC (SSS similarity criterion)
(iii) For ΔJKL and ΔZXC:
JK = 2.7, KL = 2, LJ = 3, ZX = 5, XC = 4, CZ = 6
KL/ZX = 2/4 = 1/2
JL/ZC = 3/6 = 1/2
JK/XC= 2.7/5 = 27/50
Here, KL/ZX = LJ/ZC ≠ JK/XC
Thus, ΔJKL and ΔZXC are not similar.
(iv) For ΔJKL and ΔZXC
JK = 2.5, KL = 3, ∠J = 80°, XC = 6, ZC = 5, ∠C = 80°
Here, JK/ZC = 2.5/5 = 1/2
And, KL/XC = 3/6 = 1/2
⇒ ∠K ≠ ∠C
Thus, ΔJKL and ΔZXC are not similar.
(v) For ΔJKL, we have
∠J + ∠K + ∠L = 180° (sum of angles of a triangle)
⇒ 70° + 80° + ∠L = 180°
⇒ ∠L = 180° – 70° – 80°
⇒ ∠L = 30°
In ZXC, we have
∠Z + ∠X + ∠C = 180 (Sum of angles of Δ)
⇒ ∠Z + 80° + 30° = 180°
⇒ ∠Z = 180° – 80° -30°
⇒ ∠Z = 70°
In ΔJKL and ΔZXC, we have
∠J = ∠Z = 70°
∠K = ∠X = 80°
∠L = ∠C = 30°
Thus, ΔJKL ~ ΔZXC (AAA similarity criterion)
Question 2:
In the figure below, ΔJKL ∝ ¼ ΔZXL, ∠KJL=70O and ∠ZLX = 70O. Find ∠JLK, ∠JKL AND ∠LZX

Solution:
JLX is a straight line.
Thus, ∠JLK + ∠ KLX = 180°
⇒ ∠JLK = 180° – 125°
= 55°
In ΔJLK,
∠JKL+ ∠ KJL + ∠ JLK = 180°
(Sum of the measures of the angles of a triangle is 180º.)
⇒ ∠JKL + 70º + 55º = 180°
⇒ ∠JKL = 55°
Given that ΔLJK ~ ΔLXZ.
∴ ∠LZX = ∠LKJ (Corresponding angles are equal in similar triangles)
⇒ ∠ LZX = 55°
∴ ∠LZX= ∠LKJ (Corresponding angles are equal in similar triangles)
⇒ ∠LZX= 55°
Question 3:
Diagonals JX and KZ of a trapezium JKZX with JK || ZX intersect each other at the point L. With the help of similarity criterion for two triangles, prove that JL/LX = KL/KZ.

Solution:
In ΔZLX and ΔJLK,
∠XZL = ∠JKL (Alternate interior angles as JK || XZ)
∠ZXL = ∠KJL (Alternate interior angles as JK || XZ)
∠ZLX = ∠KLJ (Vertically opposite angles)
∴ ΔZLX ~ ΔKLJ (AAA similarity criterion)
∴ ZL/KL = LX/LJ (Corresponding sides are proportional)
⇒ LJ/LX = LK/LZ
Question 4:
In the given figure, LX/LZ = LJ/KX and ∠1 = ∠2. Prove that ΔKXZ ~ ΔJLX.

Solution:
In ΔJLX, ∠KLX = ∠KXL
∴ KL = KX … (i)
Given, LX /LZ = LJ/KX
Using (i), we get
LX/LZ = LJ/LK ..(ii)
In ΔKLZ and ΔJLX,
LX/LZ = LJ/LK (using(ii))
∠L = ∠L
∴ ΔKZX ~ ΔJLX [SAS similarity criterion]
Question 5:
In the given figure, K and L are points on sides JZ and JX of ΔZXJ such that ∠Z = ∠JLK. Prove that ΔJZX ~ ΔJLK.

Solution:
In ΔJZX and ΔJKL,
∠JLK = ∠XZK (Given)
∠J = ∠J (Common angle)
∴ ΔJZX ~ ΔJLK (By AA similarity criterion)
Question 6:
In the figure given, if ΔJZL ≅ ΔJXK, prove that ΔJKL ~ ΔJZX.

Solution:
Given, that ΔJZL ≅ ΔJXK.
∴ JZ = JX [By cpct] … (i)
Also, JK = JL [By cpct] … (ii)
In ΔJKL and ΔJZX,
JK/JZ = JL/JX [Dividing equation (ii) by (i)]
∠J = ∠J [Common angle]
∴ ΔJKL ~ ΔJZX [By SAS similarity criterion]
Question 7:
In the figure given below, lines ZK and JX of ΔJZC intersect each other at the point L. Prove that:
(i) ΔZXL ~ ΔJKL
(ii) ΔZCK ~ ΔJCX
(iii) ΔZXL ~ ΔZKC
(iv) ΔLKJ ~ ΔCXJ

Solution:
(i) In ΔZXL and ΔJKL,
∠ZXL = ∠JKL (Each 90°)
∠ZLX = ∠JLK (Vertically opposite angles)
Thus, by using AA similarity criterion,
ΔZXL ~ ΔJKL
(ii) In ΔZCK and ΔJCX,
∠ZKC = ∠JXC (Each 90°)
∠ZCK = ∠JCX (Common)
Hence, by using AA similarity criterion,
ΔZCK ~ ΔJCX
(iii) In ΔZXL and ΔZKC,
∠ZXL = ∠ZKC (Each 90°)
∠LZX = ∠KZC (Common)
Hence, by using AA similarity criterion,
ΔZXL ~ ΔZKC
(iv) In ΔLKJ and ΔCXJ,
∠LKJ = ∠CXJ (Each 90°)
∠LJK = ∠CJX (Common angle)
Hence, by using AA similarity criterion,
ΔLJK ~ ΔCXJ
Question 8:
In the given figure X is a point on the side JZ of a parallelogram JKCZ and KX intersect ZC at L. Prove that ΔJKX ~ ΔCLK.

Solution:
In ΔJKX and ΔCLX,
∠J = ∠C (Opposite angles of a parallelogram)
∠JXK = ∠CKL (Alternate interior angles as JX || KC)
∴ ΔJKX ~ ΔCLX (By AA similarity criterion)
Question 9:
In the given figure, JKC and AMP are two right triangles, right angled at B and M respectively, show that:
i) ΔJZC ~ ΔJKX
(ii) CJ/XJ = ZC/KX
Solution:

(i) In ΔJZC and ΔJKX, we have
∠J= ∠J (common angle)
∠JZC = ∠JKX = 90° (each 90°)
∴ ΔJZC ~ ΔJKX (By AA similarity criterion)
(ii) Since, ΔABC ~ ΔAMP (By AA similarity criterion)
If two triangles are similar then the corresponding sides are equal,
Hence, CJ/XJ = ZC/KX
Question 10:
In the given figure CD and KH are the bisectors of ∠ZCX and ∠JKL respectively, such that D and H lie on sides ZX and JL of ΔZXC and ΔJKL respectively. If ΔZXC ~ ΔJKL. Prove that:
(i) CD/KH = ZC/JK
(ii) ΔCXD ~ ΔKLH
(iii) ΔDZC ~ ΔHKJ

Solution:
(i) Given that ΔZXC ~ ΔJKL
∴ ∠Z = ∠J, ∠X = ∠L, and ∠ZCX = ∠JKL
∠ZCX = ∠JKL
∴ ∠ZCD = ∠JGH (Angle bisector)
And, ∠DCX = ∠HKL (Angle bisector)
In ΔDZC and ΔJKH,
∠Z = ∠J (Proved above)
∠DCZ = ∠JKH (Proved above)
∴ ΔDZC ~ ΔJKH (By AA similarity criterion)
⇒ CD/KH = ZC/JK
(ii) In ΔXCD and ΔHKL,
∠XCD = ∠HK; (Proved above)
∠X = ∠L (Proved above)
∴ ΔXCD ~ ΔHKL (By AA similarity criterion)
(iii) In ΔZCD and ΔHJK
∠DCZ = ∠JKH (Proved above)
∠Z = ∠J (Proved above)
∴ ΔZCD ~ ΔHJK (By AA similarity criterion)
Question 11:
In the following figure, K is a point on side CL of an isosceles triangle JCL, where JL= JC. If JZ ⊥ LC and KF ⊥ CJ. Show that ΔJLZ ~ ΔFCK.

Solution:
It is given that JLC is an isosceles triangle.
∴ JL = JC
⇒ ∠JLZ = ∠FCJ
In ΔJLZ and ΔFKC,
∠JDL = ∠CFK (Each 90°)
∠LJZ = ∠FKC (Proved above)
∴ ΔJLZ ~ ΔFCK (By using AA similarity criterion)
Question 12:
In the given figure sides ZX and XC and median ZV of a triangle ZXC are proportional to sides HJ and JL and median HK of ΔPQR, respectively. Prove that ΔZCX ~ ΔHJL.

Solution:
It is given that:
ΔZCX and ΔHJL, ZX, CX and median ZV of ΔZCX are proportional to sides HJ, JL and median HK of ΔHJL
Or, ZX/HJ = CX/JL= ZV/
To Prove: ΔZCX ~ ΔHJL
Proof: ZX/HJ = CX/JL = ZV/HK
⇒ ZX/HJ = CX/JL = ZV/HK (V is the mid-point of CX and K is the midpoint of JL)
⇒ ΔZXV ~ ΔHJK [SSS similarity criterion]
∴ ∠ZXV = ∠HJK [Corresponding angles of two similar triangles are equal]
⇒ ∠ZXC = ∠HJL
In ΔABC and ΔPQR
ZX/HJ = CX/JL ….(i)
∠CXZ = ∠HJL….. (ii)
Hence, from equation (i) and (ii), we get
ΔCXZ ~ ΔHJL [By SAS similarity criterion]
Question 13:
J is a point on the side CK of a triangle CJK such that ∠JLC = ∠KJC. Prove that CJ2 = CK.LC

Solution:
In ΔCJL and ΔJKC,
∠CLJ = ∠CJK (Given)
∠JCL = ∠KCJ (Common angle)
∴ ΔCJL ~ ΔJKC (By AA similarity criterion)
We know that corresponding sides of similar triangles are in proportion.
∴ CJ/KC =CL/JC
⇒ CJ2 = CK.LC.
Question 14:
In the given figure, sides ZX and ZC and median ZV of a triangle CXZ are proportional to sides JK and JH and median JL of another triangle JKH, respectively. Show that ΔCXZ~ ΔJKH

Solution:
It is given that:
Two triangles ΔCXZ and ΔJKH in which ZV and JL are medians such that ZX/JK = CZ/JH = ZV/JL
To Prove: ΔZXC ~ ΔJKH
Construction: Produce ZV to F such that ZV = VF. Connect CF. Similarly produce JL to N so that JL = LN, also connect HN.
Proof:
In ΔZXV and ΔVCF, we have
ZV = VF [By Construction]
XV = VC [∴ AP is the median]
And, ∠ZVX = ∠CVF [Vertically opp. angles]
∴ ΔZXV ≅ ΔCVF [By SAS criterion of congruence]
⇒ ZX = CF [CPCT] ….. (i)
Also, in ΔJKL and ΔLNH, we have
JL = LN [By Construction]
KL = LH [∴ PM is the median]
and, ∠JLK = ∠NLH [Vertically opposite angles]
∴ ΔJKL = ΔLHN [By SAS criterion of congruence]
⇒ JK = HN [CPCT] …. (ii)
Now, ZX/JK = ZC/JH = ZV/JL
⇒ CF/HN = ZC/JH = ZV/JL … [From (i) and (ii)]
⇒ CF/HN = ZC/JH = 2ZV/2JL
⇒ CF/HN = ZC/JH = ZF/JN [∴ 2AD = AE and 2PM = PN]
∴ ΔZCF ~ ΔJHN [By SSS similarity criterion]
Therefore, ∠2 = ∠4
Similarly, ∠1 = ∠3
∴ ∠1 + ∠2 = ∠3 + ∠4
⇒ ∠Z = ∠J … (iii)
Now, In ΔZXC and ΔJKH, we have
ZX/JK = ZC/JH (Given)
∠Z = ∠J [From (iii)]
∴ ΔZXC ~ ΔJKH [By SAS similarity criterion]
Question 15:
A vertical pole of a length 6 m casts a shadow 4m long on the ground and at the same time, a tower casts a shadow 28 m long. Find the height of the tower.

Solution:
Length of the vertical pole = 6m (Given)
Shadow of the pole = 4 m (Given)
Let Height of tower = h m
Length of shadow of the tower = 28 m (Given)
In ΔZXC and ΔJKL,
∠C = ∠K (angular elevation of sum)
∠X = ∠L = 90°
∴ ΔZXC ~ ΔJKL (By AA similarity criterion)
∴ ZX/JL = XC/KL (when two triangles are similar corresponding sides are proportional)
∴ 6/h = 4/28
⇒ h = 6×28/4
⇒ h = 6 × 7
⇒ h = 42 m
Therefore, the height of the tower is 42 m.
Question 16:
In the given figure if ZV and JM are medians of triangles ZXC and JKL, respectively. Where ΔZXC ~ ΔJKL prove that ZX/JK = ZV/JM.

Solution:
It is given that: ΔZXC ~ ΔJKL
We know that, the corresponding sides of similar triangles are in proportion
.∴ ZX/JK = ZC/JL = KC/KL … (i)
Also, ∠ Z= ∠J, ∠X = ∠K, ∠C = ∠L …(ii)
As ZV and JM are medians, their opposite sides will be divided by them
.∴ XV = XC/2 and KM = KL/2 …(iii)
From equations (i) and (iii), we have
ZX/JK = XV/KM …(iv)
In ΔZXV and ΔJKM,
∠X = ∠K [Using equation (ii)]
ZX/JK = XV/KM [Using equation (iv)]
∴ ΔZXV ~ ΔJKM (By SAS similarity criterion)
⇒ ZX/JK = XV/KM = ZV/JL.
Exercise 4
Question 1:
Let ∆ PQR ~ ∆ STU and their areas be, 64cm2 and 121cm2 respectively. If TU = 15.4, then find QR.
Solution:
Given,
The area of a triangle PQR = 64 cm2
The area of a triangle STU = 121 cm2
TU = 15.4 cm
And ∆ PQR ~ ∆ STU
Therefore, area of triangle PQR/ Area of triangle STU = PQ2 / ST2
= PR2 / SU2 = QR2 / TU2 – – – – – – – – – – (1)
[If the two triangles are similar then the ratio of their areas are said to be equal to the square of the ratio of their corresponding sides]
Therefore, 64 / 121 = QR2 / TU2
=> (8/11)2 = (QR/15.4)2
=> 8/11 = QR / 15.4
=> QR = 8 x 15.4 / 11
=> QR = 8 x 1.4
QR = 11.2 cm
Question 2:
Diagonals of a trapezium PQRS with PR ǁ SR intersect each other at the point A. If PQ = 2RS, then find the ratio of the areas of the triangles PAQ and RAS.

Solution:
PQRS is a trapezium having PQ ǁ SR. The diagonals PR and QS intersect each other at a point A.
In ∆ PAQ and ∆ RAS, we have
∠1=∠2(Alternateangles) ∠3=∠4(Alternateangles) ∠5=∠6(Verticallyoppositeangle)
Therefore, ∆ PAQ ~ ∆ RAS [By AAA similarity criterion]
Now, Area of (∆PAQ) / Area of (∆RAS)
= PQ2 / RS2 [If two triangles are similar then the ratio of their areas are equal to the square of the ratio of their corresponding sides]
= (2RS)2 / RS2 [Therefore, PQ=RS]
Therefore, Area of (∆ PAQ)/ Area of (∆RAS)
= 4RS2/ RS = 4/1
Hence, the required ratio of the area of ∆PAQ and ∆RAS = 4:1
Question 3:
From the given figure, PQR and SQR are two triangles on the same base QR. If PS intersects QR at A, show that the area of triangle PQR / Area of triangle SQR = PA. SA

Solution:

Given,
PQR and SQR are the triangles in which have the same base QR. PS intersects QR at A.
To prove: Area of triangle PQR/ Area of triangle SQR = PA/ SA
Construction: Let us draw two perpendiculars PY and SX on line QR.
Proof:
We know that the area of a triangle = ½ x base x Height
Therefore, areaΔPQRareaΔSQR = 12QR×PY12QR×SX
In ∆ PYA and ∆ SXA,
∠PYA=∠SXA(Eachequalsto90∘) ∠PAY=∠SAX(Verticallyoppositeangles)
∴ΔPYA∼ΔSXA(ByAAsimilaritycriterion∴PYSX) = PASA
=> area of triangle PQR/ area of triangle SQR
=> PA/SA
Question 4:
If the areas of two triangles are similar and equal then, prove that they are congruent.

Solution:
Given:
∆ MNO and ∆ XYZ are similar and equal in area.
To prove that: ∆ MNO ≅ ∆ XYZ
Proof: Since, ∆ MNO ~ ∆ XYZ
Therefore, Area of (∆ MNO) / Area of (∆ XYZ) = NO2/ YZ2
=> NO2 / YZ2 = 1 [Since the area of triangle MNO = area of triangle XYZ]
=> NO2 / YZ2
=> NO / YZ
Similarly, we can prove that
MN = XY and MO = XZ
Thus, ∆ MNO ≅ ∆XYZ [ By SSS criterion of congruence]
Question 5:
X , Y and Z are respectively the mid-points of sides PQ, QR and RP of ΔPQR. Find the ratio of the areas of ΔXYZ and ΔPQR.

Solution:
Given:
X, Y and Z are the mid-points of sides PQ, QR and RA respectively from the ΔPQR.
To Find: area(ΔXYZ) and area(ΔPQR)
Solution: In Δ PQR, we have
Z is the midpoint of PQ (Given)
Y is the midpoint of PR (Given)
So, by the mid-point theorem, we have
ZY || RQ and ZY = ½ RQ
⇒ ZY || RQ and ZY || QX [QX = ½ QR]
∴ QXYZ is parallelogram [Opposite sides of parallelogram are equal and parallel]
Similarly, in Δ ZQX and Δ XYZ, we have
ZQ = XY (Opposite sides of parallelogram QXYZ)
ZX = ZX (Common)
QX = ZY (Opposite sides of parallelogram QXYZ)
∴ Δ ZQX ≅ ΔXYZ
Similarly, we can prove that
Δ PZY ≅ Δ XYZ
Δ YXR ≅ Δ XYZ
If triangles are congruent, then they are equal in area.
So, area(Δ FBD) = area(Δ DEF) …(i)
area(Δ AFE) = area(Δ DEF) …(ii)
and, area(Δ EDC) = area(Δ DEF) …(iii)
Now, area(Δ ABC) = area(Δ FBD) + area(Δ DEF) + area(Δ AFE) + area(Δ EDC) …(iv)
area(Δ ABC) = area(Δ DEF) + area(Δ DEF) + area(Δ DEF) + area(Δ DEF)
⇒ area(Δ DEF) = 1/4area(Δ ABC) [From (i), (ii) and (iii)]
⇒ area(Δ DEF)/area(Δ ABC) = 1/4
Hence, area(Δ DEF):area(Δ ABC) = 1:4
Question 6:
Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.

Solution:
Given:
PA and XB are the medians of triangles PQR and XYZ respectively and Δ PQR ~ Δ XYZ
To prove: Area (Δ PQR)/ Area (Δ XYZ) = PA2 / XB2
Proof: Δ PQR ~ Δ XYZ (Given)
Therefore, Area (Δ PQR) / area (Δ XYZ ) = ( PQ2 / XY2) – – – – – – (1)
And, PQ / XY = QR / YZ = RP / ZX – – – – – (2)
PQXY=12QR12YZ=RXZX
In Δ PQA and Δ XYB, we have
Therefore, ∠Q=∠Y(SinceΔPQR∼ΔXYZ)
PQ / XY = QA / YB [Prove in (1)]
Therefore, Δ PQR ~ Δ XYZ [By SAS similarity criterion]
=> PQ/XY = PA/ XB – – – – – – – (3)
Therefore, Δ PQA ~ Δ XYB
The areas of two similar triangles are proportional to the squares of the corresponding sides.
Therefore, Area of triangle PQR / Area of triangle XYZ = PQ2 / XY2 = PA2/ XB2
Question 7:
Prove that the area of an equilateral triangle described on one side of a square is equal to half the area of the equilateral triangle described on one of its diagonals.

Solution:
Given;
PQRS is a square whose one diagonal is PR. Δ PXR and Δ QYR are two equilateral triangles described on the diagonals PR and side QR of the square PQRS.
To prove:
Area of Δ QYR = ½ Area of Δ PXR
Proof:
Δ PXR and Δ QYR are both equilateral triangles (Given)
Therefore, Δ PXQ ~ Δ QYR [AAA similarity criterion]
Therefore, Area of Δ PXR / Area of Δ QYR = PR2 / QR2
(2√QRQR)2=2QR2QR2=2[Since,Diagonal=2–√side=2–√QR]
=> Area (Δ PXR) = 2 x area (Δ QYR)
=> area (Δ QYR) = ½ area (ΔPXR)
Question 8:
Tick the correct solutions and explain.
PQR and QST are two equilateral triangles such that S is the midpoint of QR. The ratio of the areas of triangles PQR and QST is:
(i) 2 : 1
(ii) 1 : 2
(iii) 4 : 1
(iv) 1 : 4

Solution:
Δ PQR and Δ QST are two equilateral triangle. S is the midpoint of QR.
Therefore, QS = SR = ½ QR
Let each side of triangle be 2a
As, Δ PQR ~ Δ QST
Therefore, area (Δ PQR ) / area (Δ QST) = PQ2 / QS2 = (2a)2 / (a)2 = 4a2 / a2 = 4/1 = 4:1
Hence, The correct option is (iii)
Question 9:
Sides of two similar triangles are in the ratio 4 : 9. Areas of these triangles are in the ratio
(i) 2 : 3
(ii) 4: 9
(iii) 81 : 16
(iv) 16 : 81

Solution:
PQR and XYZ are two similarity triangles Δ PQR ~ Δ XYZ (Given)
And, PQ / XY = PR / XZ = QR / YZ = 4/9 (Given)
Therefore, Area of ΔPQR / Area of Δ XYZ = PQ2 / XY 2 [the ratio of the areas of these triangles will be equal to the squares of the ratio of the corresponding sides]
Therefore, Area of Δ PQR / Area of Δ XYZ = (4/9)2 = 16/81
=> 16:81
Hence, the correct option is (iv)
Exercise 5
Question 1:
Sides of the triangles are as follows:
- i) 7 cm, 25 cm, 24 cm
- ii) 3 cm, 6 cm, 8 cm
iii) 50 cm, 100 cm, 80 cm
- iv) 5 cm, 12 cm, 13 cm
Determine which of them are right-angled triangles. Write the length of its hypotenuse.
Solution:
- i) Sides of the triangle given are 7 cm, 25 cm and 24 cm.
Squaring the length of these sides we get 49 cm, 625 cm and 576 cm.
However, 49 + 576 = 625
(7)2 + (24)2 = (25)2
It satisfies the Pythagoras theorem. Hence, it is a right-angled triangle.
Length of Hypotenuse = 25 cm.
- ii) Sides of the triangle given are 3 cm, 6 cm and 8 cm.
Squaring the length of these sides we get 9 cm, 36 cm and 64 cm.
However, 9 + 36 ≠ 64
(7)2 + (24)2 ≠ (25)2
It does not satisfy the Pythagoras theorem. Hence, it is not a right-angled triangle.
iii) Sides of the triangle given are 50 cm, 100 cm and 80 cm.
Squaring the length of these sides we get 2500 cm, 10000 cm and 6400 cm.
However, 2500 + 6400 ≠ 10000
(50)2 + (80)2 ≠ (100)2
It does not the Pythagoras theorem. Hence, it is not a right-angled triangle.
- iv) Sides of the triangle given are 5 cm, 12 cm and 13 cm.
Squaring the length of these sides we get 25 cm, 144 cm and 169 cm.
However, 25 + 144 = 169
(5)2 + (12)2 = (13)2
It satisfies the Pythagoras theorem. Hence, it is a right-angled triangle.
Length of Hypotenuse = 13 cm.
Question 2:
ABC is a right-angled triangle at point A. P is a point on BC such that AP ⊥ BC. Prove that AP2 = BP x PC.

Solution:
Given: ΔABC is a right-angled triangle at point A. P is appointed on BC such that AP ⊥ BC.
To prove: AP2 = BP x PC
Proof:
In ΔABC, we have
AB2 = AP2 + BP2 [By Pythagoras theorem]
Or, AP2 = AB2 – BP2 ……….. (i)
In ΔAPC, we have
AC2 = AP2 + PC2 [By Pythagoras theorem]
Or, AP2 = AC2 – PC2 ……….. (ii)
Adding (i) and (ii), we get
2AP2 = (AB2 – BP2) – (AC2 – PC2)
= BC2 – BP2 – PC2 [ BC2 = AB2 + AC2]
= (BP + PC)2 – BP2 – PC2
= 2BP x PC
∴ AP2 = BP x PC
Question 3:
In the given figure, PQM is a right-angled triangle at P. Also PR ⊥ QM.
Show that
i) PQ2 = QR x QM
ii) PR2 = QR x MR

Solution:
i) In ΔPMQ and ΔRPQ, we have
∠MPQ = ∠PRQ = 90o
∠PQM = ∠RQP (Common angle)
∴ΔPMQ ~ ΔRPQ [AA similarity criterion]
⇒ PQ/RQ = QM/PQ
⇒ PQ2 = QR x QM
ii) Let ∠RPQ = x
In ΔRQP,
∠RQP = 180o – 90o – x
∠RQP = 90o – x
Similarly, in ΔRPM
∠RPM = 90o – ∠RQP
= 90o – x
∠RMP = 180o – 90o – (90o – x)
∠RMP = x
In ΔRQP and ΔRPM, we have
∠RQP = ∠RPM
∠RPQ = ∠RMP
∠PRQ = ∠MRP = 90o
∴ ΔRQP ~ ΔRPM [By AAA similarity criterion]
⇒PR/MR = QR/PR
⇒PR2 = QR x MR
Question 4:
PQR is an isosceles right-angled triangle at point R. Prove that PQ2 = 2PR2.
Solution:

Given: ΔPQR is an isosceles triangle right angled at R.
In ΔPRQ, ∠R = 90o
PR = QR (Given)
PQ2 = PR2 +QR2 [By Pythagoras theorem]
= PR2 + PR2 [Since, PR = QR]
PQ2 = 2PR2
Question 5:
PQR is an isosceles triangle with PR = QR. Given that PQ2 = 2PR2. Prove that PQR is a right-angled triangle.
Solution:

Given that ΔPQR is an isosceles triangle having PR = QR and PQ2 = 2PR2
In ΔPRQ,
PR = QR (Given)
PQ2 = 2PR2 (Given)
= PR2 + PR2
= PR2 + QR2 [Since, PR = QR]
Hence, by Pythagoras theorem ΔPQR is a right-angle triangle.
Question 6:
PQR is an equilateral triangle of side 2a. Find each of its altitudes.
Solution:

Given: PQR is an equilateral triangle of side 2a.
Draw PS ⊥ QR
In ΔPSQ and ΔPSR, we have
PQ = PR [Given]
PS = PS [Given]
∠PSQ = ∠PSR = 90o
Therefore, ΔPSQ ≅ ΔPSR by RHS congruence.
In right-angled ΔPSQ,
(PQ)2 = (PS)2 + (QD)2
(2a)2 = (PS)2 + a2
⇒ (PS)2 = 4a2 – a2
⇒ (PS)2 = 3a2
⇒ PS = 3–√a
Question 7:
Sum of the squares of the sides of a rhombus is equal to the sum of the squares of its diagonals. Prove it.
Solution:

Given: PQRS is a rhombus whose diagonals are PR and QS. They intersect at O.
To prove: PQ2 + QR2 + RS2+ PS2 = PR2 + QS2
Since the diagonals of as rhombus bisect each other ar tight angles.
Therefore, PO = RO and QO = SO
In ΔPOQ,
∠POQ = 90o
PQ2 = PO2 + QO2 ……. (i) [By Pythagoras]
Similarly,
PS2 = PO2 + SO2 ……. (ii)
RS2 = SO2 + RO2 ……. (iii)
QR2 = RO2 + QO2 ……. (iv)
Adding equation (i) + (ii) + (iii) + (iv) we get,
PQ2 + PS2 + RS2 + QR2 = 2(PO2 + QO2+ RO2 + SO2)
= 4PO2 + 4QO2 [Since, PO = RO and QO = SO]
= (2PO)2 + (2QO2) = PR2 + QS2
Question 8:
In the given figure, O is a point in the interior of a triangle PQR.

OS ⊥ QR, OT ⊥ PR and OU ⊥ PQ. Show that
i) OP2 + OQ2 + OR2 – OS2 – OT2 – OU2 = PU2 + QS2 + RT2
ii) PU2 + QS2 + RT2 = PT2 + RS2 + QU2
Solution:
Join OP, OQ and OR
i) Applying Pythagoras theorem in ΔPOU, we have
OP2 = OU2 + PU2
Similarly, in ΔQOS
OQ2 = OS2 + QS2
Similarly, in ΔROT
OR2 = OT2 + TR2
Adding these equations,
OP2 + OQ2 + OR2 = OU2 + PU2 + OS2 + QS2 + OT2 + RT2
OP2 + OQ2 + OR2 – OS2 – OT2 – OU2 = PU2 + QS2 + RT2.
ii) PU2 + QS2 + RT2 = (OP2 – OT2) + (OR2 – OS2) + (OQ2 – OU2)
∴ PU2 + QS2 + RT2 = PT2 + RS2 + QU2.
Question 9:
A ladder of 10 m length reaches a window of 8m above the ground. Find the distance of the foot of the ladder from the base of the wall.
Solution:

Let QP be the wall and PR be the ladder,
Therefore, by Pythagoras theorem, we have
PR2 = PQ2 + QR2
102 = 82 + QR2
QR2 = 100 -64
QR2 = 36
QR = 6 m
Therefore, the distance of the foot of the ladder from the base of the wall is 6 m.
Question 10:
An airplane leaves an airport and flies due north at a speed of 1,000 km/hr. At the same time, another airplane leaves the same airport and flies due west at a speed of 1,200 km/hr. How far apart will be the two planes after one and half hours?
Solution:

Speed of the first aeroplane = 1000 km/he
Distance covered by first aeroplane due north in one and half hours
(OA) = 1000 x 3/2 km = 1500 km
Sped of the second aeroplane = 1200 km/hr
Distance covered by second aeroplane due west in one and half hours
(OB) = 1200 x 3/2 km = 1800 km
In right angle ΔPOQ, we have
PQ2 = PO2 + OQ2
⇒ PQ2 = (1500)2 + (1800)2
⇒ PQ = 2250000+3240000−−−−−−−−−−−−−−−√
= 5490000−−−−−−−√
= 30061−−√ km
Hence, the distance between two aeroplanes will be 30061−−√ km.
Question 11:
Two planes of heights 6 m and 11m respectively stand on a plane ground. If the distance between the feet of the poles is 12 m, find the distance between their tops.
Solution:

Let PQ and RS be the poles of height 6 m and 11 m respectively.
Therefore RO = 11 – 6 = 5 m
From the figure, it can be observed that PO = 12 m
Applying Pythagoras theorem for ΔPOR, we get
PO2 = OR2 + PR2
(12m)2 + (5m)2 = (AC)2
AC2 = (144+25) m = 169 m
AC = 13 m
Therefore, the distance between their tops is 13 m.
Question 12:
S and T are points on the sides RP and RQ respectively of a triangle PQR right angled at R. Prove that PT2 + QS2 = PQ2 + ST2
Solution:

Applying Pythagoras theorem in ΔPRT, we get
PR2 + RT2 = PT2 ………. (i)
Applying Pythagoras theorem in ΔQRS, we get
QR2 + RS2 = QS2 ………. (ii)
Adding (i) + (ii), we get
PR2 + RT2 + QR2 + RS2 = PT2 + QS2 ………. (iii)
Applying Pythagoras theorem in ΔRST, we get
ST2 = RS2 + RT2
Applying Pythagoras theorem in ΔPQR, we get
PQ2 = PR2 + RQ2
Putting these values in equation (iii), we get
ST2 + PQ2 = PT2 + QS2.
Question 13:
The perpendicular from P on side QR of a ΔPQR intersects QR at S such that SQ = 3RS. Prove that 2PQ2 = 2PR2 + QR2.

Solution:
Given that in ΔPQR, we have
PS ⊥ QR and SQ = 3RS
In right-angled triangles PSQ and PSR, we have
PQ2 = PS2 + QS2 ……. (i)
PR2 = PS2 + SR2 ……. (ii) [By Pythagoras theorem]
(ii) – (i), we get
PQ2 – PR2 = QS2 – SR2
= 9SR2 – SR2 [Since, SQ = 3SR]
= 8(QR/4)2 [Since, QR = SQ + RS = 3RS +RS = 4RS]
Therefore, PQ2 – PR2 = QR2/2
⇒ 2(PQ2 – PR2) = QR2
Therefore, 2PQ2 = 2PR2 + QR2.
Question 14:
In an equilateral triangle PQR, S is a point on side QR such that QS = 1/3 QR. Prove that 9PS2 = 7PQ2.
Solution:

Let the side of the equilateral triangle be a, and PT be the altitude of ΔPQR.
∴ QT = TR = QR/2 = a/2
And, PT = 3√a2
Given that, QS = 1/3 QR
∴ QS = a/3
ST = QT – QS = a/2 – a/3 = a/6
Applying Pythagoras theorem in ΔPST, we get
PS2 = PT2 + ST2
PS2 = (a3√2)2 + (a6)2
= 3a24 + (a236)
= 28a236
= 79 AB2
⇒ 9AD2 = 7AB2
Question 15:
In an equilateral triangle, prove that three times the square of one side is equal to four times the square of its altitudes.
Solution:

Let the side of the equilateral triangle be a, and PO be the altitude of ΔPQR.
∴ QO = OR = QR/2 = a/2
Applying Pythagoras theorem in ΔPQO, we get
PQ2 = PO2 + QO2
a2=AE2+(a2)2 AE2=a2–a24 AE2=3a24
4AE2 = 3a2
⇒ 4 x (Square of altitude) = 3 x (Square of one side)
Question 16:
Choose the correct solution and justify:
In ΔPQR, PQ = 6√3 cm, PR = 12 cm and QR = 6 cm.
The angle Q is:
i) 120o
ii) 60o
iii) 90o
iv) 45o
Solution:

Given that, PQ = 6√3 cm, PR = 12 cm and QR = 6 cm
We can observe that
PQ2 = 108
PR2 = 144
And, BC2 = 36
PQ2 + BC2 = PR2
The given triangle, ΔPQR is satisfying Pythagoras theorem.
Therefore, the triangle is a right-angled triangle at B.
∴ ∠B = 90o
Hence, the correct option is (iii)
Related Links
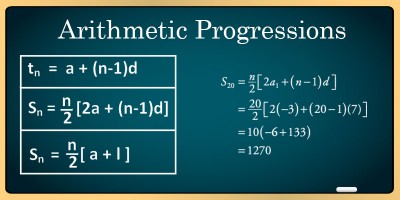
NCERT Solutions for Class 10 Maths – Arithmetic Progressions and Geometric Progression
General Form of an A.P:
Let us consider a series with n number of terms:
a1 + a2 + a3 + a4 + a5 + a6 + a7 …………………………….. = an
The above series of numbers is said to form an Arithmetic Progression (A.P) if,
a2 – a1 = a3 – a2 = a4 – a3 = …………………………. an – an-1 = d
Here, d = common difference of the A.P and it can be positive negative or zero.
Thus the general form of an A.P can be written as:
a, a+ d, a+ 2d, a+ 3d, a+ 4d, a+ 5d …………………………. a + (n – 1)d
Where a = First Term
And, d = Common Difference
Exercise 5.1
Q-1: From the given A.P write the first term (a) and common difference (d).
(i) 2, 4, 6, 8, 10…..
Sol.
Here, a = 2
Since d = a2 – a1
Therefore, d = 4 – 2 = 2
Q-2: Check whether the following list of numbers form an A.P? If they form an A.P then write next three terms.
(i) 5, 25, 45, 65………
Sol.
Here, a = 5
Since for an A.P: a2 – a1 = a3 – a2 = a4 – a3
Therefore, 25 – 5 = 45 – 25 = 65 – 45 = 20
Hence the given number forms an A.P with d = 20
Therefore next three terms of this A.P are: 65 + 20 = 85
85 + 20 =105
105 + 20 = 125
(ii) 3–√,6–√,9–√,12−−√,…………..
Sol.
Here, a = 3–√
Since for an A.P: a2 – a1 = a3 – a2 = a4 – a3
Therefore, 6–√−3–√=9–√−6–√=12−−√−9–√=3–√
Hence the given number forms an A.P with d = 3–√
Therefore, next three terms of this A.P are: 12−−√+3–√=15−−√15−−√+3–√=18−−√18−−√+3–√=21−−√
(iii) −12,−14,−18……
Sol.
Here, a = −12
Since for an A.P: a2 – a1 = a3 – a2 = a4 – a3
Therefore, −14−12≠−18−14
Hence the given numbers does not form an A.P
The nth term of an A.P is given by:
an = a + (n – 1)×d
Here, a = first term of an A.P and d = common difference
Example-1: Let us consider an A.P 2, 7, 12, 17……. Find its 9th term.
Sol.
Here, a = 2
d = 7 – 2 = 17 – 12 = 5
n = 9
Therefore, a9 = 2+ (9 – 1)5
a9 = 42
Therefore, 9th term of this A.P = 4
Example-2 Fourth term of an A.P is 12 and Sixth term of an A.P is 18. Determine the A.P
Sol.
Given, a4 = 12 and a6 = 18
Let a be the first term and d be the common difference of an A.P
Then 12 = a + (4 – 1)d
Therefore, 12 = a + 3d . . . . (1)
And 18 = a + (6 – 1)d
Therefore 18 = a + 5d . . . . . . . (2)
Now, equation (2) – equation (1)
18 – 12 = 5d – 3d Therefore d = 3
Putting d = 3 in equation (2) we get
18 = a + 5×(3)
Therefore a = 3
Hence, the required A.P is 3, 6, 9, 12, 15, 18, 21………
Example -3 Determine which term of the following A.P : 14, 9, 4 . . . . . . is -96 ?
Sol.
From the given A.P,
a = 14,
d = -5,
an = -96,
n =??
Since, an = a + (n – 1)d
Therefore -96 = 14 + (n – 1)×-5
(or) 5n = -96 -14 -5
Therefore n = -23 (neglecting –ve sign)
Therefore, 23rd term of this A.P is -96.
Example-4 Check whether 328 is the term in the following A.P: 76, 97, 118, 139………
Sol.
From the given A.P:
a = 76,
d = 97 – 76 = 21,
an = 328
Since, an = a + (n – 1)d
Therefore 328 = 76 + (n – 1)×21
(or) 21n = 328 + 21 – 76
(or) n = 13
Since n is a +ve integer
Therefore 328 is the 13th term of this given A.P
Example -5 Find how many two digit numbers are divisible by 5.?
Sol.
Two digit numbers that are divisible by 5 are: 10, 15, 20, 25…………………95
Now, from the given A.P
a = 10
d = 5
an = 95
n = ??
Since, an = a + (n – 1)d
Therefore 95 = 10 + (n – 1)×5
(or) 5n = 95 – 10 + 5
n = 18
Therefore there are 18 two digit numbers that are divisible by 5.
Example-6 From the given A.P: 15, 8, 1, -6………….. -111. Find 8th term from last term.
Sol.
On reversing the given A.P:
a = -111 (in this case, last term will now become its first term)
d = 7 (If we go from first term to last term then d = -7 (8 – 15), so if we reverse this A.P d will become +7)
n = 8
a8 = ??
Since, an = a + (n – 1)d
Therefore, a8 = -111 + (8 – 1)×7 = -111 + 49
a8 = -62
Therefore, 8th term from last term = -62
Exercise – 5.2
Q.1 In the given A.P: 26, 40, 54, 68 ………………. which term is 208?
Sol.
From the given A.P:
a = 26,
d = 40 – 26 = 14,
an = 208,
n = ??
Since, an = a + (n – 1)d
Therefore, 208 = 26 + (n – 1)×14
14n = 208 + 14 – 26
Hence, n = 14
14th term of this given A.P is 208
Q2. Find how many total numbers of terms are there in each of the following A.P
(i) 17, 26, 35, 44,……………………….179
Sol.
From the given A.P :
a = 17,
d = (26 – 17) = 9,
an = 179
n = ??
since, an = a + (n – 1)d
Therefore, 179 = 17 + (n – 1)×9
9n = 179 – 17 + 9
Therefore n = 19
Hence, there are 19 terms in this given A.P
(ii)13,223,433,…………………….3373
From the given A.P :
a = 13,
d = 223–13=7,
an = 3373,
n = ??
Since, an = a + (n – 1)d
Therefore, 3373=13+(n−1)7
21n=337+21-1
Therefore n= 17
Hence, there are 17 terms in this given A.P
Q3. Seventh term of an A.P is -1 and fourth term is 41. Determine the A.P and hence find its 17th term.
Sol.
Given, a7 = -1 and a4 = 41
Let a be the first term and d be the common difference of an A.P
-1 = a + (7 – 1)d
-1 = a + 6d . . . . . . . . . (1)
And 41 = a + (4 – 1)d
41 = a + 3d . . . . . . . (2)
Now, equation (1) – equation (2)
-1 – 41 = 6d – 3d Therefore, d = -14
Putting d = -14 in equation (2) we get
41 = a + 3×(-14)
Therefore, a = 83
Therefore the required A.P is 83, 69, 55, 12, 41, 27, 13………
Now, from the given A.P:
a = 83,
d = 69 – 83 = -14
n = 17
a17 = ??
Since, an = a + (n – 1)d
Therefore a17 = 83 + (17 – 1)× -14
a17 = 208 + 14 – 26
a17 = -141
Therefore 17th term of this given A.P is -141
Q.4 There are 26 terms in an A.P of which fifth term is 61 and the last term is 292. Find 12th term
Sol.
Given,
a5 = a + (5-1)d = 61
61 = a + 4d…………………………………(1)
a26 = a + (26 – 1)d = 292
292 = a + 25d……………………………(2)
Equation (2) – Equation (1)
292 – 61 = 25d – 4d
Therefore, d = 11
Putting the value of d in equation (1) we get
61 = a + 4 × 11
Therefore, a = 17
Hence a12 = 17 + (12 – 1)×11 = 138
Therefore 12th term of this given A.P is 138
Q.5 Find which term of an A.P is zero, if fourth term and tenth of an A.P are -26 and 52 respectively.
Sol.
Given,
an = 0
a4 = a + (4 – 1)d = -26
-26 = a + 3d………………………………(1)
a10 = a + (10 – 1)d = 52
52 = a + 9d……………………………(2)
Equation (2) – Equation (1) we get:
52 + 26 = 9d – 3d
Therefore, d = 13
On putting the values of d in equation (1) we get:
-26 = a + 3×13
Therefore, a = -65
Now, let nth term be 0
Therefore, 0 = a + (n – 1)d
0 = -65 + (n – 1)×13
0 = -65 + 13n -13
n = 6
Therefore 6th term of the given A.P is zero.
Q.6 Find the common difference d, if 14th term of an A.P exceeds its 7th term by 49.
Sol.
Since, an = a + (n – 1)d
Therefore, a14 = a + (14 – 1)d
Similarly, a7 = a + (7 – 1)d
Now, according to the given condition:
a14 – a7 = 49
49 = {a + (14 – 1)d} – {a + (7 – 1)d}
49 = 13d – 6d
Therefore, d = 7 (Common Difference)
Q.7 Consider a series : 3, 20, 37, 54,71 …………………………..,Which term of this given A.P will be 289 more than its 32nd term.
Sol.
Given, a = 3
d = 20 – 3 = 17
Therefore, a32 = a + (32 – 1)×d
a32 = 3 + 31×17
a32 = 530
Now, according to the given condition:
an – a32 = 289 (Since, an > a32)
(3+ (n – 1)17) – 530 = 289
819 = 3 + 17n – 17
n = 49
Thus 49th term of this given A.P will be 289 more than its 32nd term.
Q.8 Find the first three terms of an A.P, if sum of 12th and 15th term is 219 and sum of 5th and 7th term is 114.
Sol.
Since, an = a + (n-1)d
Therefore, according to the given conditions:
a12+ a15 = 219
[a + (12-1)d] + [a + (15-1)d] = 219
2a + 25d = 219 ……………………………………………. (1)
And, a5 + a7 = 114
[a +(5-1)d] + [a+(7-1)d] = 114
2a + 10d = 114 ……………………………………………………(2)
Subtracting equation (2) from equation (1) we get
25d – 10d = 105
Therefore, d = 7
Putting values of d in equation (2) we get
2a + 10×7 = 114
Therefore a = 22
Hence 1st term of this A.P = a = 22
2nd term of this A.P = 22+(2-1)×7 = 29
3rd term of this A.P = 22+(3-1)×7 = 36
Q.9 Nikhil started his work in 2002 at an annual salary of Rs 9000 and each year he received an increment of Rs 300. Find in which year his income will reach Rs 12900?
Sol.
Since, each year Nikhil’s salary is increased by Rs 300 and his starting salary was Rs 9000.
Therefore, this forms an A.P with a = 9000 and d = 300
Given, an = 12900 and n = ??
Since, an = a +(n-1)d
Therefore, 12900 = 9000 + (n-1)×300
300n = 12900 + 300 – 9000
Therefore n = 14
Hence, the salary of Nikhil will be 12900 Rs after 14 years from 2002.
Q.10 Find the first three terms of an A.P, if sum of 11th and 14th term is 126 and sum of 4th and 6th term is 66.
Sol.
Since, an = a + (n-1)d
Therefore, according to the given conditions:
a11+ a14 = 126
[a + (11-1)d] + [a + (14-1)d] = 126
2a + 23d = 126 ……………………………………………. (1)
And, a4 + a6 = 66
[a +(4-1)d] + [a+(6-1)d] = 66
2a + 8d = 66 ……………………………………………………(2)
Subtracting equation (2) from equation (1) we get
23d – 8d = 60
Therefore, d = 4
Putting values of d in equation (2) we get
2a + 8×4 = 66
Therefore a = 17
Hence 1st term of this A.P = a = 17
2nd term of this A.P = 17+(2-1)×4 = 21
3rd term of this A.P = 17+(3-1)×4 = 25
Q.11 In the first week of a particular year Deepak saved Rs 10 and then he increased his weekly savings by 2.25Rs. If Deepak was able to increase his weekly savings to Rs 46 in nth week. Find n
Sol.
Money saved by Deepak in 1st week = 10Rs
Money saved by Deepak in 2nd week = 10 + 2.25 = 12.25Rs
Money saved by Deepak in 3rd week = 12.25 + 2.25 =14.50Rs
Therefore, the above given situation forms an A.P: 10, 12.25, 14.50, 16.75…………..
Here, a = 10
d = 2.25
an = 46
Since, an= a+(n-1)d
Therefore 46= 10+(n-1)2.25
2.25n=46-10+2.25
Therefore n= 17
Hence in 17th week if that year Deepak was able to increase his weekly savings to Rs 46.
Q.12 Find 26th term from the last term of A.P: 7, 12, 17,22………..187.
Sol.
Here, a = 187 (In this case, last term will be first term)
d= -5 (since the given A.P is reversed, therefore d = 12 -17 = 7 – 12 = -5)
And, n = 26
Since, an= a + (n – 1)d
Therefore an = 187 + (26 – 1)×-5
an= 62
Therefore 26th term from the last term is 62.
Q.13 Consider two A.Ps :- 9, 15, 21, 27,…….. and 63,66, 69, 72,…….. Find the value of n, such that the nth term of both the A.P’s is equal.
Sol.
For First A.P, a = 7 and d = 16-7 = 8
Since an = a+(n-1)d
Therefore an= 9+(n-1)6
(Or) an= 6n+3
For second A.P, a = 9 and d = 15-9 = 6
Since, an = a + (n – 1)d
Therefore, an = 63 + (n – 1)×3
(Or) an = 3n +60
Now, according to the given condition:
3n+60=6n+3
Therefore n = 19
Therefore, 19th term of both the A.P’s is equal.
Q.14 Find how many three digit numbers are divisible by 9
Sol.
First 3- digit number divisible by 9 = 108
Second 3- digit number divisible by 9 = 117
Similarly last 3-digit number divisible by 9 = 999
Hence it forms an A.P with d = 9,
108, 117, 126……………999
Now, from the above A.P:
a= 108, d= 9, an= 999
n=??
Since, an= a+ (n-1)×d
Therefore 999= 108+ (n-1)×9
9n=999-108+9
Therefore n= 900
Hence, there are 900 three digit numbers that are divisible by 9.
Formula for Sum of first n terms:
Let us suppose that there are n terms in an A.P, therefore sum of first n terms of an A.P is given by Sn = n2 [2a+(n-1)d]
Here, a = first term, d = common difference and n = number of terms whose sum is to be determined.
REMEMBER: an = Sn – Sn–1
Example-1: In an A.P -2, 5, 12, 19,……… Find the sum of first 8 terms.
Sol.
Here, a = -2 and d = (12-5) = 7 and n = 8
Since, Sn = n2 [2a+(n-1)d]
Therefore Sn = 82 [2×-2+(8-1)7]
= 4 × [45]
Therefore sum of first 8 terms = 180.
Example – 2 How many terms of an A.P 34, 43, 52, 61,………………… must be taken so that there sum is equal to 1002.
Sol.
Given, a = 34, d = 9 and Sn = 1002
Since, Sn = n2 [2a+(n-1)d]
Therefore, Sn = 1002 = n2 [2×34+(n-1)×9]
1002×2 = n×[59 + 9n]
9n2 + 59n – 2004 = 0
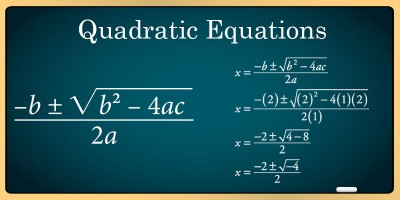
Now, from the above quadratic equation: a = 9, b = 59 and c = -2004
Substituting the values of a, b and c in quadratic formulae we get:
n=−(59)+(59)2−4(9×−2004)√2×9 and n= −(59)−(59)2−4(9×−2004)√2×9
n=−59+3481+72144√18 and n=−59−3481+72144√18
n=−59+75625√18 and n=−59−75625√18
n = −59+27518 and n= −59+27518
n=12 and n= 12
Therefore, the sum of first 12 terms in the given A.P will be 2004.
Example-3 Find the sum of first 100 odd positive integers.
Sol.
Odd positive integers can be written as: 3, 5, 7, 9, 11……
Here a = 3, d = 2 and n = 100
Since Sn = n2 [2a+(n-1)d]
Therefore, Sn = 1002 [2×3+(100-1)×2]
= 50[6+198]
= 10200
Therefore the sum of first 100 odd positive integers = 10200
Example – 4 The nth term of any particular series in given by (3n – 5). Find sum of first 16 terms.
Sol.
Given, an = 3n – 5
Therefore, a1 = 3×1 – 5 = -2
a2 = 3×2 – 5 = 1
a3 = 3×3 – 5 = 4
Therefore, the series becomes -2, 1, 4 . . . . .
Since [1 – (-2)] = (4 – 1) = 3 = d
Hence, the above series forms an A.P with a = -2 and d = 3
Since, the sum of first n terms is given by: Sn = n2 [2a+(n-1)d]
Sn = 162 [2×-2+(16-1)×3]
= 8(-4 + 45)
= 328
Therefore the sum of first 16 terms is 328
Exercise 5.3
Q1. Find the sum of the following given AP’s:
(i) 3, 7, 11, 15 . . . . . . . up to 12 terms.
Sol.
Here a = 3, d = (7 – 3) = 4 and n = 12
Since, the sum of first 12 terms is given by: Sn = n2 [2a+(n-1)d]
Therefore, Sn = 122 [2×3+(12-1)×4]
= 6×(6 + 44) = 300
Therefore, the sum of first 12 terms is 300.
(ii) –17, –30, –43. . . . . . . . up to 16 terms.
Sol.
Here a = -17, d = [-30 – (-17)] = -13 and n = 16
Since, the sum of first n terms is given by: Sn = n2 [2a+(n-1)d]
Therefore, Sn = 162 [2×(-17) + (16-1)× -13]
= 8×(-34 – 195) = -1832
Therefore, the sum of first 16 terms is -1832
(iii) 0.8, 1.3, 1.8. . . . . . . up to 50 terms.
Sol.
Here a = 0.8, d = (1.3 – 0.8) = 0.5 and n = 50
Since, the sum of first n terms is given by: Sn = n2 [2a+(n-1)d]
Therefore, Sn = 502 [2×0.8+(50 – 1)×0.5]
= 25×(1.6 + 24.5) = 652.5
Therefore, the sum of first 50 terms is 652.5
Q.2 The first term of an A.P is 11 and last term of an A.P is 91. If sum of this A.P is 867, find the total number of terms in that A.P and common difference.
Sol.
Given, a = 11, l = 91 (last term) and Sn = 867
Since, Sn = n2 [2a+(n-1)d]
(or) Sn = n2 [a+a+(n-1)d]
(or) Sn = n2 [a + l] (since an = a +(n – 1)d = l)
Therefore, 867 = n2 [11+91]
867×2 = 102n
Therefore, n = 17
Since l = a + (17 – 1)d (since there are total 17 terms)
Therefore, 91 = 11 + 16d
d = 5
Therefore, total numbers of terms are 17 and common difference is 5
Q.3 First term of an A.P is 17 and last term of an A.P is 407. How many terms are there in an A.P also find its sum if common difference is 13.
Sol.
Given, a = 17, d = 13, l = 407 (last term) = an
Since, an = a + (n – 1)d
Therefore, 407 = 17 + (n – 1)×13
13n = 407 + 13 – 17
Therefore, n = 31
Since Sn = n2 [2a + (n – 1)d]
(or) Sn = 312 [a+a+(n – 1)d]
(or) Sn = 312 [a + l] (since an = a +(n – 1)d = l)
Therefore, Sn = 312 [17+407]
Sn = 6572
Therefore, total number of terms in the given A.P are 31 and there sum is 6572.
Q4. 2nd and 4th terms of an A.P are 30 and 44 respectively. Find the sum of first 45 terms.
Sol.
Given, a2 = 30 and a4 = 44
Since, an = a + (n – 1)d
Therefore, a2 = a + (2-1)d
(or) 30 = a + d . . . . . . . . . . . (1)
And, a4 = a + (4 – 1)d
44 = a + 3d . . . . . . . . . (2)
Subtracting equation (1) from equation (2) we get
14 = 2d
Therefore d = 7, on putting value of d in equation (2) we get
44 = a + 3 × 7
Therefore a = 23
Since, the sum of first n terms is given by: Sn = n2 [2a+(n-1)d]
Therefore, for n = 45
Sn = 452[2×23+(45 – 1)×7]
Sn = 452[354]
Therefore, Sn = 7965
Hence, the sum of first 45 terms is 7965
Q.5 If the sum of first n terms of any particular A.P is given by 2n2 – 6n. Find first five terms.
Sol.
Given, Sn = 2n2 – 6n
Therefore, S1 = -4 (2×12 – 6×1)
S2 = -4 (2×22 – 6×2)
Similarly, S3 = 0, S4 = 8, S5 =20
Since an = Sn – Sn –1
Therefore, a1 = S1 = -4
a2 = S2 – S1 = -4 – (-4) = 0
a3 = S3 – S2 = 0 – (-4) = 4
a4 = S4 – S3 = 8 – 0 = 8
a5 = S5 – S6 = 20-8 = 12
Therefore, first five terms of an A.P are: -4, 0, 4, 8, 12
Q.6 A total sum of Rs 7500 is to be used to give 6 cash prizes to students of Saint Marry School for their overall excellent academic performance. If cost of each prize is Rs 200 less than its preceding prize, find the value of each of the prizes.
Sol.
Let the cost of 1st prize be = a
Given, d = -200 (since each prize is Rs 200 less than its preceding prize)
and Sn = 7500
Since, the sum of first n terms is given by: Sn = n2 [2a+(n-1)d]
Therefore, for n = 6
7500 = 62[2×a + (6 – 1)×-200]
7500 = 3(2a – 1000)
2500 = 2a – 1000
a = 1750
Therefore, cost of prizes will be:
1st prize = a = 1750Rs,
2nd prize = (a – d) = 1550Rs,
Similarly, 3rd prize = 1350Rs, 4th prize = 1150Rs, 5th prize = 950Rs and 5th prize = 750Rs
Q.7 In a construction contract there is a penalty for delay in completion of a contract beyond a certain date. For the delay of one day it is Rs 500, for 2nd day it is Rs 600, and Rs 700 for the 3rd day and so on, If the penalty for each succeeding day is increased by Rs 100 and the job was delayed for 28 days. Find how much money the contractor has to pay as penalty??
Sol.
Given, a = 500 (since, penalty on 1st day is 500 Rs)
d = 100 (since, penalty increases by Rs 100 for each succeeding day.)
And n = 28
Since, the sum of first n terms is given by: Sn = n2 [2a+(n-1)d]
Therefore, for n = 6
Sn = 282[2×500 + (28 – 1)×100]
= 14(3700)
= 51800 Rs
Therefore, the contractor paid Rs 51800 as penalty.
Q.8 Fauzia took part in a sports event; there was a race in which 8 balloons were placed in a straight line. A basket was placed at a distance of 5m from the first balloon and all 8 balloons are 2m apart. Fauzia started from basket, picks up the nearest balloon and runs back to the basket to drop it and she continues in the same way until all balloons are in the basket. Find the total distance Fauzia covered.
Sol.

To pick up 1st balloon and drop it into basket Fauzia covered distance = 2×5m = 10m
Similarly for 2nd balloon, distance covered by Fauzia = 2(5+2) = 14m
Similarly for 3rd balloon, distance covered by Fauzia = 2(5+2+2) = 18m
Since there are 8 balloons therefore, n = 8
Here, a = 10 and d = (18-14) = 4
Since, the sum of first n terms is given by: Sn = n2 [2a+(n-1)d]
Therefore, for n = 8
Sn = 82[2×10 + (8 – 1)×4]
= 4(48)
= 192m
Therefore, total distance covered by Fauzia = 192m
Q.9 The product of three numbers in an A.P is -10 and their sum is 6. Find the numbers.
Sol.
Let the numbers be (a – d), a, (a + d)
Now, according to the given conditions:
(a – d) + a + (a + d) = 6
3a = 6, therefore a = 2
And, (a – d)× a ×(a + d) = -10
(2 – d)(2)(2 + d) = -10
4 – d2 = -5
d2 = 9
Therefore d = 3 and d = -3
Now, if d = 3
Then the numbers are: [ -1, 2, 5 ]
And, if d = -3
Then the numbers are: [ 5, 2, -1]
Extra Questions
LAQ
QUESTIONS-:
- In an A.P., given pn=4,q=2,An=−14,, find ‘n’ and p.
Ans.- Given-: pn=4,q=2,An=−14,
As we know that, pn = p + (n-1)q
∴ p + (n-1)2 + 4
⇒ p + 2n – 2 = 4
⇒ p + 2n = 4 + 2
⇒ p +2n = 6 ……(i)
Also, we know that An = n2[2p+(n−1)q]
∴n2[2p+(n−1)2]=−14
⇒n[2p+(n−1)2]=−14×2
⇒n[2p+2n−2]=−28
⇒n[p+(p+2n)−2]=−28
⇒n[p+6−2]=−28…[Using(i)]
⇒n[p+4]=−28
⇒n=−28p+4…(ii)
Now, putting the value of n = n=−28p+4 in equation (i),
We get
p+2(−28p+4)=6
⇒p−56p+4=6
⇒p(p+4)−56p+4=6
⇒p2+4p−56=6(p+4)
⇒p2+4p−56=6p+24
⇒p2+4p−56−6p−24=0
⇒p2−2p−80=0
⇒p2−10p+8p−80=0
⇒p(p−10)+8(p−10)=0
⇒(p−10)(p+8)=0
∴p=10,orp=−8
Now, putting the value of p = 10 in (i), we have
10 + 2n = 6
⇒2n=−4
⇒n=−2, which is not possible
Now putting the value of p = -8 in (i), we get
-8 + 2n = 6
⇒2n=14 ⇒n=7
Hence, p = -8 and n = 7
- A contract on construction job specifies a penalty for delay of completion beyond a certain date as follows -: Rs 100 for the first day, Rs 150 for the second day, Rs 400 for the third day, etc.; the penalty for each succeeding day being Rs 50 more than for the preceding day. How much does a delay of 30 days cost the contractor?
Ans.- Since the penalty for each succeeding day is Rs 50 more than for the preceding day. Therefore, the amount of penalty for different days form an A.P. with first term p (= 100) and the common difference q (= 50). We have to find how much a delay of 30 days costs the contractor. In other words, we have to find the sum of 30 terms of the A.P.
∴Requiredsum=3022×100+(30−1)×5 [ becauseAn=n2[2p+(n−1)q] ⇒Requiredsum=15(200+29×50)
⇒Requiredsum=15(200+1450) ⇒Requiredsum=15×1650=24750
Thus, a delay of 30 days will cost the contractor of Rs. 24750.
- A sum of Rs. 560 is to be used to give seven cash prizes to students of a school for their overall academic performance. If each prize is Rs. 20 less than its preceding prize, find out the value of each of the prizes.
Ans.- Total amount of seven prizes = Rs.560
Let us assume the value of first prize be Rs.p
By the given condition, we have
P, p-20, p-40,…
Now, m2−m1=p−20−p=−20
Similarly,m3−m2=p−40−p+20=−20 Sincem2−m1=m3−m2=−20,whichisconstant.
Therefore, it is an A.P. with common difference (q) = -20
Let K = p + (p-20) + (p-40) + … upto 7 terms
Here, m = p, q =-20 and n = 7
Now, Kn=n2[2m+(n−1)q]
∴K7=72[2p+(7−1)(−20)]
⇒K7=72[2p+(6)(−20)]
⇒560=72[2p−120]
[∴Totalamountof7prizes=Rs.560=K7]
⇒2p−120=560×27
⇒2(p−60)=160
⇒p−60=1602=80
⇒p=80+60=140
Hence, the amount of each prize (in rs.) respectively is-:
140, 140-20, 140-40, 140-60, 140-80, 140-100, 140-120
i.e, Rs.140, Rs.120, Rs.100, Rs.80, Rs.60, Rs.40, Rs.20 respectively.
- A sum of Rs. 1000 is to be used to give ten cash prizes to students of a school for their overall academic performance. If each prize is Rs. 20 less than its preceding prize, then find out the value of each of the prizes.
Ans.- Here, the total amount of ten prizes is = Rs.1000
Now, Let us assume the value of first prize be RS. P
So, by the given condition, we have prizes of denomination (in Rs.) p, p – 20, p – 40, …
Now, m2−m1=p−20−p=−20
m3−m2=p−40−p+20=−20
Since, m2−m1=m3−m2=−20, which is constant.
Therefore, it is an A.P. with common difference (q) = -20
Hence, A10=p+(p−20)+(p−40)+….upto10terms
Here, m = p, q = -20 and n = 10
Now, An=n2[2m+(n−1)q][ becauseA10=1000]
⇒1000=5(2p−180) ⇒1000=10p−900 ⇒10p=1900 ⇒p=190
Hence, amount of each prize (in Rs.) respectively is 190, (190-20), (190-40), (190-60), (190-80), (190-100), (190-120), (190-140), (190-160) and (190-180)
i.e., Rs.190, Rs.170, Rs.150, Rs.130, Rs.110, Rs.90, Rs.70, Rs.50, Rs.30 and Rs.10.
- A spiral is made-up of successive semicircles, with centres alternately at P and Q, starting with centre at P, of radii 0.5 cm, 0.1 cm, 1.5 cm, 2.0 cm, … as shown in fig. What is the total length of such a spiral made-up of 13 consecutive semicircles ? (Takeπ=227)
Ans.- Since radii od semicircles are 0.5 cm, 1.0 cm, 1.5 cm, 2.0 cm …
Now, the length of semicircle (l1) = Perimeter of first semicircle = πr=227×0.5cm
=227×510=117cm
So now, the length of the second semicircle(l2) = Perimeter of second semicircle
= πr=227×1.0cm=227cm
Similarly, we can get the length of other semicircle as,
l3=337cmandl4=447cm and so on upto 13 semicircles.
Let us assume ‘A’ be the total length of all semicircles.
i.e., p= l3=337cmandl4=447cm … upto 13 terms
Here, p=117,q=227−117=117andn=13
Since, An=n2[2p+(n−1)q]
∴A13=132[2×117+(13−1)×117] =132[227+1327] =132×1547=143cm
- 200 logs are stacked in the following manner : 20 logs in the bottom row, 19 in the next row, 18 in the row next to it and so on. Find out in how many rows are 200 logs placed and how many logs are in the top row ?
Ans.- Suppose 200 logs are stacked in ‘s’ rows.
There are 20 logs in the first row and the number of logs in a row is one less than the number of logs in the preceding row. So, number of logs in various rows form an A.P. with first term p (=20) and the common difference q (= -1). As there are 200 logs in all rows.
∴ Sum of ‘s’ terms of an A.P. with p = 20 and q = -1 is 200
⇒s2{2p+(s−1)q}=200 ⇒s2{2×20+(s−1)×−1}=200 ⇒s2(40−s+1)=200 ⇒n(41−s)=400 ⇒s2−41s+400=0⇒(s−25)(s−16)=0 ⇒s=16ors=25
Now, If s =25, then number of logs in 25th row is equal to 25th terms of an A.P. with first term 20 and the common difference (-1).
∴ number of logs in 25th row = p + 24q = 20 – 24 = -4
Clearly, this is not meaningful.
∴ s = 16
Thus, logs are placed in 16 rows.
Number of logs in top row = Number of logs in 16th row
= 16th term of an A.P. with p = 20 and q = -1
Hence, there are 5 logs in the top row.
- The sum of 4thand 8th terms of an A.P. is 24 and the sum of 6th and 10th terms is 44. Calculate A.P.
Ans.Let us assume ‘p’ be the first term and ‘q’ be the common difference of the required A.P.
∴A4+A8=24
⇒42{2p+(4−1)q}+82{2p+(8−1)q}=24
⇒2{2p+3q}+4{2p+7q}=24
⇒4p+6q+8p+28q=24⇒12p+34q=24
Or, 6p + 17q = 12 ….(i)
And, A6+A10=44
⇒62{2p+(6−1)q}+102{2p+(10−1)q}=44
⇒3{2p+5q}+5{2p+9q}=44
⇒6p+15q+10p+45q=44⇒16p+60q=44
Or, 4p+15q = 11 ….(ii)
Now, multiplying (i) by 2 and (ii) by 3, we get
12p + 34q = 24 …..(iii)
12p + 45q = 33 ……(iv)
Now, Subracting (iii) from (iv), we have 11q = 9 or q = 911
From (ii), we have 4p+15×911=11
⇒4p=11−13511=121−13511=−1411 ⇒p=−1411×14=−722
Hence, the required A.P. is -:
−722,−722+911,−722+1811, ….
Or, −722,12,2922, ….
- The 17thterm of an A.P. is 5 more than twice its 8th If the 11th term of the A.P. is 43, then calculate its ‘nth’ term.
Ans.- Given, p11=43
P + 10q = 43 ….(i)
[Usingpn=p+(n−1)q]
And, p17=5+2p8
P + 16q = 5 + 2[p + 7q]
[Usingpn=p+(n−1)q]
P + 16q = 5 + 2p + 14q
⇒ p – 2q = -5 ….(ii)
Subtracting (ii) from (i), we get
p+10q – (p-2q) = 43 – (-5)
p+10q-p+2q = 43+5 ⇒12q=48⇒q=4 …(iii)
From (iii) and (i), we have
P + 40 = 43 ⇒p=3
We know that, pn=p+(n−1)q
pn=3+(n−1)4=3+4n−4=4n−1
Hence, the ‘nth’ term of given A.P. is 4n – 1.
- Determine the common difference of an A.P. whose first term is 5 and the sum of it first four term is half the sum of the next four terms.
Ans.- Given, First term = p = 5
And, A4=12(thesumofp5,p6,p7,p8)
= 12(A8−A4)⇒2A4=A8−A4⇒3A4=A8
A4=42[2p+(4−1)q] [UsingAn=n2(2p+(n−1)q)]
= 2 (10 + 3q) = 20 + 6q
A8=82(10+7q)=4(10+7q)=40+28q
So, 3A4=3(20+6q)=60+18q
3A4=A8 (According to question)
60 + 18q = 40 + 28q
⇒10q=20⇒q=2
∴ The common difference (q) = 2.
- The sum of first six terms of an A.P. is 42. The ratio of its 10thterm to its 30th term is 1 : 3. Determine the first and the 13th term of the A.P.
Ans.- Let us assume ‘p’ be the first tern and ‘q’ be the common difference.
Here, A6=42
62{2p+(6−1)q}=42
2p+5q = 14 …..(i)
Also, p10p30=13
⇒p+9qp+29q=13 ⇒3p+27q=p+29q ⇒3p−p=29q−27q
⇒2p=2q …..(ii)
Subtracting (ii) from (i), we get
5q + 2q = 14
7q = 14
q = 2
Now, from (ii), we have -:
2p−2×2=0⇒p=2
So now, p13=p+12q=2+12×2⇒2+24=26
Therefore, the first term of the given A.P. is 2 and its 13th term is 26.
- If the sum of first ‘s’ terms of an A.P. is ‘n’ and the sum of first n terms is ‘s’, then prove that the sum of its first (s+n) terms is –(s+n).
Ans.- Let us assume ‘p’ be the first term and ‘q’ be the common difference
∴As=n ⇒s2[2p+(s−1)q]=n
⇒2p+(s−1)q=2ns ….(i)
And, An=s
⇒n2[2p+(n−1)q]=s
⇒2p+(n−1)q=2sn ….(ii)
Subtracting (ii) from (i), we get,
(s – 1 – n + 1)q = 2ns−2sn
(s – n)q = 2(n2−s2)sn = 2(n+s)(n−s)sn
⇒q=−2(s+n)sn …..(iii)
Also, from (i), we get -: 2p=2ns−(s−1)q …..(iv)
And now, the sum of first (s + n) terms
= s+n2[2p+(s+n−1)q]
= s+n2[2ns−(s−1)q+(s+n−1)q] [Using (iv)]
= s+n2[2ns+nq]=n(s+n)2[2s+q]
= n(s+n2)[2s−2(s+n)sn] [Using(iii)]
= n(s+n2)[2n−2s−2nsn]
= n(s+n2)[−2ssn]=−(s+n)
- In an A.P., the sum of first terms is given by -:
An=3n22+5n2. Determine the 25th term of the A.P.
Ans.- Here, An=3n22+5n2=3n2+5n2
⇒An−1=3(n−1)2+5(n−1)2 ⇒3n2+3−6n+5n−52 ⇒3n2−n−22
Now,
pn=An−An−1
=3n2+5n2−3n2−n−22
=5n+n+22=6n+22=2(3n+1)2=3n+1
pn=3n+1
∴p1=3×1+1=4
∴p2=3×2+1=7
∴p3=3×3+1=10
Hence, the common difference (q) = 7 – 4 = 3
∴p2=3×2+1=7
- A manufacturer of T.V. sets produced 600 sets in the third year and 700 sets in the seventh year. Assuming that the production increase uniformly by a fixed number of every year, calculate-:
(i) The production in the 1st year.
(ii) The production in the 10thyear.
(iii) The total production in first 7years.
Ans.- Let us assume the number of sets produced in 1st year be ‘x’ and ‘y’ be the increase in population every year.
We are given, x + 2y = 600 …..(i)
And, x + 6y = 700 …..(ii)
Now subtracting equation (i) from (ii), we get
4y = 100 or y = 25
Now, Substituting y = 25 in equation (i), we get
x = 550
(1) Production in the first year = x = 550
(2) Production in 10th year = x+9y = 550+(9)(25) = 550 + 225 = 775
(3) Total production in first 7years = x + (x+y) +(x+2y) + …..+ (x+6y)
We know that,
Sum = n2[2x+(n−1)y]
Here, x = 550, y = 25, n = 7
∴Sum=n2[2x+(n−1)y]
= 72[2×550+(7−1)(25)]
= 72[1100+(6)(25)]
= 72[1100+(6)(25)]
= 4375
∴ Total production in first 7 years = 4375
SAQ 2 MARK
QUESTIONS-:
- The amount of money in the account every year, when rs 5000 is deposited at compound interest at 5% per annum.
Ans.- Here, Principal = Rs.5000
Rate of interest = 5% per annum
Therefore, Annual (A) = 5000(1+5100)
Now, the sequence becomes 5000(1+5100),
5000(1+5100)2,5000(1+5100)3,…
Here, a2−a1≠a3−a2
Therefore, the given amount of money in the account every year does not form an A.P.
- In which of the following situations, does the list of numbers involved make an arithmetic progression and why ?
(i) The amount of air present in a cylinder when a vaccum pump removes 16 of air remaining in the cylinder at a time.
(ii) The cost of digging a well after every metre of digging, when it costs Rs.250 for the first metre and rises by Rs. 100 for each subsequent metre.
Ans.- Let us assume, x1= p units
∴x2=p−16p=56punits ∴x3=56p−16×56p=56p−536p=2536punits
Similarly, ∴x4=2536p−16×2536p=2536p−25216p=125216punits
So, the list of numbers is p, 56p,2536p,125216p,…
Now here, x2−x1≠x3−x2
Hence, it is not an A.P.
- Write first four terms of the A.P. whose first term is 20 and common difference is 20.
Ans.- In this case we have, p= 20, q= 20
∴Firstterm(p1)=p=20
Secondterm(p2)=p1+q=20+20=40
Similarly,Thirdterm(p3)=p2+q=40+20=60
Now,Fourthterm(p4)=p3+q=60+20=80
Hence, the required four terms are 20, 40, 60, 80.
4.Write the first four terms of the A.P. whose first term is -1 and the common difference is 12.
Ans.- Here we have, p=-1, q=12
So, Firstterm(p1)=p=−1
Secondterm(p2)=p1+q=−1+12=−12
Similarly,Thirdterm(p3)=p2+q=−12+12=0
Similarly,Fourthterm(p4)=p3+q=0+12=12
Hence, the required four terms are −1,−12,0,12.
- Write four terms of the A.P. whose first term is -1.25 and the common difference is -0.25.
Ans.- Here we have, p=-1.25, q=-0.25
So, Firstterm(p1)=p=−1.25
Secondterm(p2)=p1+q=−1.25=(−0.25)=−1.25−0.25=−1.50
Similarly,Thirdterm(p3)=p2+q=−1.50+(−0.25)=−1.50−0.25=−1.75
Similarly,Fourthterm(p4)=p3+q=−1.75+(−0.25)=−1.75−0.25=−2.0
Hence, the required four terms are -1.25, -1.50, -1.75, -2.0
- Write first four terms of the A.P. when the first term ‘p’ and the common difference ‘q’ are given as follows-:
(i) p = -2, q = 0 (ii) p = 4, q = -3
Ans.- (i)Here, First term = p = -2
Second term = p+q = -2+0 = -2
Third term = p+2q = -2+2(0) =-2
Similarly, Fourth term = p+3q = -2+3(0) =-2
Hence, the required four terms are -2, -2, -2, -2.
(ii) We have, First term = p = 4
Second term =p+q =4+(-3)=1
Third term = p+2q = 4+2(-3)= -2
Similarly, Fourth term = p+3q= 4+3(-3)= -5
Hence, the required four terms are 4, 1, -2, -5.
- For the following A.P., write the first term and the common difference of the following 3, 1, -1, -3, …
Ans.- Here A.P. is 3, 1, -1, -3, …
So, the first term p1= 3
Now, p2−p1=1−3=−2,
p3−p2=−1−1=−2, p4−p3=−3−(−1)=−3+1=−2
i.e., pn+1−pnissameeverytime.
So therefore, for the given A.P. common difference (q) = -2
- For the following A.P., write the first term (p1) and the common difference (q) 13,53,93,133,…
Ans.- Here the given A.P. is 13,53,93,133,…
So, First term ((p1))= 13
Now, p2−p1=53−13=5−13=43
p3−p2=93−53=9−53=43 p4−p3=133−93=13−93=43
i.e. , pn+1−pnissameeverytime.
So therefore, for the given A.P. common difference(q)=43
- For the following A.P.s, write the first term (p) and the common difference (q)-:
(i) -5, -1, 3, 7, … (ii) 0.6, 1.7, 2.8, 3.9, ….
Ans.- Given here, First term (p) = -5
Now the common difference (q) = -1-(-5) = 3-(-1) = 7-3 = 4
∴ Thus, for the given A.P., first term (p) is -5 and the common difference (q) is 4.
(ii) Given here, First term (p) = 0.6
Similarly, the common difference (q) = 1.7 – 0.6 = 2.8 – 1.7 = 3.9 – 2.8 = 1.1
∴ Thus, for the given A.P., first term (p) is 0.6 and the common difference (q) is 1.1.
- Find whether the following list of numbers form an A.P If they form an A.P., find the common difference (q) and write three more terms p,p2,p3,p4,…
Ans.- Here the given list of numbers is p,p2,p3,p4,…
So, first term (p1) = p
Now, p2−p1=p2−p=p(p−1)
p3−p2=p3−p2=p2(p−1) p4−p3=p4−p3=p3(p−1)
As, p2−p1≠p3−p2
Hence, therefore the given list of numbers does not form an A.P.
- Find whether the following list of numbers form an A.P. If they forman A.P., then find the common difference (q) and write three more terms 3, 3+2–√, 3+22–√, 3+32–√,…
Ans.- Here the given list of numbers is -: 3, 3+2–√, 3+22–√, 3+32–√,…
Now, first term (p1) = 3
So, p2−p1=3+2–√−3=2–√
p3−p2=3+22–√−(3+2–√)
= =3+22–√−3−2–√=2–√
Similarly,
p4−p3=3+32–√−(3+22–√)
= p4−p3=3+32–√−3−22–√=22–√
So here it is concluded that, pn+1−pnissameeverytime.
Hence, the given list of numbers form an A.P. in which, q = 2–√
Now the next three more terms are -:
3+32–√+2–√=3+42–√ 3+42–√+2–√=3+52–√
And , 3+52–√+2–√=3+62–√
∴ Hence, the three more terms are
3+42–√,3+52–√and3+62–√
- Find whether the following list of numbers form an A.P. If they form an A.P., find the common difference (q) and write three more terms 2–√,8–√,18−−√,32−−√,…
Ans.- Here the given list of numbers is -: 2–√,8–√,18−−√,32−−√,…
Now here, the first term (p1)=2–√
So, p2−p1=8–√−2–√=4×2−−−−√−2–√
= 22–√−2–√=2–√
p3−p2=18−−√−8–√=9×2−−−−√−4×2−−−−√
= 32–√−22–√=2–√
p4−p3=32−−√−18−−√=16×2−−−−−√−9×2−−−−√
= 42–√−32–√=2–√
So here it is concluded that, pn+1−pnissameeverytime.
Hence, the given list of numbers form an A.P. in which, q = 2–√
Now the next three more terms are -:
32−−√+2–√=16×2−−−−−√+2–√=42–√+2–√
= 52–√=25×2−−−−−√=50−−√
50−−√+2–√=25×2−−−−−√+2–√=52–√+2–√
= 62–√=36×2−−−−−√=72−−√
72−−√+2–√=36×2−−−−−√+2–√=62–√+2–√
= 72–√=49×2−−−−−√=98−−√
∴ Hence, the three more terms are -:
50−−√,72−−√and98−−√
- Find whether the following list of numbers form an A.P. If they form an A.P., find the common difference(q) and write three more terms -: 12,52,72,73,…
Ans.- Given list of numbers is-: 1,25,49,73,…
Here, the first term (p1)=1
Now, p2−p1=25−1=24
p3−p2=49−25=24
Similarly, p4−p3=73−49=24
So here it is concluded that, pn+1−pnissameeverytime.
Hence, the given list of numbers form an A.P. in which, q = 24
Now the next three more terms are -: 73+24 = 97
97+24 = 121
And , 121+24 = 145
Hence, the three more terms are 97, 121 and 145.
- Fill in the blanks p = 15, q = 9, n = 12, pn = …
Here, ‘p’ is the first term, ‘q’ is the common difference and pn = nnt term of an A.P.
Ans.- Given, p = 15, q = 9, n = 12, pn = ?
As we know that,
pn = p+(n−1)q
p12 = 15 + (12-1)9
= 15 + 99 = 114
Hence, p12 =114
- Fill in the blanks p = 20, q = 4, n = …, pn =12
Ans.- Here, ‘p’ is the first term,’ q’ is the common difference and pn is the nth term of an A.P.
Given, p = 20, q = 4, pn = 12, n = …..
As we know that-: pn = p + (n – 1)q
⇒ 12 = 20 + (n – 1)4
⇒ 12 = 20 + 4n-4
⇒ 4n = 12 – 20 + 4 = -4
⇒ n = -1
∴Hence,n=−1
- In the following A.P., find the missing term-: 4, ?, 32.
Ans.- Here given A.P. is 4, ?, 32.
Let us assume the missing number be ‘p’
i.e., 4, p, 32 is an A.P.
∴ p – 4 = 32 – p
⇒ p + p = 32 + 4
⇒ 2p = 36
⇒ p = 18
Hence, the missing term is 18.
- How many terms are there in A.P. 7, 16, 25, …, 542 ?
Ans.- Given here, pn=540
P + (n+1)q = 542
⇒7+(n−1)9=542 ⇒9n–2=542 ⇒9n=540 ⇒n=60
Hence, the number of terms is 60.
- Find the value of s, if the numbers q, 2q + s, 3q +6 are three consecutive terms of an A.P.
Ans.- Here, q, 2q + s, 3q +6 are in A.P.
∴ 2q+s-q = 3q+6-2q-s
⇒ q+s = q+6-s
⇒ 2s = 6
⇒ s = 3
- Find the common difference (q) of an A.P. whose first term is 12 and the 8thterm is 176. Also mention its 4th
Ans.- Let us assume p8=176
⇒p+7q=176
⇒12+7q=176
⇒7q=176−12
⇒7q=176−12
So now, p4=p+3q=12+3(13)=12+1=32
- The angles of a triangle are in A.P. The greatest angle is twice the least. Find all the angles of the triangle.
Ans.- Let us assume the three angles of the triangle be p, q and r, where p is the smallest angle and r is the largest angle.
According to the given condition-: r = 2p ….(i)
Also, p + q + r =180∘ ….(ii)
[ becausesumofanglesofthe:triangleis180∘]
Here, 2q = p + r ….(iii)
⇒ = 2q = p + 2p ….[using (i)]
⇒ = 2q = 3p
⇒q=32p
Now, from (ii), we have -: p+32p+2p=180∘
⇒92p=180∘ ⇒p=180∘×29=40∘ ∴q=32×40∘=60∘ andr=2(40∘)=80∘
Hence, angle of the triangle are 40∘,60∘,80∘respectively.
- Determine the 2ndterm of an A.P. whose 6th term is 12 and 8th term is 22.
Ans.- Here, p6=12⇒p+5q=12…(i)
And, p8=22⇒p+7q=22…(ii)
Now, subtracting (i) from (ii), we have
2q = 10
⇒ q = 5
Now, from (i), we have
P + 5(5) = 12
⇒ p = 12 – 25
⇒ p = -13
∴p2=p+q=−13+5=−8
- Find the number of all three-digit natural numbers which are divisible by 9.
108, 117, 126, 135, ….., 999
Ans.- Here, p = 108, q = 9 and pn=999
P + (n – 1)q = pn
108 + (n-1)9 = 999
⇒ (n – 1)9 = 999 – 108
⇒ (n – 1) = 8919=99
⇒ n = 99 + 1 = 100
- The 8thterm of an A.P. is equal to three times its third term. If its6th term is 22, then find the A.P.
Ans.- Let us assume ‘p’ and ‘q’ be the first term and the common difference of the required A.P.
∴ p6 = p + 5d = 22 ….(i)
And, p8=3(p2)
⇒p+7q=3(p+2q) ⇒p+7q=3p+6q
⇒q=2p …..(ii)
From the equations (i) and (ii), we have
P + 5(2p) = 22
⇒11p=22 ⇒p=2
Similarly from (ii), we have q = 2(2) = 4
Hence, the required A.P. is 2, 6, 10, 14,….
- Find the 20thterm from the last term (end) of the A.P. -: 3, 8, 13, ….,253.
Ans.- We have an A.P. -:3, 8, 13, …, 253.
Here, p = 3, last term x = 253 and the common difference q = 5
Now, ‘nth’ term from end = x + (-n + 1)q
We have 20th term from end as
= 253 + (-20+1)5 = 253 – 95 = 158.
OR
20TH term from the last term of the given A.P.
= 20th term of the A.P. 253, 248, 243, ….
= p + 19q
= 253 + 19 (-5)
253 – 95 = 158
- The 9thterm of an A.P. is equal to 6 times its second term. If its 5th term is 22, then find the A.P.
Ans.- Let us assume ‘p’ and ‘q’ be the first term and common difference of the required A.P.
Here, p5=22
⇒p+4q=22 …(i)
And, p9=6p2
⇒p+8q=6(p+q) ⇒p+8q=6p+6q
⇒5p=2q ….(ii)
From (i) and (ii), we have -:
p + 2(5)p = 22
⇒11p=22 ⇒p=2
From (ii), we have 5(2) = 2q ⇒q=5
Hence, the required A.P. is 2, 7, 12, 17, …
- The sum of the first 15 terms of an A.P. is 750 and its first term is 15. So find its 20th
Ans.- Here, first term p = 15 and A15=750
An=n2[2p+(n−1)q] ⇒750=152[2×15+(15−1)q] 750=152(30+14q)
750 = 225 + 105q
⇒105q=750–225 ⇒q=525105=5
We have to find 20th term
So as we know, pn=p+(n−1)q
∴p20=15+(19)5=15+95=110
- The 16thterm of an A.P. is more than twice its 8th If the 12th term of the A.P. is 47, then find out its ‘nth’ term.
Ans.- Here, p12=47…(given)
⇒p+11q=47…(i)
And, p16=1+2p8
⇒p+15q=1+2(p+7q)
So we got, P – q =-1 ……..(ii)
Subracting (ii) from (i), we get
⇒12q=48⇒q=4
Hence, p – 4 = -1 ⇒p=3 ….[using(ii)]
So, pn=3+(n−1)4=4n−1…[Usingpn=p+(n−1)q]
- Sum of the first 20 terms of an A.P. is -240, and its first term is 7. Find out its 24th
Ans.- Here, p = 7 …..(given)
A20=−240
⇒202(2×7+19q)=−240 ………..UsingAn=n2(2p+(n−1)q)
⇒10(14+19q)=−240 ⇒19q=−24−14⇒q=−2
So now, we have to find the 24th term of A.P. i.e -:
p24=7+23×−2[Usingpn=p+(n−1)q]
= 7 – 46
= -39
Arithmetic progression VSAQ
- Find next term of the A.P 3–√,12−−√,7–√,…
Ans. Here p=3–√,q=12−−√−3–√=23–√−3–√=3–√
Next term to
27−−√=27−−√+3–√=33–√+3–√=43–√
=3×16−−−−−√=48−−√
- In an A.P., 6 times the 6th term is equal to 11 times the 11 term, then find its 17th term
Ans. 6x6=11x11
6(x+5y)=11(x+10y)
⇒ 6x+30y=11x+11y
⇒ 11x−6x=−11y+30y
⇒ 11x=−80y
⇒ x=−16y
⇒x+16y=0 ⇒x17=0
- If the nth term of an A.P. is (2n =1), then find the sum of its first three terms.
Ans. Here, we have pn=2n+1
p1=2×1+1=3i p2=2×2+1=5i p3=2×3+1=7i
Adding p1+p2+p3=3+5+7=15
= sum of first three terms
s3=32(3−7)=30215
[ Using Sn=n2(p+l) ]
- Find the sum of first 20 odd natural numbers.
Ans. We know that sum of n odd natural number = n2
∴ sum of odd natural numbers =202=400
- If a+1 ,2a+1, 4a+1 are in A.P. , then find the value of a.
Ans. 2a + 1 – a – 1 = 4a- 1 – 2a – 1
⇒ a= 2a -2 ⇒ a=2
- If p-1 ,p+3 ,3p-1 are in A.P. , then find the value of p.
Sol. p + 3 – p + 1 = 3p – 1 – p – 3
⇒ 4 = 2 p – 4
⇒ p=4
- Which term of the A.P. 24,21, 28,…. Is the first negative terms ?
Ans. a_n< 0
a+(n-1 )d < 0
24 +(n-1)(-3)< 0
24-3n +3 < 0
-3n<-27
n>9
- Which term of the A.P. 1,4,7,….is 88?
Ans. .let a_n =88
a+(n-1)d=88
1+(n−1)3=88 ⇒3n−2=88 ⇒3n=90 ⇒n=30
- Which term of the A.P. 92, 88, 84, 80,… is zero.
Ans. let
an=0 a+(n−1)d=0
⇒a+(n−1)d=0
⇒92+(n−1)(−4)=0
⇒96−4n=0
⇒4n=96 ⇒n=24
- Whichterm of the A.P. 100, 90, 80,… is zero.
Ans. let
an=0 a+(n−1)d=0 ⇒100+(n−1)(−10)=0 ⇒−10n+110=0 ⇒n=0 ⇒n=11
- Find the 15th term of the A.P. x-7,x-2, x-3,….
Sol.
a15=a+14d
=x−7+14(5)
=x−7+70
=x+63
- what is the sum of all natural number from 1 to 1oo ?
Sol.
sn=n(n+1)2 s100=100(100+1)2
=100(101)2
=5050
Arithmetic Progressions Long Answer Questions
- Jassi saves $32 during the first month, $36 in the second month and $40 in the second month and $40 in the third month. If she continues to save in this manner, in how many months will she save $2000 ?
Ans. According to the question we have
32 + 36 + 40 + …n terms = 2000
n2[2×32+(n−1)4]=2000
[ because Here a= 32 ,d= 36 – 32 = 4 and no. of terms be n]
⇒32n+2n2−2n−2000=0 ⇒2n2+30n−2000=0 ⇒n2+15n−1000=0 ⇒n2+40n−25n−1000=0 ⇒(n−25)(n+40)=0
⇒ Either n – 25 =0 or n + 40 = 0
⇒ n =25 or n= -40
n cannot be =-40 ,because no. of terms cannot be –ve
Hence ,Jassi saves $2000 in 25 months.
- Solve the Equation -4 + (-1) + 2 +… + x= 437
Ans. In the LHS of the given equation, we have
a = -4 and d = -1 – (-4) = -1+4 =3 and l=x
∴ -4+(-1)+2+… +x =437
⇒n2(−4+x)=437 [ becauseSn=n2(a+l)]
⇒ n(-4+x) =874 …(i)
Also , x = -4 +(n-1)d …(ii)
[ becausean=a+(n−1)d]
From (i) and (ii) we have,
n( – 4 – 4 + (n-1)d) = 874
⇒ -8n +n(n-1)3 = 874
⇒ −8n+3n2−3n−874=0
⇒ 3n2−11n−874=0
n=11±(−11)2−4×3×(−874)√2×3 n=11±121+10488√6 =11±1036=11+1036,11−1036
=19,−926 (rejecting)
n = 19
From (ii) , we obtain
x= -4 +(19-1)3
x= -4 + 54
x= 50
- Yashraj Pal is repaying his total loan of Rs 118000 by paying every month starting with the first installment of Rs 1000.If he increases the installment by Rs 100 every month, what amount will be paid by him in the 30thinstallment? What will be the amount of loan does he have to pay after 30th installment?
Ans. Since Yashraj Pal is repaying his loan of Rs 118000 with first installment of Rs. 1000 and increases each installment by Rs. 100.
∴ his installments are Rs 1000, Rs1100 ,Rs 1200, Rs 1300,… which forms an A.P.
Here, first term is Rs. 1000 and common difference is Rs. 100
∴ 30th installment = a30=a+29d
=Rs( 1000 + 29 x 100)
=Rs (1000 +2900) = Rs. 3900
Amount paid in 30 installments = S30
⇒S30=Rs.302(2×1000+29×100)
= Rs. 15(2000 + 2900)
= Rs. 15(4900)
= Rs. 73500
Amount of loan that still have to be paid
= Rs. (118000 – 73500)
= Rs. 44500
- In an A.P., given pn=4,q=2,An=−14,, find ‘n’ and p.
Ans.- Given-: pn=4,q=2,An=−14,
As we know that, pn = p + (n-1)q
∴ p + (n-1)2 + 4
⇒ p + 2n – 2 = 4
⇒ p + 2n = 4 + 2
⇒ p +2n = 6 ……(i)
Also, we know that An = n2[2p+(n−1)q]
∴n2[2p+(n−1)2]=−14
⇒n[2p+(n−1)2]=−14×2
⇒n[2p+2n−2]=−28
⇒n[p+(p+2n)−2]=−28
⇒n[p+6−2]=−28…[Using(i)]
⇒n[p+4]=−28
⇒n=−28p+4…(ii)
Now, putting the value of n = n=−28p+4 in equation (i),
We get
p+2(−28p+4)=6
⇒p−56p+4=6
⇒p(p+4)−56p+4=6
⇒p2+4p−56=6(p+4)
⇒p2+4p−56=6p+24
⇒p2+4p−56−6p−24=0
⇒p2−2p−80=0
⇒p2−10p+8p−80=0
⇒p(p−10)+8(p−10)=0
⇒(p−10)(p+8)=0
∴p=10,orp=−8
Now, putting the value of p = 10 in (i), we have
10 + 2n = 6
⇒2n=−4
⇒n=−2, which is not possible
Now putting the value of p = -8 in (i), we get
-8 + 2n = 6
⇒2n=14 ⇒n=7
Hence, p = -8 and n = 7
Arithmetic Progressions SAQ 2 Marks
- Two Arithmetic Progressions (AP) have the same common difference. The first term of one AP is two and that of the other is seven. The difference their 10th terms is the same as the difference between their 21stterms, which is the same as the difference between any two corresponding terms? Why?
Ans. In this question, first terms of the AP’s are 2 and 7.
Let Common Difference of the 2 AP’s be d.
∴ Difference between their 10th terms
= a10−A10
= 7+9d-2-20d
= 7-2 = 5
The difference between their 21st terms
= a21−A21
= 7+20d-2-20d
= 7-2 = 5
The difference between any two corresponding terms of the AP’s is the same as the difference between their first terms.
- Find the value of the middle most term(s) of the AP -11, -7, -3,… ,49
Ans. Here , a = -11 , d = -7-(-11) = 4 and an =49
∴ a + (n-1)d = an
-11 + (n-1)4 = 49
⇒ (n-1)4 = 60
⇒ (n-1) = 15
⇒ n=16
As n is even number, there will be two middle terms which are (162)th and (162+1)th i.e. 8th and 9th terms.
∴ a8 =a + 7d = -11 + 7(4) = -11 + 28 = 17
a9 = a + 8d = -11 + 8(4) = -11 + 32 = 21
Hence required two middle most terms are 17 and 21.
- Determine the AP whose 5thterm is 19 and the difference of the 8th term from the 13th term is 20.
Ans. Let a be the first term and d be the common difference of given AP
a5 = a + 4d = 19 …(i)
And a13−a8=20
⇒ a + 12d – a – 7d = 20
⇒ 5d = 20 ⇒ d = 4
From(i) we have
a + 4(4) =19
a=19-16 =3
Hence required AP is 3, 7, 11, 15, … .
- Find whether 55 is a term of the AP 7, 10, 13,… or not. If not find which term it is.
Ans. Here, a=7 ,d = 13-10 = 3 and an = 55
∴ a + (n-1)d = 55
7 + (n-1)3 =55
(n-1)3 = 48
(n-1) =16
n = 17
Hence ,55 is the 17th term of the given AP
- Split 207 into three parts such that these are in AP and the product of the two smaller part is 4623.
Ans. Let the 3 parts of 207 be a-d ,a and a+d
∴ a – d + a + a + d = 207
3a = 207
a = 2073
a = 69
Also , (a-d)a =4623
⇒ (69-d)69 =4623
⇒ 69-d =67
⇒ d = 69-67 = 2
Therefore ,the 3 parts of 207 are 69-2, 69, 69+2
i.e. 67 , 69 and 71.
Related Links
NCERT Solutions for Class 10 Maths – Quadratic Equations
Polynomials with degree 2 are called quadratic polynomials. When this polynomial is equated to zero, we get a quadratic equation.
Its general form is a2 + bx + c = 0, where a, b and c are real numbers and a ≠ 0.
In many real life situations we deal with quadratic equations.
Suppose, we have to make a table of 50m2 area with its length twice as its breadth then,

Let x be the breadth of the table Therefore, its length will be 2x
Since, length × breadth = area
Therefore, x.2x = 50
So, 2x2 = 50 (quadratic equation)
x2 =25 that gives, x = 5
Thus the length of that table will be = 2x = 10m and breadth will be 5m.
EXERCISE – 4.1
Represent the following statements mathematically:
Q.1 Rahul and Nikhil together have 50 chocolates. Both of them lost 5 chocolates each and the product of number of chocolates they have now is 300.Find how many chocolates they actually had?
Sol.
Let, x be the number of chocolates Rahul had Then, the total number of chocolates Nikhil had = (50 – x) chocolates.
After loosing 5 chocolates Rahul had (x – 5) chocolates and Nikhil had (50 – x – 5) chocolates.
Now, according to the given condition:
(x – 5) (45 – x) = 300
45x – x2 – 225 + 5x=300
x2 – 50x + 525 = 0
This is the required quadratic equation.
Q2. Check whether (x – 7)2 +4 = 2x – 9 is a quadratic equation.
Sol.
On, simplifying the above equation we get:
x2 + 49 – 14x + 4 = 2x – 9
x2 – 16x + 62=0
General form of quadratic equation is ax2 + bx + c = 0
Therefore, the given equation is a quadratic equation.
Q.3 Check whether x(x-7) + 3 = (x + 6) (x – 6) is a quadratic equation.
Sol.
On, simplifying the above equation we get:
x2 – 7x + 3 = x2 – 36
7x – 33 = 0
This is not a quadratic equation because general form of a quadratic equation is ax2+bx+c=0 with a ≠ 0 and in this equation a = 0.
Q.4 Check whether (x – 2)3 = 0 is a quadratic equation.
Sol.
On, simplifying the above equation we get:
x3 – 8 – 6x2+12x = 0.
Degree of this polynomial equation is 3,
Thus, it is a cubic polynomial equation not a quadratic equation.
Q.5 Rahul’s father is 27 years older than him. 3 years from now the product of their ages will be 1224. We would like to find Rohan’s present age.
Sol.
Let the present age of Rahul be x years
Then, present age of Rahul’s father will be = (x + 27) years
Now, their ages after 3 years:
Rahul’s age = (x + 3) years
Rahul’s father age = x + 27 + 3 = (x + 30) years
According to given condition:
(x+3) (x+30) = 1224
x2 + 30x +3x +90 –1224 = 0
x2 + 33x – 1134 = 0. (Where x is the present age of Rahul in years)
This is the required quadratic equation.
Q.6 There is a rectangular park of area 860m 2. The length (meters) of the park is three more than twice its breadth (meters). Form a quadratic equation to solve this problem.
Sol.
Let, x be length (meters) and y be breadth (meter) of the rectangular plot
Now, according to given condition:-
x = 3 + 2y ……… (1)
And
x.y = 860m2…… (2)
Now, substituting equation (1) in (2) we get
(3 + 2y) y = 860
2y2 + 3y = 860
2y2 + 3y – 860 = 0 where y = breadth (in meters)
This is the required quadratic equation.
Q.7 A train is covering a distance of 540 km from one city to another city at a uniform speed. If the speed of train had been reduced by 6 km/h, then to cover the same distance the train would have taken 1 hour more. Form a quadratic equation of this situation.
Sol.
Let the train travels at the uniform speed of x km/hr.
Therefore,
Time taken to cover 540km = 540xhours
Now,
When speed is reduced by 6km/h then time taken to cover the same distance = 540x−6hours
Now, according to given condition:
540x−6−540x=1
540x – 540 ( x – 6 ) = x ( x – 6 )
x2 – 6x – 3240 = 0. Where x= speed of train in km/h
This is the required quadratic equation.
Finding roots of quadratic equation by factorization
Exercise 4.2
Q1. Find the roots of quadratic equation by factorization:
x2−(3–√+2–√)x+6–√ = 0
Sol.
x2−3–√x−2–√x+6–√=0
x(x−3–√)−2–√(x−3–√)=0
(x−3–√)(x−2–√)=0
Therefore x=2–√ or x=3–√
Q2. Find the roots of quadratic equation:
10x2−73–√x+3=0
Sol.
10x2−73–√x+3=0
x2−73√x10+310=0
x2−(3√2+3√5)x+310=0
x2−3√2x−3√5x+310=0
x(x−3√2)−3√5(x−3√2)=0
(x−3√2)(x−3√5)=0
Therefore x=3√2 or x=3√5
Q3. Find the roots of quadratic equation:
400x2 – 40x + 1 = 0
Sol.
Since, 400x2 – 40x + 1 = 0
Therefore, x2−110x+1400=0
x2−110x+1400=0
x2−(120+120)x+1400=0
x2−120x−120x+1400=0
x(x−120)−120(x−120)
(x−120)(x−120)
Therefore x=120 or x=120
Q4. Find the roots of quadratic equation:
x2– 16x + 63 = 0
Sol.
Since, x2– 16x + 63 = 0
Therefore, x2– (9+7)x + 63 = 0
x2– 9x-7x + 63 = 0
x(x – 9) – 7( x – 9 ) = 0
(x -9) ( x – 7 ) = 0
Therefore, x = 9 or x = 7
Q5. Find the roots of quadratic equation:
3–√x2+7x+23–√=0
Sol.
3–√x2+7x+23–√=0
x2+73√x+2=0
x2+63√x+13√x+2=0
x(x+63√)+13√(x+23–√)=0
(x+13√)(x+23–√)=0
Therefore x=−13√ or x=−23–√
Q.6 Product of two numbers x and y is 144 and their sum is 25. Find x and y.
Sol.
According to given condition:
x . y = 144 ……………………….. (1)
And,
x + y = 25 ……………………………. (2)
Substituting equation (2) in (1) we get:
x(25 – x) = 144
(or)
x2 – 25x + 144= 0
x2 – (16 + 9)x + 144= 0
x2 – 16x – 9x + 144= 0
x(x – 16) – 9(x – 16) = 0
Therefore, x = 16 or x = 9
So, if first number (x) is 16 then second number (y) will be (25 -16) = 9
(or)
If first number is(x) 9 then second number(y) will be (25-9) = 16.
Q7. Sum of square of two consecutive positive integers is 481, find the numbers.
Sol.
Let, 1st number be x
Therefore, second number will be (x+1)
According to the given condition:
x2 + (x + 1)2 = 481
x2 + x2 + 2x + 1 = 481
2x2 + 2x – 480 = 0
x2 + x – 240 = 0
x2 + (16 – 15) x – 240 = 0
x2 + 16x – 15x – 240 = 0
x(x + 16) – 15(x + 16) = 0
(x – 15) (x + 16) = 0
Therefore,
x= 15 (or) x= -16
Since, the positive integers can’t be -16
Hence two consecutive positive integers are 15 and 16.
Q.8 The base of a right angled triangle is 3cm more than its altitude. If the hypotenuse is 15cm. Find the length of its base and altitude.
Sol.
In right angled triangle ABC:

Let, length of altitude = x cm
Therefore base = (x + 3) cm
Now, according to the given condition:
(AB)2 + (AC)2 = (BC)2 (From Pythagoras theorem)
(x)2 + (x+3)2 = (15)2
x2 + x2 +6x + 9 = 225
2x2 + 6x – 216 = 0
x2 + 3x – 108 = 0
This is required quadratic equation, on further solving this equation:
x2 + 3x – 108 = 0
x2 + (12 – 9)x – 108 = 0
x2 + 12x – 9x – 108 = 0
x(x + 12) – 9(x + 12) = 0
(x + 12) (x – 9) = 0
Therefore, x = -12 (or) x = 9
Since length cannot be negative. Therefore, neglecting -12cm
Hence the length of altitude = 9 cm and length of the base = 12cm
Finding roots by method of completing the square
Equations like x2 – 4x – 8 = 0, cannot be solved by method of factorization because it doesn’t factor.
These types of equations can be solved by method of completing the square.
i.e x2 – 4x – 8 = 0 or x2 – 4x = 8
Our main target is to convert x2 – 4x in form (x – a)2 which is equal to x2 – 2ax + a2.
So, we will try to break the middle term in such a way, that 4x can be written in form of 2ax.
i.e 4x= 2(2.x) thus, here a = 2.
Therefore, if we will add 22 on both LHS and RHS, we get the following equation:-
x2 – 2(2x) + 22 = 8 +22
(or), (x – 2)2 = 12
(x – 2)2 = 12−−√
(x – 2)2 – (12−−√)2= 0
Since, a2−b2=(a+b)(a–b)
Therefore, (x−2−12−−√) (x−2+12−−√)
x=2+12−−√ or x=2−12−−√
This is how we find roots of a given equation by method of completing the square.
Exercise – 4.3
Q.1 Find the roots of the equation 25x2 + 20x – 13 = 0 by method of completing the square.
Sol.
(5x)2 + 20x = 13
(5x)2 + 2(5x.2)+ 22 = 13+22
(5x+2)2 – 17 = 0
Therefore (5x+2)2−(17−−√)2=0
(5x+2+17−−√) (5x+2−17−−√)=0
Therefore x=−2+17√5 or x=−2+17√5
Q.2 Find the roots of the equation 9x2 + 12x – 5 = 0 by method of completing the square.
Sol.
9x2 + 12x = 5
(3x)2 + 2(3x.2) = 5
(3x)2 + 2(3x.2) + 22 = 5 + 22
(3x+2)2 = 9
(3x+2)2 – 32 = 0
(3x + 2 + 3) (3x + 2 – 3) = 0
(3x + 5) (3x – 1) = 0
Therefore x=−53 or x=13
Q.3 Find the roots of the equation 3x2 – 16x + 5 = 0 by method of completing the square.
Sol.
To solve the above equation either divide the entire equation by 3 to make 3x2 a perfect square but it will be much easier to solve the equation if we multiply the above equation by 3 to make 3x2 a perfect square.
Therefore, 9x2 – 48x = -15
(3x)2 – 2(3x.8) =-15
(3x)2 – 2( 3x.8 ) + 82 = -15 + 82
(3x – 8)2 – 49 = 0
(3x-8)2 – 72 = 0
(3x-8+7)(3x-8-7)=0
(3x-1)(3x-15)=0
Therefore x=13 or x=5
Q.4 Find the roots of the equation 2x2 + x – 4 = 0 by method of completing the square.
Sol.
Now, for making 2x2 a perfect square, multiplying the above equation by 2
Therefore,
4x2 + 2x = 8
Now,
(2x)2+2(2x.12)=8
(2x)2+2(2x.12)+14=8+14
(2x+12)2−(334−−√)2=0
(2x+12+33√2)(2x+12−33√2)=0
Therefore x=−1+33√4 or x=−1+33√4
Consider a quadratic equation:
ax2 + bx+ c = 0 with a ≠ 0
The roots of the following quadratic equation are given by:
x=−b+b2−4ac√2a and x=−b−b2−4ac√2a
(or) x=−b±b2−4ac√2a
This is known as quadratic formulae for finding roots of quadratic equation.
Nature of roots:
The term (b2 – 4ac) is known as discriminant and it determines the nature of roots of the quadratic equation.
If b2 – 4ac > 0, then the equation will have two distant real roots.
If b2 – 4ac = 0, then the equation will have two equal real roots.
If b2 – 4ac < 0, then the equation will have unreal roots.
Exercise 4.4
Q.1 Find the roots of following equation:
x+1x=5, with x ≠ 0.
Sol.
On simplifying the above equation we get:
x2 – 5x + 1 = 0
Here, a=1, b = – 5 and c = 1.
On putting the values of a, b and c in quadratic formulae we get:
x=−(−5)+(−5)2−4(1×1)√2×1 and x=−(−5)−(−5)2−4(1×1)√2×1
x=5+25−4√2 and x=5−25−4√2
x=5+21√2 and x=5−21√2
Q.2 Find the roots of following equation:
1x−1x−3=7, where x≠0,3
Sol.
On simplifying the above equation we get:
7x2 – 21x + 3 = 0
Here, a = 7, b = -21 and c = 3
On putting the values of a, b and c in quadratic formulae we get:
x=−(−21)+(−21)2−4(7×3)√2×7 and x=−(−21)−(−21)2−4(7×3)√2×7
x=21+441−84√14 and x=21−441−84√14
x=21+357√14 and x=21−357√14
Q.3 Find the nature of roots of the following quadratic equation:-
7x2 + x + 2 = 0
Sol.
Here, a = 7, b = 1 and c=2
Since, discriminant (D) = b2 – 4ac ………….. (1)
On putting the values of a, b and c in equation (1) we get: –
D = 12 – 4(7×2)
Therefore D = -55
Since, D < 0. This equation will have no real roots.
Q.4 Find the values of k in the following quadratic equation so that they have two equal real roots 3x2 + kx + 5 = 0
Sol.
Given, 3x2 + kx + 5 = 0
Here a = 3, b = k and c = 5
Now, If b2 – 4ac = 0, then we will get two equal real roots.
Therefore,
k2 – 4×3×5 = 0
k2 = 60
Therefore for equal roots k= 215−−√
Q.5 Find the values of k in the following quadratic equation so that they have two equal real roots.
kx(x – 9) + 3 = 0
Sol.
On simplifying the given equation:
kx2 – 9kx +3 = 0
Here a = k, b = -9k and c = 3
Now, If b2 – 4ac = 0, then we will get two equal real roots.
Therefore,
(-9k)2 – 4(k×3) = 0
81k2 – 12k = 0
(or)
k(81k – 12) = 0
Since value of k cannot be 0.
Therefore for equal roots k= 1281
Q.6 Find the nature of roots of the following quadratic equation, if real roots exist, Find them.
2x2 + x – 4 = 0
Sol.
Here a = 2, b = 1 and c = – 4
Since,
D = b2 – 4ac
Therefore,
D= 12 – 4(- 4 × 2)
D= 1 + 32
Therefore, D= 33
Since, D > o. The given quadratic equation will have real roots.
On putting the values of a, b and c in the quadratic formulae we get:
x=−(1)+(1)2−4(2×−4)√2×2 and
x=−(1)−(1)2−4(2×−4)√2×2
x=−1+1+32√4 and x=−1−1+32√4
x=−1+33√4 and x=−1−33√4
Q.7 Deepak’s father is 27 years older than him. 3 years from now the product of their ages will be 1224. Find the present age of Deepak.
Sol.
Let the present age of Deepak be x years
Then, present age of Deepak’s father will be = (x + 27) years
Now, their ages after 3 years: –
Deepak’s age = (x + 3) years
Deepak’s father age = x + 27 + 3 = (x + 30) years
According to given condition,
(x+3) (x+30) = 1224
x2 + 30x +3x +90 –1224 = 0
x2 + 33x – 1134 = 0 (Where x is the present age of Deepak in years)
This is the required quadratic equation.
Here, a = 1, b = 33 and c = -1134
On putting the values of a, b and c in the quadratic formulae we get:
x=−(33)+(33)2−4(1×−1134)√2×1 and x=−(33)−(33)2−4(1×−1134)√2×1
x=−33+1089+4536√2 and x=−33−1089+4536√2
x=−33+5625√2 and x=−33−5625√2
x=−33+752 and x=−33−752
Therefore x=21 and x= -54
Since, age cannot be negative. Therefore neglecting x = -54
Thus the present age of Deepak = x = 21 years
And the present age of Deepak’s father will be = (x + 27) = 48 years
Q.8 There is a rectangular park of area 860m 2. The length (meters) of the park is three more than twice its breadth (meters). Find the length and breadth of this rectangular park.
Sol.
Let, x be length (meters) and y be breadth (meter) of the rectangular plot
Now, according to given condition:
x = 3 + 2y ……… (1)
And
x.y = 860m2…… (2)
Now, substituting equation (1) in (2) we get
(3 + 2y) y = 860
2y2 + 3y = 860
2y2 + 3y – 860 = 0 where y = breadth (in meters)
This is the required quadratic equation.
Here, a = 2, b = 3 and c = -860
On putting the values of a, b and c in the quadratic formulae we get:
y=−(3)+(3)2−4(2×−860)√2×2 and y=−(3)−(3)2−4(2×−860)√2×2
y=−3+9+6880√4 and y=−3−9+6880√4
y=−3+6889√4 and y=−3−6889√4
y=−3+834 and y=−3−834
Therefore y=20 and y= -21.5
Since, length cannot be negative. Therefore neglecting y = -21.5
Thus breadth of the rectangular park = y = 20m
And length of the rectangular park, x = (3 + 2y) = 43m
Q.9 A train is covering a distance of 540km from one city to another city at a uniform speed. If the speed of train had been reduced by 6km/h, then to cover the same distance the train would have taken 1hour more. Find the uniform speed of train.
Sol.
Let the train travels at the uniform speed of x km/hr
Therefore,
Time taken to cover 540km = 540x hrs
Now,
When speed is reduced to 6km/h then time taken to cover the same distance = 540x−6 hrs
Now, according to given condition:
540x−6−540x=1
540x – 540 ( x – 6 ) = x ( x – 6 )
x2 – 6x – 3240 = 0 Where x= speed of train in km/h
This is the required quadratic equation.
Here, a = 1, b = -6 and c = -3240
On putting the values of a, b and c in the quadratic formulae we get:
x=−(−6)+(−6)2−4(1×−3240)√2×1 and x=−(−6)−(−6)2−4(1×−3240)√2×1
x=6+36+12960√2 and x=6−36+12960√2
x=6+12996√2 and x=6−12996√2
x=6+1142 and x=6−1142
Therefore x=60 and x= -54
Since, speed cannot be negative. Therefore neglecting x = -54
Thus, the uniform speed of train = x = 60km/h.
Q.10 A chocolate factory produces a certain number of chocolates in a day. It was observed that on a particular day the cost of production of each chocolate (in rupees) was 5 more than thrice the number of chocolates produced on that day. If total cost of production on that day was 750 rupees. Find the number of chocolates produced and the cost of each chocolate.
Sol.
Let x be number of chocolates produced and y be the cost of each chocolate.
Now, According to given conditions:
y = 5 + 3x…………………. (1)
And
x.y = 750 ……………. (2)
Substituting values of equation (1) in equation (2) we get:
x(3x + 5) = 750
3x2 + 5x – 750 = 0
This is the required quadratic equation.
Here, a = 3, b = -5 and c = -750
On putting the values of a, b and c in the quadratic formulae we get:
x=−(−5)+(−5)2−4(3×−750)√2×3 and x=−(−5)−(−5)2−4(3×−750)√2×3
x=5+25+9000√6 and x=5−25+9000√6
x=5+9025√6 and x= 5−9025√6
x=5+956 and x=5−956
Therefore x=503 and x= -15
Since, number of chocolates cannot be negative. Therefore neglecting x = -15
Hence number of chocolates produced = x = 503 chocolates
And cost of each chocolate = y = (5 + 3x) = 55 Rs
Q.11 Find two consecutive even positive integers, whose product is 168.
Sol.
Let x be the first number, then second number will be (x + 2).
Now, according to the given condition:
x(x + 2) = 168
(or) x2 + 2x -168 = 0
Here a =1, b = 2 and c = -168
Now, substituting the values of a, b and c in quadratic formulae we get:
x=−(2)+(2)2−4(1×−168)√2×1 and x=−(2)−(2)2−4(1×−168)√2×1
x=−2+4+672√2 and x=−2−4+672√2
x=−2+262 and x=−2−262
Therefore x=12 and x= -14
Since x can’t be negative, therefore neglecting x = -14.
Therefore first number= x = 12 and second number = (x + 2) = 14
Q.12 Find two consecutive even positive integers, sum of whose squares is 100.
Sol.
Let x be the first number, then second number will be (x + 2)
Now, According to the given condition:
x2 + (x+2)2 = 100
x2 + x2 + 4 + 4x = 100
2x2 + 4x – 96 = 0
x2 + 2x – 48 = 0
Here, a =1, b = 2 and c = -48
Now, substituting the values of a, b and c in quadratic formulae we get:
x=−(2)+(2)2−4(1×−48)√2×1 and x=−(2)−(2)2−4(1×−48)√2×1
x=−2+4+192√2 and x=−2−4+192√2
x=−2+142 and x=−2−142
Therefore x=6 and x= -8
Since x can’t be negative, therefore neglecting x = -8
Therefore first number = x = 6 and second number = (x + 2) = 8
Q.13 It takes 105mints more for a motor boat to go 35km upstream than to return downstream at the same spot. If speed of a motor boat in still water is 21 km/h. Find speed of the stream.
Sol.
Since 60mints = 1 hour
Therefore 105mints = 10560 hours
Let the speed of stream be x km/h
Therefore, speed of boat in upstream = (21-x) km/h
And speed of boat in downstream = (21+x) km/h
Since time=distancespeed
Therefore time taken by motorboat to go upstream = 3521−xhours
And time taken by motorboat to go downstream = 3521+xhours
Now, according to the given condition:
3521−x−3521+x=10560
35(21+x−21+x212−x2)=74
2x441−x2=74×35
40x=441−x2
x2+40x−441=0
Now, from the above quadratic equation:- a = 1, b = 40 and c = -441
Substituting the values of a, b and c in quadratic formulae we get:
x=−(40)+(40)2−4(1×−441)√2×1 and x=−(40)−(40)2−4(1×−48)√2×1
x=−40+1600+1764√2 and x=−40−1600+1764√2
x=−40+3364√2 and x=−40−3364√2
x=−40+582 and x=−40−582
Therefore x =9 and x = -49
Since distance can’t be negative, therefore neglecting x = -49.
Therefore, the speed of stream = 9 km/h.
Q.14 If the difference in the parameter of two squares is 32m and sum of the areas of both squares is 544m2. Find the sides of both the squares.
Sol.
Let x be the side of 1st square and y be the side of 2nd square.
Now, according to the given conditions: –
4x – 4y = 32
Or, x – y = 8 …..………………………….. (1)
And
x2 + y2 = 544 …………………………… (2)
Now, substituting equation (1) in equation (2) we get:
(8 – y)2 + y2 = 544
y2 + 64 – 16y + y2 = 544
2y2 -16y – 480 = 0
Or, y2 – 8y – 240 = 0
Now, from the above quadratic equation:- a = 1, b = -8 and c = -240
Substituting the values of a, b and c in quadratic formulae we get:
y=−(−8)+(−8)2−4(1×−240)√2×1 and y=−(−8)−(−8)2−4(1×−240)√2×1
y=+8+64+960√2 and y=+8−64+960√2
y=8+1024√2 and y=8−1024√2
y=8+322 and y=8−322
Therefore y=20 and y= -12
Since distance can’t be negative, therefore neglecting y = -12.
Therefore, The side of 2nd square = y = 20m
And the side of 1st square = (8 + y) = 28m
Q.15 If the average speed of an express train is 15km/h more than that of a passenger train and for covering a distance of 150km between two stations A and B an express train takes 97hours less than a passenger train. Find the average speed of train A and train B.
Sol.
Let x km/h be the average speed of a passenger train.
Therefore, Average speed of an express train will be (x + 15) km/h.
Now, time taken by an express train to cover 150km = 150x+15 hours
And, time taken by a passenger train to cover the same distance = 150x hours
Now, according to the given condition:
150x – 150x+15= 97
150(x+15−x)x(x+15)=97
150×15×7=9(x2+15x)
50×5×7=x2+15x
Therefore x2+15x−1750=0
Now, from the above quadratic equation:- a = 1, b = 15 and c = -1750
Substituting the values of a, b and c in quadratic formulae we get:
x=−(15)+(15)2−4(1×−1750)√2×1 and x=−(15)−(15)2−4(1×−1750)√2×1
x=−15+225+7000√2 and x=−15−225+7000√2
x=−15+7225√2 and x=−15−7225√2
x=−15+852 and x=−15−852
Therefore x=35 and x= -50
Since speed can’t be negative, therefore neglecting x = -50.
Therefore, speed of passenger train= x = 35km\h.
Andspeed of express train= (x + 15) = 50km\h.
Q.16 Two water taps with different diameters are used to fill a particular tank, both the taps together can fill a tank in 245hours.
However, if they are operated separately, tap with larger diameter can fill the tank 4 hours early as compared to the tap with smaller diameter. Find the time in which each tap can separately fill the tank.
Sol.
Let, x hours be the time taken by the tap with smaller diameter to fill a tank separately.
Thus, in 1 hour it can fill 1x part of the tank.
Let, the time taken by the tap with larger diameter to fill a tank separately is (x-4) hours.
Thus, in 1 hour it can fill 1x−4 part of the tank.
Both the taps together can fill tank in 245hours.
Therefore in 1hour both the taps can fill 524 part of the tank.
Now, according to the given condition:
1x+1x−4=524 x−4+xx(x−4)=524 48x−965=x2−4x
5x2−68x+96=0
Now, from the above quadratic equation:- a = 5, b = -68 and c = 96
Substituting the values of a, b and c in quadratic formulae we get:
x=−(−68)+(−68)2−4(5×96)√2×5 and x= −(−68)−(−68)2−4(5×96)√2×5
x=68+4624−1920√10 and x=68−4624−1920√10
x=68+2704√10 and x=68−2704√10
x= 68+5210 and x=68−5210
Therefore x=12 and x= 1.6
When x = 1.6, It doesn’t satisfy the given conditions, therefore neglecting x = 1.6
Therefore the time taken by the tap with smaller diameter to fill the tank separately = x = 12 hours
And the time taken by the tap with larger diameter to fill the tank separately = (x – 4) = 8 hours
Q.17 There is a requirement of a rectangular park of area 575m2 and perimeter 100m. Check whether it’s possible or not? If possible then find length and breadth of that park.
Sol.
Let x be length of the park and y be breadth of the park in meters.
Now, according to the given conditions:
2(x + y) = 100
(or)
x + y = 50 ……………………… (1)
And
x.y = 575 ……………………. (2)
Now, substituting equation (1) in equation (2) we get:
(50 – y)y = 575
y2 -50y +575=0
Now, from the above quadratic equation: a = 1, b = -50 and c = 575
Since, D=b2−4(ac)
Therefore, D=32−4(1×−108)
Therefore D=441
Since, D≥0, Therefore the above quadratic equation will have real roots and hence the given situation is practically possible.
Now, from the quadratic formulae:
x=−(3)+(3)2−4(1×−108)√2×1 and x=−(3)−(3)2−4(1×−108)√2×1
x=−3+9+432√2 and x=−3−9+432√2
x=−3+441√2 and x=−3−441√2
x=−3+212 and x=−3−212
D=b2−4ac
Therefore D = (−50)2−4(1×575)
Therefore D= 200
Since, D≥0 Therefore The above quadratic will have real roots and hence it is possible to design a park with given conditions.
Now,
y=−(−50)+(−50)2−4(1×575)√2×1 and y=−(−50)−(−50)2−4(1×575)√2×1
y=50+2500−2300√2 and y=50−2500−2300√2
y=50+200√2 and y= 50−200√2
y=50+102√2 and y=50−102√2
Therefore y=25+52–√ and y=25−52–√
Now,
If breadth of park = y=25+52–√m
Then, Length of park = (50 – y) =x=25–52–√m
And If breadth of park = y=25−52–√m
Then Length of park = (50 – y) = x=25+52–√m
Q.18 There is a circular park with diameter equal to 15m having two gates one for entry and other for exit fixed at diametrically opposite ends. A plant has to be planted on the boundary of a circular park in such a way that the difference of its distance from two opposite fixed gates P and Q from boundary is 3 metres.
Check whether the above give situation is possible or not?
And if it is possible to do so, then find the distance of plant from both the gates P and Q.
Sol.

Let P be the location of the plant, A and C represents the location of gates of the park.
Now, According to the given condition:
PC – AP = 3
PC = 3 + AP
= 3 + x
Therefore, AC2 = AP2 + PC2 (Pythagoras Theorem)
152 = x2 + (x + 3)2
225 = x2+x2+9+6x
2x2 + 6x – 216 = 0
(or) x2 + 3x – 108 = 0
Now, from the above quadratic equation a = 1, b =3 and c = -108
Since,D=b2−4(ac)
Therefore D=32−4(1×−108)
Therefore D=441
Since, D≥0, Therefore the above quadratic equation will have real roots and hence the given situation is practically possible.
Now, from the quadratic formulae:
x=−(3)+(3)2−4(1×−108)√2×1 and x=−(3)−(3)2−4(1×−108)√2×1
x=−3+9+432√2 and x=−3−9+432√2
x=−3+441√2 and x=−3−441√2
x=−3+212 and x=−3−212
Therefore x=9 and x= -12
Since, the distance cannot be negative therefore neglecting x= -12
Therefore, AP = x =9m and PC = (x+3) = 12m.
Q.19 Find the value of k, if one root of the quadratic equation k2x2 – 21x + k + 9 = 0 is 1.
Sol.
From the given quadratic equation: – a= k2, b= -21 and c= (k + 9)
Let the other root of this quadratic equation be β
Therefore, sum of their roots = 1 + β = −ba =21k2
1+β=21k2
Therefore β=21−k2k2…………(1)
And product of their roots = 1 × β = ca = k+9k2
Therefore β=k+9k2…………(2)
Now, from equation (1) and equation (2) we get:
k+9k2=21−k2k2
Therefore k2+k−12=0
Now, from the above quadratic equation: a = 1, b = 1 and c = -12
Substituting the values of a, b and c in quadratic formulae we get:
k=−(1)+(1)2−4(1×−12)√2×1 and k=−(1)−(1)2−4(1×−12)√2×1
k=−1+1+48√2 and k=−1−1+48√2
k=−1+49√2 and k=−1−49√2
k=−1+72 and k=−1−72
k=3 and k= -4.
Therefore, the value of k is 3 or -4
Q.20 Determine the nature of roots of equation: 2x2 + 3x + 9 = 0
Sol.
From the given equation a = 2, b = 3 and c = 9.
Since, D = b2 – 4ac
Therefore, D = 33 – 4(9×2)= -63
Since D < 0, the given equation has no real roots.
Q.21 If the ratio of roots of quadratic equation kx2 + 18x + 15 = 0 is 1:5. Find the possible values of k.
Sol.
From the given quadratic equation: a = k, b = 18 and c = 15
Since, roots of the given quadratic equation are in ratio 1:5
Therefore 1st root will be α and 2nd root will be 5α.
Now, sum of roots = α + 5α = −ba= −18k
Therefore, 6α=−18k
Therefore α=−3k ……………….(1)
And product of roots = α × 5α = ca=15k
Therefore α2=3k………… (2)
Now, on substituting equation (1) in equation (2) we get:
(−3k)2=3k
Therefore, k = 2
Related Links
NCERT Solutions for Class 10 Maths – Pair of Linear Equations in Two Variables
Exercise1
Question 1:
Astitva tells his daughter, “Seven years ago I was seven times as old as you were then and also three years from now, I shall be three times as old as you will be.” Represent this situation algebraically and graphically.
Solution:
Let the present age of Astitva be ‘x’.
And, the present age of his daughter be ‘y’.
Seven years ago,
Age of Astitva = x-7
Age of his daughter =y-7
According to the question,
x−7=7(y−7) ⇒x−7=7y−49
⇒x−7y=−42 ………………………(i)
Three years from now,
Age of Astitva will be ‘x+3’
Age of his daughter will be ‘y+3’
According to the question,
x+3=3(y+3) ⇒x+3=3y+9
⇒x−3y=6 …………………(ii)
Subtracting equation (i) from equation (ii) we have
(x−3y)−(x−7y)=6−(−42) −3y+7y=6+42 ⇒4y=48 ⇒y=12
The algebraic equation is represented by
x−7y=−42 x−3y=6
For x−7y=−42
x=−42+7y
The solution table is
| X | -7 | 0 | 7 |
| Y | 5 | 6 | 7 |
For x−3y=6 or x=6+3y
The solution table is
| X | 6 | 4 | 0 |
| Y | 0 | -1 | -2 |
The graphical representation is-

Question 2:
A cricket team coach purchases 3 bats and 3 balls for Rs 3900. Later on, he buys 1 bat and 2 more balls of the same kind for Rs 1200. Represent the situation algebraically and geometrically.
Solution:
Let us assume that the cost of a bat be ‘Rs x’
And,the cost of a ball be ‘Rs y’
According to the question, the algebraic representation is
3x+6y=3900 x+2y=1300
For 3x+6y=3900
x=3900−6y3
The solution table is
| x | 300 | 100 | -100 |
| y | 500 | 600 | 700 |
For,
x+2y=1300 x=1300−2y
The solution table is
| x | 300 | 100 | -100 |
| y | 500 | 600 | 700 |
The graphical representation is as follows.

Question 3:
The cost of 2 kg of apples and 1 kg of grapes on a day was found to be Rs 160. After a month, the cost of 4 kg of apples and 2 kg of grapes is Rs 300. Represent the given situation algebraically and geometrically.
Solution:
Let the cost of 1 kg of apples be ‘Rs x’
And, cost of 1 kg of grapes be ‘Rs y’
According to the question, the algebraic representation is
2x+y=160 4x+2y=300
For, 2x+y=160
y=160−2x
The solution table is
| X | 50 | 60 | 70 |
| Y | 60 | 40 | 20 |
For 4x+2y=300,
y=300−4x2
The solution table is
| x | 70 | 80 | 75 |
| y | 10 | -10 | 0 |
The graphical representation is as follows.

Exercise-2
Question 1:
Find the graphical solution for the given problems.
(a).In Class 10th, 10 students participated in a Maths test. If there are 4 more girls than the total number of boys, find how many girls and boys participated in the test.
(b)The total cost of 5 erasers and 7 chocolates is Rs.50, but the total cost of 7 erasers and 5 chocolates is Rs. 46. Now, calculate the cost of one eraser and the cost of one chocolate.
Solution:
(a)Let there are x number of girls and y number of boys. As per the given question, it is represented as follows.
x+y=10x−y=4Nowx+y=10,x=10−y
| X | 5 | 4 | 6 |
| Y | 5 | 6 | 4 |
Forx−y=4,x=4+y
| X | 4 | 5 | 3 |
| Y | 0 | 1 | -1 |
Hence, the table is represented in graphical form as follows.

From the figure, it is seen that the given lines cross each other at point (7, 3). Therefore, there are 7 girls and 3 boys in the class .
(b)Let 1 pencil costs Rs.x and 1 pen costs Rs.y. According to the question, the algebraic representation is
5x+7y=507x+5y=46For5x+7y=50, x=50−7y5
| x | 3 | 10 | -4 |
| y | 5 | 0 | 10 |
7x+5y=46
| X | 8 | 3 | -2 |
| Y | -2 | 5 | 12 |
Hence, the graphic representation is as follows.

From the figure, it is seen that the given lines cross each other at point (3, 5).
So, the cost of a eraser is 3/- and cost of a chocolate is 5/-.
Question 2:
Comparing the given ratios, a1a2,b1b2andc1c2,Figure out whether the lines are parallel or coincident:
(a) 5x−4y+8=0
7x+6y−9=0
(b) 9x+3y+12=018x+6y+24=0
(c) 6x−3y+10=02x−y+9=0
Solution:
(a) 5x−4y+8=0
7x+6y−9=0
Comparing these equations with a1x+b1y+c1=0
and a2x+b2y+c2=0
We get,
a1=5,b1=−4,c1=8a2=7,b2=6,c2=−9 a1a2=57 b1b2=−46=−23
Since a1a2≠b1b2
So, the pairs of equations given in the question have a unique solution and the lines cross each other at exactly one point.
(b) 9x+3y+12=018x+6y+24=0
Comparing these equations with a1x+b1y+c1=0
and a2x+b2y+c2=0
We get,
a1=9,b1=3,c1=12a2=18,b2=6,c2=−24 a1a2=918=12 b1b2=36=12 c1c2=1224=12
Since a1a2=b1b2=c1c2
So, the pairs of equations given in the question have infinite possible solutions and the lines are coincident.
(c) 6x−3y+10=02x−y+9=0
Solution: Comparing these equations with a1x+b1y+c1=0
and a2x+b2y+c2=0
We get,
a1=6,b1=−3,c1=10a2=2,b2=−1,c2=9 a1a2=62=31 b1b2=−3−1=31 c1c2=109
Since a1a2=b1b2≠c1c2
So, the pairs of equations given in the question are parallel to each other and the lines never intersect each other at any point and there is no possible solution for the given pair of equations.
Question 3:
Compare the given ratios, a1a2,b1b2andc1c2 find out whether the following pair of linear equations are consistent, or inconsistent.
(a) 3x+2y=5;2x−3y=7
(b) 2x−3y=8;4x−6y=9
(c) 32x+53y=7;9x−10y=14
Solution:
(a) 3x+2y=5;2x−3y=7
a1a2=32 b1b2=−23 c1c2=57
Since a1a2≠b1b2
So, the given equations intersect each other at one point and they have only one possible solution. The equations are consistent.
(b) 2x−3y=8;4x−6y=9
a1a2=24 b1b2=−3−6 c1c2=89
Since a1a2=b1b2≠c1c2
So, the equations are parallel to each other and they have no possible solution. So, the equations are inconsistent.
(c) 32x+53y=7;9x−10y=14
a1a2=329=16 b1b2= frac53−10=−16 c1c2=714
Since a1a2≠b1b2
So, the equations are intersecting each other at one point and they have only one possible solution. So, the equations are consistent.
Question 4:
Find whether the following pairs of linear equations are consistent or inconsistent. If consistent, find the solution graphically:
(a)x + y = 5, 2x + 2y = 10
(b)2x + y – 6 = 0,4x – 2y – 4 = 0
Solution:
(a)x + y = 5, 2x + 2y = 10
a1a2=12 b1b2=12 c1c2=510=12
Since a1a2=b1b2=c1c2
∴ the equations are coincident and they have infinite number of possible solutions.
So, the equations are consistent.
x + y = 5, 2x + 2y = 10
| x | 4 | 3 | 2 |
| y | 1 | 2 | 3 |
And, 2x + 2y = 10
x=10−2y2
| x | 4 | 3 | 2 |
| y | 1 | 2 | 3 |
So, the equations are represented as follows:

From the figure, it is seen that the lines are overlapping each other.
Therefore, the equations have infinite possible solutions.
Question 5:
Consider the two equations x − y + 1 = 0 and 3x + 2y − 12 = 0. Draw a graph for both. Find out the coordinates of the vertices of the triangle formed by these lines and the x-axis, and shade the triangular region.
Solution:
x−y+1=0
Or
x=y−1
| x | 0 | 1 | 2 |
| y | 1 | 2 | 3 |
3x+2y−12=0x=12−2y3
Hence, the graphic representation is as follows.

From the figure, it can be observed that these lines are intersecting each other at point (2, 3) and x-axis at (−1, 0) and (4, 0). Therefore, the vertices of the triangle are (2, 3), (−1, 0), and (4, 0).
Exercise 3
Question 1:
Using substitution method, solve the given pair of linear equations.
- i) x – y = 3
x3+y2=6
- ii) a + b = 1
a – b = 4
iii) 0.2a + 0.3b = 1.3
0.4a+0.5b = 2.3
- iv) 3a – b = 3
9a – 3b = 9
- v) 3x2–5y3=−2
x3+y2=136
- vi) 2–√x+3–√y=0
3–√x–8–√y=0
Solution:
i) x – y = 3- – – – – – – – – – – (I)
x3+y2=6– – – – – – – – (II)
From (I), we get
x = y + 3- – – – – — – – – (III)
Substituting the value of x in equation (II), we get
y+33+y2=6
2y + 6 + 3y = 36
5y = 30
y = 6- – – – – – – – – (IV)
Substituting the value of y in equation (III), we get x = 9
∴ x = 9, y = 6
ii) a + b = 14- – – – – – – – – (I)
a – b = 4 — – – – – – – – – (II)
From (I), we get a = 14 – b- – – – – – – – – – (III)
Substituting the value of a in equation (II), we obtain
(14 – b) – b = 4
14 – 2b = 4
10 = 2b
b = 5- – – – – – – – – (IV)
Substituting (IV) in (III), we get
a = 14 – b
= 14 – 5
= 9
∴ a = 9, b = 5
iii) 0.2a + 0.3b = 1.3- – – – – – – (I)
0.4a + 0.5b = 2.3- – – – – – – – (II)
From equation (I), we get
a=1.3−0.3b0.2 – – – – – – – – (III)
Substituting the value of a in equation (II), we get
0.4(1.3−0.3b0.2)+0.5b=2.3
2.6 – 0.6b + 0.5b = 2.3
2.6 – 2.3 = 0.1b
0.3 = 0.1b
b = 3- – – – – – – – (IV)
Substituting the value of b in equation (III), we get
a=1.3−0.3∗30.2 =1.3−0.90.2=0.40.2=2
∴ a = 2, b = 3
iv) 3a – b = 3- – – – – – – – (I)
9a – 3b = 9 – – – – – – – – (II)
From (I), we get b = 3a – 3 – – – – – – – – (III)
Substituting the value of b in equation (II), we get
9a – 3(3a – 3) = 9
9a – 9a + 9 = 9
9 = 9
This is always true.
The given pair of equations has infinite solutions and the relation between these variables can be given by b = 3a – 3
Therefore, one of its possible solutions is a = 1, b = 0.
v) 3x2–5y3=−2 – – – – – – – – (I)
x3+y2=136 – – – – – – – – (II)
From equation (I), we get
9x – 10y = -12
x=−12+10y9 – – – – – – – – (III)
Substituting the value of x in equation (II), we get
−12+10y93+y2=136 −12+10y27+y2=136 −24+20y+27y54=136
47y = 117 + 24
47y = 141
y = 3 – – – – – – – – (IV)
Substituting the value of y in equation (III), we get
x=−12+10∗39=189=2
Hence, x = 2, y = 3
vi) 2–√x+3–√y=0 – – – – – – – – (I)
3–√x–8–√y=0 – – – – – – – – (II)
From equation (I), we get
x=−3√y2√ – – – – – – – – (III)
Substituting the value of x in equation (II), we get
3–√(−3√y2√)−8–√y=0 −3y2√−22–√y=0 y(−32√−22–√)=0
y = 0- – – – – – – – (IV)
Substituting the value of y in equation (III), we get
x = 0
∴ x = 0, y = 0
Question 2:
Solve 2a + 3b = 11 and 2a – 4b = -24 and calculate the value of m in b = ma + 3.
Solution:
2a + 3b = 11 (I)
2a – 4b = -24 (II)
From equation (II), we get
a=11−3b2 (III)
Substituting the value of a in equation (II), we get
2(11−3b2)−4b=−24
11 – 3b – 4b = -24
-7b = -35
b = 5 (IV)
Putting the value of b in equation (III), we get
a=11−3∗52=−42=−2
Hence, a = -2, b = 5
Also,
b = ma + 3
5 = -2m +3
-2m = 2
m = -1
Question 3:
Using substitution method, find the solution by forming the pair of linear equations for the given problems.
i) The coach of a cricket team buys 7 bats and 6 balls. It cost her Rs 3800. Later, she buys 3 bats and 5 balls. It cost her Rs 1750. Find the cost of single bat and single
Solution:
Let the cost a bat be x and cost of a ball be y.
According to the question,
7x + 6y = 3800 …………….. (I)
3x + 5y = 1750 ……………… (II)
From (I), we get
y=3800−7x6 ………………. (III)
Substituting (III) in (II). we get
3x+5(3800−7x6)=1750 3x+95003−35x6=1750 3x−35x6=1750−95003 18x−35x6=5250−95003 −17x6=−42503
-17x = -8500
x = 500 ……………….. (IV)
Substituting the value of x in (III), we get
y=3800−7∗5006=3006=50
Hence, the cost of a bat is Rs 500 and cost of a ball is Rs 50.
ii) The difference between two numbers is 26 and one number is three times the older. Find the two numbers.
Solution:
Let the two numbers be x and y respectively, such that y > x.
According to the question,
y = 3x ……………… (1)
y – x = 26 …………..(2)
Substituting the value of (1) into (2), we get
3x – x = 26
x = 13 ……………. (3)
Substituting (3) in (1), we get y = 39
Hence, the numbers are 13 and 39.
iii) Five years hence, the age of Rahul will be three times that of his son. Five years ago, Rahul’s age was seven times that of his son. Calculate their present ages.
Solution:
Let the age of Rahul and his son be x and y respectively.
According to the question,
(x + 5) = 3(y + 5)
x – 3y = 10 ………………… (1)
(x – 5) = 7(y – 5)
x – 7y = -30 ……………….. (2)
From (1), we get x = 3y + 10 ……………………. (3)
Substituting the value of x in (2), we get
3y + 10 – 7y = -30
-4y = -40
y = 10 ………………… (4)
Substituting the value of y in (3), we get
x = 3 x 10 + 10
= 40
Hence, the present age of Rahul and his son is 40 years and 10 years respectively.
iv) If 2 is added to both the numerator and the denominator a fraction becomes 8/11. If 3 is added to both the numerator and the denominator it becomes 5/6. Find the fraction.
Solution:
Let the fraction be x/y.
According to the question,
x+2y+2=911
11x + 22 = 9y + 18
11x – 9y = -4 …………….. (1)
x+3y+3=56
6x + 18 = 5y +15
6x – 5y = -3 ………………. (2)
From (1), we get x=−4+9y11 …………….. (3)
Substituting the value of x in (2), we get
6(−4+9y11)−5y=−3
-24 + 54y – 55y = -33
-y = -9
y = 9 ………………… (4)
Substituting the value of y in (3), we get
x=−4+8111=7
Hence the fraction is 7/9.
v) The larger of two supplementary angles exceeds the smaller by 18o. Find the two angles.
Solution:
Let the larger angle by xo and smaller angle be bo.
We know that the sum of two supplementary pair of angles is always 180o.
According to the question,
x + y = 180o……………. (1)
x – y = 18o ……………..(2)
From (1), we get x = 180o – y …………. (3)
Substituting (3) in (2), we get
180o – y – y =18o
162o = 2y
y = 81o ………….. (4)
Using the value of y in (3), we get
x = 180o – 81o
= 99o
Hence, the angles are 99o and 81o.
vi) A taxi charge consists of a fixed charge + charge for the distance covered. For a distance of 10 km and 15 km, the charge paid is Rs 105 and Rs 155 respectively. Calculate the fixed charge and the charge per km. Also, calculate travel cost for 25 km.
Solution:
Let the fixed charge be Rs x and per km charge be Rs y.
According to the question,
x + 10y = 105 …………….. (1)
x + 15y = 155 …………….. (2)
From (1), we get x = 105 – 10y ………………. (3)
Substituting the value of x in (2), we get
105 – 10y + 15y = 155
5y = 50
y = 10 …………….. (4)
Putting the value of y in (3), we get
x = 105 – 10 * 10 = 5
Hence, fixed charge is Rs 5 and per km charge = Rs 10
Charge for 25 km = x + 25y = 5 + 250 = Rs 255
Exercise 4
Question 1:
Using the method of elimination and method of substitution, solve the given liner equations.
(1) a + b = 5 and 2a – 3b = 4
(2) 3a + 4b = 10 and 2a – 2b = 2
(3) 3a = 5b – 4 = 0 and 9a = 2b + 7
(4) a2+2b3=−1 and a−b3=3
Solution:
(1) By the method of elimination.
A + B = 5 – – – – – – – – (i)
2A – 3B = 4 – – – – – – – – (ii)
When the equation (i) and is multiplied by (ii), we get
2A + 2B = 10 – – – – – – – – (iii)
When the equation (ii) is subtracted from (iii) we get,
5B = 6
B = 65 – – – – – – – (iv)
Substituting the values obtained in (i) we get,
A=5−65=195 ∴A=195,B=65
By the method of substitution:
From the equation (i), we get:
A = 5 – B – – – – – – – (v)
When the value is put in equation (ii) we get,
2(5 – B) – 3B = 4
-5B = -6
B = 65
When the values are substituted in equation (v), we get:
A=5−65=195 ∴A=195,B=65
(2) By the method of elimination:
3A + 4B = 10 – – – – – – -(i)
2A – 2B = 2 – – – – — – – (ii)
When the equation (i) and (ii) is multiplied by 2, we get:
4A – 4B = 4 – – – – – – – (iii)
When the Equation (i) and (iii) are added, we get:
7A = 14
A=2 – – – – – – – – – (iv)
Substituting equation (iv) in (i) we get,
6 + 4B = 10
4B = 4
B = 1
Hence, A = 2 and B = 1
By the method of Substitution
From equation (ii) we get,
A = 1 + B – – – – – – – – (v)
Substituting equation (v) in equation (i) we get,
3(1 + B) + 4B = 10
7B = 7
B= 1
When B= 1 is substituted in equation (v) we get,
A = 1 + 1 = 2
Therefore, A =2 and B = 1
(3) By the method of elimination:
3A – 5B – 4 = 0 – – – – – – – (i)
9A = 2B + 7
9A – 2B – 7 = 0 – – – – – – – (ii)
When the equation (i) and (iii) is multiplied we get,
9A – 15B – 12 = 0 – – – – – (iii)
When the equation (iii) is subtracted from equation (ii) we get,
13B = -5
B=−513 – – – – – – – – (iv)
When equation (iv) is substituted in equation (i) we get,
3A+2513−4=0 3A=2713 A=913 ∴A=913,B=−513
By the method of Substitution:
From the equation (i) we get,
A=5B+43 – – – – – – – – (v)
Putting the value (v) in equation (ii) we get,
9(5B+43)−2B−7=0
13B = -5
B=−513
Substituting this value in equation (v) we get,
A=5(−513)+43 A=913 ∴A=913,B=−513
(4) By the method of Elimination
A2=2B3=−1
3A + 4B = -6 – – – – – – – (i)
A−B3=3
3A – B = 9 – – – – – – – (ii)
When the equation (ii) is Subtracted from equation (i) we get,
5B = -15
B = 3 – – – – – – (iii)
When the equation (iii) is substituted in (i) we get,
3A – 12 = -6
3A = 6
A = 2
Hence, A = 2 , B = -3
By the method of Substitution:
From the equation (ii) we get,
A=B+93 – – – – – – – – – (v)
Putting the value obtained from equation (v) in equation (i) we get,
3(B+93)+4B=−6
5B = -15
B= -3
When B= -3 is substituted in equation (v) we get,
A=−3+93=2
Therefore, A = 2 and B = -3
Question 2:
Find the solutions for the given pair of linear equation by the method of elimination for the following questions:
(1) If 1 is added in the numerator and 1 subtracted from that of the denominator, a fraction is then reduced to 1. It becomes ½ if 1 is added only to the denominator. What is the fraction?
(2) Reuben was thrice as old as Alex, 5 years back. And Reuben will be twice as old as Alex, 10 years from now. What are the ages of Reuben and Alex?
(3) The sum of digits of a two digit number is 9. Also when this number is multiplied nine times, it is two times the number when obtained by reversing the order of the digits. Find the number.
(4) Manna went to the bank to withdraw a sum of Rs 2000. She had asked the cashier to give her Rs 50 and Rs 100 notes only. Manna got 25 notes in total. Find how many Rs 50 notes and Rs 100 notes she had received.
(5) A lending library has a fixed charge for the first three days and an additional charge for each day thereafter. Sara paid a sum of Rs 27 for a book to keep it for seven days, while Rosy paid a sum of Rs 21 for the book that she had kept for five days. Find the fixed charge and the charge that will cost for each extra day.
Solution:
(1) Let the fraction be a/b
According to that of the given information,
A+1B−1=1 => A – B = -2 – – – – – – – – – – – (i)
AB+1=12 => 2A – B = 1 – – – – – – – – (ii)
When equation (i) is Subtracted from equation (ii) we get A = 3 – – – – – – (iii)
When A = 3 is substituted in equation (i) we get,
3 – B = -2
-B = -5
B = 5
Hence, the fraction is 3/5
(2) Let the present age of Reuben = a
And the present age of Alex = b
According to the information that is given,
(A – 5 ) = 3 ( B – 5 )
A – 3B = -10 – – – – – – – – – (i)
(A + 10) = 2(B + 10)
A – 2B = 10 – – – – – – – – – – – (ii)
When the equation (i) is subtracted from equation (ii) we get,
B = 20 – – – – – – – – – (iii)
Substituting B = 20 in equation (i), we get:
A – 60 = -10
A = 50
Hence, the present age of Reuben is 50 yrs
And the present age of Alex is 20 yrs
(3) Let the unit digit and tens digit of a number be A and B respectively.
Then, Number (n) = 10B + A
N after reversing the digits = 10A + B
According to the given information, A + B = 9 – – – – – – – – – (i)
9(10B + A) = 2(10A + B)
88 B – 11 A = 0
-A + 8B = 0 – – – – – – – – (ii)
Adding the equations (i) and (ii) we get,
9B = 9B
= 1 (3)
Substituting this value in the equation (i) we get A= 8
Hence the number (N) is 10B + A = 10 x 1 +8 = 18
(4) Let the number of Rs 50 notes be A and the number of Rs 100 notes be B
According to the given information,
A + B = 25 – – – – – – – – – – – (i)
50A + 100B = 2000 – – – – – – – (ii)
When equation (i) is multiplied with (ii) we get,
50A + 50B = 1250 – – – – – – – – – (iii)
Subtracting the equation (iii) from the equation (ii) we get,
50B = 750
B = 15
Substituting in the equation (i) we get,
A = 10
Hence, Manna has 10 notes of Rs 50 and 15 notes of Rs 100.
(5) Let the fixed charge for the first three days be Rs A and the charge for each day extra be Rs B.
According to the information given,
A + 4B = 27 – – – – – (i)
A + 2B = 21 – – – – – – (ii)
When equation (ii) is subtracted from equation (i) we get,
2B = 6
B = 3 – – – – – – (iii)
Substituting B = 3 in equation (i) we get,
A + 12 = 27
A = 15
Hence, the fixed charge is Rs 15
And the Charge per day is Rs 3
Exercise-5
Question 1:
From the given pairs of linear equations find the ones with unique solution, no solution or infinitely many solutions. If there is a unique solution, find it by using cross multiplication method.
(i) x-3y-3 =0 and 3x-9y-2=0 (ii) 2x+y =5 and 3x +2y =8
(iii)6x – 10y =40 and 3x-5y = 20 (iv)x -3y – 7 =0 and 3x -3y -15=0
Solution:
(i) 3x – 9y -2 =0
x – 3y – 3 =0
a1/a1=1/3 , b1/b2 = -3/-9 =1/3, c1/c2=-3/-2 = 3/2
a1a2=b1b2≠c1c2
Since, the given set of lines are parallel to each other they will not intersect each other and therefore there is no solution for these equation.
(ii) 2x + y = 5
3x +2y = 8
a1a2=23,b1b2=12,c1c2=−5−8 a1a2≠b1b2
Since they intersect at a unique point these equations will have a unique solution by cross multiplication method:
xb1c2−c1b2=yc1a2−c2a1=1a1b2−b1a2 x−8+10=y−15+16=14−3
x/ 2 = y/1 = 1
x =2 , y =1.
(iii) 6x -10y = 40
3x – 5y = 20
a1 / a2 = 3/6 = 1/2 , b1/b2 = -5/-10 =1/2, c1/c2 =-20/-40 = ½
a1/a2 = b1/b2 = c1/c2
Since the given sets of lines are overlapping each other there will be infinite number of solutions for this pair of equation.
(iv) 3x – 3y – 15 =0
x – 3y – 7 = 0
a1/a2 = 1/3, b1/b2 = 1 , c1/c2 = -7/-15
a1a2≠b1b2
Since this pair of lines are intersecting each other at a unique point, there will be a unique solution.
By cross multiplication,
x/(45-21) = y/(-21+15) = 1/(-3+9))
x/24 = y/-6 =1/6
x/24 = 1/6 and y/-6 = 1/6
∴ x = 4 and y =1.
Question 2:
(i) Find the values of a and b at which the following pair of linear equations will have infinite solution.
3y + 2x = 7
(a+b)y +(a-b)x = 3a + b -2
(ii) Find the value of k at which the following set of linear equations won’t have a solution.
3x + y =1
(2k – 1)x + (k-1)y = 2k + 1
Solution:
(i) 3y + 2x -7 =0
(a + b) y + (a-b)y – (3a + b -2) = 0
a1/a2=2/a-b , b1/b2=3/a+b , c1/c2=-7/-(3a + b -2)
For infinitely many solutions,
a1/a2 = b1/b2 = c1/c2
2/a-b = 7/3a + b – 2
6a + 2b – 4 = 7a – 7b
a – 9b = -4 ……(i)
2/a-b = 3/a+b
2a + 2b = 3a – 3b
a -5b = 0 …….(ii)
Subtracting (i) from (ii), we get
4b = 4
b =1
Substituting this eqn in (ii), we get
a -5 x 1= 0
a = 5
Thus at a = 5 and b = 1 the given equations will have infinite solutions.
(ii) 3x + y -1 = 0
(2k -1)x + (k-1)y – 2k -1 = 0
a1/a2 = 3/2k -1 , b1/b2=1/k-1 ,c1/c2 = -1/-2k -1 = 1/ 2k +1
For no solutions
a1/a2 = b1/b2 ≠ c1/c2
3/2k-1 = 1/k -1 ≠ 1/2k +1
3/2k – 1 = 1/k -1
3k -3 = 2k -1
k =2
Therefore, for k = 2 the given pair of linear equations will have no solution.
Question 3:
Solve the given pair of linear equations using cross multiplication method and substitution method:
3x + 2y = 4
8x + 5y =9
Solution:
- 8x + 5y = 9 ….(1)
3x + 2y = 4 ….(2)
From equation (2) we get
x = 4 – 2y / 3 …. (3)
Using this value in equation 1 we get
8(4-2y)/3 + 5y = 9
32 – 16y +15y = 27
-y = -5
y = 5 ……(4)
Using this value in equation (2), we get
3x + 10 = 4
x = -2
Thus , x = -2 and y = 5.
Now, Using Cross Multiplication method:
8x +5y – 9 = 0
3x + 2y – 4 = 0
x/(-20+18) = y/(-27 + 32 ) = 1/(16-15)
-x/2 = y/5 =1/1
Thus , x = -2 and y =5.
Question 4:
For the problems given below, form a pair of linear equations and also solve them (if a solution exists).
(i) When 1 is subtracted from the numerator of a fraction it becomes 1/3 and it becomes 1/4 when 8 is added to the denominator. Find the fraction.
(ii) Gah and Hag are 100kms apart on a highway. A car starts from Gah and another from Hag at the same time. If the cars move towards each other, they meet in one hour and if they move in the same direction, they meet after 5 hours. Find the speed of the two cars.
(iii) Monthly hostel charges are fixed, except for the mess charge which is included in it. Dom a eats for 20 days in the mess and pays Rs1000 as her monthly hostel charge. Nimi eats for 26 days and pays Rs.1180 as hostel charges. What is the fixed charge and the cost of food per day?
(iv) Lama scored 40 in an exam, acquiring 3 marks higher for each right answer and losing 1 mark for every wrong answer. If 4 marks had been awarded for every correct answer and 2 marks reduced for every wrong answer, Lama would have scored 50 marks. Find the number of questions in the exam.
Solution:
(i) let the fraction be x/y
So, according to question :
(x-1)/y = 1/3 => 3x – y = 3…..(1)
x/(y + 8) = 1/4 => 4x –y =8 ….(2)
Subtracting equation (1) from (2) , we get
x = 5 ……(3)
Using this value in equation (2) we get
4×5 – y = 8
y= 12.
Therefore, the fraction is 5/12.
(ii)Let the speed of the first and second cars be x km/h and y km/h respectively.
Respective speed of the two cars when they are moving in the same direction = (x -y) km/h
Respective speed of the two cars when they are headed towards each other = (x + y)km/h
According to the question;
5(x-y) =100
x – y =20 ….. (1)
Also,
1(x +y )=100 …..(2)
Adding equations (1) and (2) we get
2x =120
x = 60 km/h ……(3)
Using this in equation (1) we get
60-20= y
y= 40 km/h.
(iii) let x be the fixed charge and y be the charge of food per day.
According to the question
x + 20y = 1000…. (i)
x + 26y = 1180….(ii)
Subtracting (i) from (ii) we get
6y=180
y= Rs.30
Using this value in equation (ii) we get
x = 1180 -26 x 30
= Rs.400.
(iv) Let x be the number of correct numbers and y be the number of incorrect answers.
According to the question,
3x – y = 40 …..(i)
4x – 2y = 50
2x-y = 25….(ii)
Subtracting equation (ii) from (i), we get
x=15…………(iii)
Using this in equation (i) we get
3(15) – 40 =y
y= 5
Therefore, the number of correct answers = 15
the number of incorrect answers= 5
the number of questions =20
EXERCISE – 6
Solve the following pairs of equations by reducing them to a pair of linear equations:
Question 1:
12a+13b=2
13a+12b=136
Solution:
Let us assume 1a=mand1b=n, then the equation will change as follows.
m2+n3=2⇒3m+2n−12=0 …….(1)
m3+n2=136⇒2m+3n−13=0 …….(2)
Now, Using cross-multiplication method, we get
m−26−(−36)=n−24−(−39)=19−4 m10=q15=15 m10=15andq15=15
m = 2 and n = 3
1a=2and1b=3 a=12andb=13
Question 2:
4a+3b=14
4a−4b=23
Solution:
Putting 1a=m in the given equation we get,
So, 4m + 3b = 14 => 4m + 3b – 14 = 0 ………..(1)
3m – 4b = 23 => 3m – 4b – 23 = 0 ………..(2)
By cross-multiplication, we get,
m−69−56=b−42−(−92)=1−16−9 m−125=b50=−125 m−125=−125andb50=−125
m = 5 and b = -2
m = 1a = 5
a = 15
b = -2
Question 3:
7a−2bab=5
7b−2a=5 …..(i)
8a+7bab=15
8b+7a=15 …..(ii)
Solution:
Substituting 1a=m in the given equation we get,
– 2m + 7n = 5 => -2 + 7n – 5 = 0 ……..(iii)
7m + 8n = 15 => 7m + 8n – 15 = 0 ……(iv)
By cross-multiplication method, we get,
m−105−(−40)=n−35−30=1−16−49 m−65=n−65=1−65 m−65=1−65andb−65=1−65
m = 1 and n = 1
m = 1a = 1 n = 1a = 1
a = 1 b = 1
Question 4:
10a+b+2a−b=4
15a+b−5a−b=−2
Solution:
Substituting 1a+b=m and 1a−b=n in the given equations, we get,
10m + 2n = 4 => 10m + 2n – 4 = 0 …..(i)
15m – 5n = -2 => 15m – 5n + 2 = 0 …..(ii)
Using cross-multiplication method, we get,
m4−20=n−60−(−20)=1−50−30 m−16=n−80=1−80 m−16=1−80andb−80=1−80
m = 15 and n = 1
m = 1a+b=15 and n = 1a−b=1
a + b = 5 …..(iii)
and a – b = 1 …..(iv)
Adding equation (iii) and (iv), we get
2a = 6 => a = 3 …….(v)
Putting the value of a = 3 in equation (3), we get
b = 2
Hence, a = 3 and b = 2
Question 5:
2a√+3b√=2
4a√−9b√=−1
Solution:
Substituting 1a√=m and 1b√=n in the given equations, we get
2m + 3n = 2 …….(i)
4m – 9n = -1 ……(ii)
Multiplying equation (i) by 3, we get
6m + 9n = 6 ………..(iii)
Adding equation (ii) and (iii), we get
10m = 5
m = 12 ……(iv)
Now by putting the value of ‘m’ in equation (i), we get
2×12+2n=2
3n = 1
n = 13
m = 1a√=12
a−−√=2
a = 4
n = 1b√=13
b√=3
b = 9
Hence, a = 4 and b = 9
Question 6:
5a−1+1b−2=2
6a−1−3b−2=1
Solution:
Substituting 1a−1=m and 1b−2=n in the given equations, we get,
5m + n = 2 ……(i)
6m – 3n = 1 …..(ii)
Multiplying equation (i) by 3, we get
15m + 3n = 6 ……..(iii)
Adding (ii) and (iii) we get
21m = 7
m = 13
Putting this value in equation (i), we get
5×13+n=2 n=2−53=13 m=1a−1=13⇒a−1=3⇒a=4 n=1b−2=13⇒b−2=3⇒b=5
Hence, a = 4 and b = 5
Question 7:
6a + 3b = 6ab
=> 6b+3a=6 …..(i)
2a + 4b = 5ab
=> 2b+4a=5 …….(ii)
Substituting 1a=m and 1b=n
3m + 6n – 6 = 0
4m + 2n – 5 = 0
By cross-multiplication method, we get
m−30−(−12)=n−24−(−15)=16−24 m−18=n−9=1−18 m−18=1−18andn−9=1−18
m = 1 and n = 1/2
m = 1/a = 1 and n = 1/b = 1/2
a = 1 b = 2
Hence, a = 1 and b = 2
Question 8:
13a+b+13a−b=34
12(3a+b)−12(3a−b)=−18
Solution:
substituting 13a+b=mand13a−b=n in the given equations, we get
m + n = 3/4 ……(1)
m/2 – n/2 = -1/8
m – n = -1/4 ……(2)
Adding (1) and (2), we get
2m = 3/4 – 1/4
2m = 1/2
Putting in (2), we get
1/4 – n = -1/4
n = 1/4 + 1/4 = 1/2
m = 13a+b=14
3a + b = 4 …….(3)
n = 13a−b=12
3a – b = 2 ………(4)
Adding equations (3) and (4), we get
6a = 6
a = 1 ……..(5)
Putting in (3), we get
3(1) + b = 4
b = 1
Hence, a = 1 and b = 1
Exercise 7
Question 1:
The ages of Reuben and Alex are at a difference of 3 yrs. Reuben’s father Thomas is twice as old as Reuben and Alex is twice as old as his sister Ann. The ages of Ann and Thomas are at a difference of 30 yrs. What is the present age of Reuben and Alex?
Solution:
The age difference between Reuben and Alex is 3 yrs.
Either Alex is 3 yrs older than that of Reuben or Reuben is 3 yrs older than Alex. From both the cases we find out that Reuben’s father’s age is 30 yrs more than that of Ann’s age.
Let the ages of Reuben and Alex be A and B respectively.
Therefore, the age of Thomas = 2 x A = 2A yrs.
And the age of Alex’s sister Ann B/2 yrs
By using the information that is given,
Case (i)
When Reuben is older than that of Alex by 3 yrs then A – B = 3 – – – – – – – – (1)
2A−B2=30
4A – B = 60 – – – – – – – – – – – (2)
By subtracting the equations (1) and (2) we get,
3A = 60 – 3 = 57
A=573=19
Therefore, the age of Reuben = 19 yrs
And the age of Alex is 19 – 3 = 16 yrs.
Case (ii)
When Alex is older than Reuben,
B – A = 3 – – – – – – – – – (1)
2A−Y2=30
4A – B = 60 – – – – – – – – – (2)
Adding the equation (1) and (2) we get,
3A = 63
A = 21
Therefore, the age of Reuben is 21 yrs
And the age of Alex is 21 + 3 = 24 yrs.
Question 2:
Sangam says, “Give me Rs100, bro! I’ll become two times richer than you” Reuben replies, “If you give me Rs 10, I’ll become six times richer than you” What is the capital amount of Sangam and Reuben.
[Hint: A + 100 = 2 ( B – 100) , B + 10 =6(A- 10)]
Solution:
Let Sangam have Rs A with him and Reuben have Rs B with him.
Using the information that is given we get,
A + 100 = 2(B – 100) A + 100 = 2B – 200
Or A – 2B = -300 – – – – – – – (1)
And
6(A – 10) = ( B + 10 )
Or 6A – 60 = B + 10
Or 6A – B = 70 – – – – – – (2)
When equation (2) is multiplied by 2 we get,
12A – 2B = 140 – – – – – – – (3)
When equation (1) is subtracted from equation (3) we get,
11A = 140 + 300
11A = 440A
=> 40
Using A =40 in equation (1) we get,
40 – 2B = -300
40 + 300 = 2B
2B = 340
B = 170
Therefore, Sangam had Rs 40 and Reuben had Rs 170 with them.
Question 3:
A train travelling at a uniform speed covers a certain distance. If the train would have travelled 10km/hr faster, then it would have reached 2 hours prior to the scheduled time. And if the train were slower by 10km/hr, it would have taken 3 hours more than the scheduled time. Find the distance covered by the train.
Solution:
Let the speed of the train be A km/hr and the time taken by the train to travel a distance be N hours and the distance to travel be X hours.
Speed of the train: DistancetravelledbythetrainTimetakentotravelthatdistance
A=N(distance)X(time)
Or, N = AX – – – – – – – – – – – (1)
Using the information that is given, we get:
(A+10)=X(N−2)
(A + 10) (N – 2) = X
DN + 10N – 2A – 20 = X
By using the equation (1) we get,
– 2A + 10N = 20 – – – – – – – – – – (2)
(A−10)=X(N+3)
(A – 10) (N + 3) = X
AN – 10N + 3A – 30 = X
By using the equation (1) we get,
3A – 10N = 30 – – – – – – – – – (3)
Adding equation (2) and equation (3) we get,
A = 50
Using the equation (2) we get,
( -2) x (50) + 10N = 20
-100 +10N = 20
=> 10N = 120
N = 12hours
From the equation (1) we get,
Distance travelled by the train, X = AN
=> 50 x 12
=> 600 km
Hence, the distance covered by the train is 600km.
Question 4:
The students of a class are made to stand in rows and if there are 3 students that are extra in a row, there would be 1 row lesser. And if 3 students are lesser in a row, there would be 2 more rows. Find the number of students in the class.
Solution:
Let the number of rows be A and the number of students in a row be B.
Total number of students:
= Number of rows x Number of students in a row
=AB
Using the information that is given,
First Condition:
Total number of students = (A – 1) ( B + 3)
Or AB = ( A – 1 )(B + 3) = AB – B + 3A – 3
Or 3A – B – 3 = 0
Or 3A – Y = 3 – – – – – – – – – – – – – (1)
Second condition:
Total Number of students = (A + 2 ) ( B – 3 )
Or AB = AB + 2B – 3A – 6
Or 3A – 2B = -6 – – – – – – – – – (2)
When equation (2) is subtracted from (1)
(3A – B) – (3A – 2B) = 3 – (-6)
-B + 2B = 3 + 6B = 9
By using the equation (1) we get,
3A – 9 =3
3A = 9+3 = 12
A = 4
Number of rows, A = 4
Number of students in a row, B = 9
Number of total students in a class => AB => 4 x 9 = 36
Question 5:
In a ΔZXC, ∠B=2(∠A + ∠B) and ∠C=3. What are the three angles?
Solution:
Given,
∠C = 3 ∠B = 2(∠B + ∠A)
∠B = 2 ∠A+2 ∠B
∠B=2 ∠A
∠A – ∠B= 0- – – – – – – – – – – – (i)
We know, the sum of all the interior angles of a triangle is 180O.
Thus, ∠ A +∠B+ ∠C = 180O
∠A + ∠B +3 ∠B = 180O
∠A + 4 ∠B = 180O– – – – – – – – – – – – – – -(ii)
Multiplying 4 to equation (i) , we get
8 ∠A – 4 ∠B = 0- – – – – – – – – – – – (iii)
Adding equations (iii) and (ii) we get
9 ∠A = 180O
∠A = 20O
Using this in equation (ii), we get
20O+ 4∠B = 180O
∠B = 40O
3∠B =∠C
∠C = 3 x 40 = 120O
Therefore, ∠A = 20O
∠B=40O
∠C = 120O
Question 6:
Represent the equations 5x–y = 5 and 3x – y =3 in a graph. What are the coordinates of the vertices of the triangles formed by the y-axis and these lines?
5x – y = 5
=> y =5x – 5
Its solution table will be.
| X | 2 | 1 | 0 |
| Y | 5 | 0 | -5 |
Also given,3x – y = 3
y = 3x – 3
Its solution table will be.
| X | 2 | 1 | 0 |
| Y | 3 | 0 | -3 |
The graphical representation of these lines will be as follows:

From the above graph we can see that the triangle formed is ∆ABC by the lines and the y axis. Also the coordinates of the vertices are A(1,0) , C(0,-5) and B(0,-3)
Question 7:
Find the solutions of the following set of linear equations.
(i) x/a –y/b = 0 , ax + by = a2+b2
(ii) by + ax= c, ay + bx = 1+ c
(iii) px + qy = p-q, qx – py = p+q
(iv) 152x -378y =-74
-378x + 152y = -604
(v) (a + b) y+(a-b)x = a 2− 2ab – b2
(x + y)(a+b) = a 2+ b2
Solution:
(i) x/a – y/b = 0
=> bx − ay = 0 ……. (i)
ax + by = a 2 + b 2 …….. (ii)
Multiplying a and b to equation (i) and (ii) respectively, we get
b2x − aby = 0 …………… (iii)
a2x + aby = a 3 + ab3 …… (iv)
Adding equations (iii) and (iv), we get
b2x + a 2x = a 3 + ab2
x (b2 + a2 ) = a (a2 + b2 ) x = a
Using equation (i), we get
b(a) − ay = 0
ab − ay = 0
ay = ab,
y = b
(ii)Given,
ax + by= c…………………(i)
bx + ay = 1+ c………… ..(ii)
Multiplying a to equation (i) and b to equation (ii), we obtain
a2x + aby = ac ………………… (iii)
b2x + aby = b + bc …………… (iv)
Subtracting equation (iv) from equation (iii),
(a 2 − b 2 ) x = ac − bc– b
x=c(a−b)−ba2−b2
From equation (i), we obtain
ax +by = c
a{c(a−b)−b)a2−b2}+by=c ac(a−b)−aba2−b2+by=c by=c–ac(a−b)−aba2−b2 by=a2c−b2c−a2c+abc+aba2−b2 by=abc−b2c+aba2−b2 y=c(a−b)+aa2−b2
(iii)
px + qy = p − q ……………… (i)
qx − py = p + q ……………… .. (ii)
Multiplying p to equation (1) and q to equation (2), we get
p2x + pqy = p2 − pq ………… (iii)
q2x − pqy = pq + q2 ………… (iv)
Adding equation (iii) and equation (iv),we get
p2x + q2 x = p2 + q2
(p2 + q2 ) x = p2 + q2
x=p2+q2p2+q2=1
From equation (i), we get
p(1) + qy = p – q
qy = − q y = − 1
(iv) 152x − 378y = − 74
76x − 189y = − 37
x=(189y-137)/76………(i)
− 378x + 152y = − 604
− 189x + 76y = − 302 ………….. (ii)
Using the value of x in equation (ii), we get
−189(189y−3776)+76y=−302
− (189) 2 y + 189 × 37 + (76) 2 y = − 302 × 76
189 × 37 + 302 × 76 = (189) 2 y − (76) 2y
6993 + 22952 = (189 − 76) (189 + 76) y
29945 = (113) (265) y
y = 1
Using equation (i), we get
x =(189-37)/76
x=152/76 =2
(v)
(a + b) y + (a – b) x = a2− 2ab − b2 …………… (i)
(x + y)(a + b) = a 2 + b2
(a + b) y + (a + b) x = a 2 + b 2 ………………… (ii)
Subtracting equation (ii) from equation (i), we get
(a − b) x − (a + b) x = (a 2 − 2ab − b 2 ) − (a2 + b2 )
x(a − b − a − b) = − 2ab − 2b2
− 2bx = − 2b (b+a)
x = b + a
Substituting this value in equation (i), we get
(a + b)(a − b) +y (a + b) = a2− 2ab – b2
a2 − b2 + y(a + b) = a2− 2ab – b2
(a + b) y = − 2ab
y=-2ab/(a+b)
Question 8:
A cyclic quadrilateral ABDC is given below, find its angles.

Solution:
It is know that the sum of the opposite angles of a cyclicquadrilateral is 180o
Thus, we have
∠C +∠A = 180
4y + 20− 4x = 180
− 4x + 4y = 160
x − y = − 40 ……………(1)
And, ∠B + ∠D = 180
3y − 5 − 7x + 5 = 180
− 7x + 3y = 180 ………..(2)
Multiplying 3 to equation (1), we get
3x − 3y = − 120 ………(3)
Adding equation (2) to equation (3), we get
− 7x + 3x = 180 – 120
− 4x = 60
x = −15
Substituting this value in equation (i), we get
x − y = − 40
-y−15 = − 40
y = 40-15
= 25
∠A = 4y + 20 = 20+4(25) = 120°
∠B = 3y − 5 = − 5+3(25) = 70°
∠C = − 4x = − 4(− 15) = 60°
∠D = 5-7x
∠D= 5− 7(−15) = 110°