Exercise1 

Question 1:

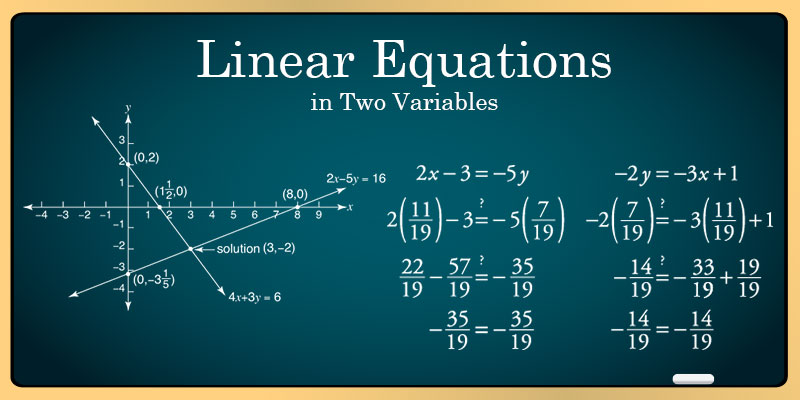
Astitva tells his daughter, “Seven years ago I was seven times as old as you were then and also three years from now, I shall be three times as old as you will be.”  Represent this situation algebraically and graphically.

Solution:

Let the present age of Astitva be ‘x’.

And, the present age of his daughter be ‘y’.

Seven years ago,

Age of Astitva = x-7

Age of his daughter =y-7

According to the question,

x−7=7(y−7) ⇒x−7=7y−49

⇒x−7y=−42         ………………………(i)

 

Three years from now,

Age of Astitva will be ‘x+3’

Age of his daughter will be ‘y+3’

According to the question,

x+3=3(y+3) ⇒x+3=3y+9

⇒x−3y=6       …………………(ii)

 

Subtracting equation (i) from equation (ii) we have

(x−3y)−(x−7y)=6−(−42) −3y+7y=6+42 ⇒4y=48 ⇒y=12

 

The algebraic equation is represented by

x−7y=−42 x−3y=6

For x−7y=−42

x=−42+7y

 

The solution table is

 

 

X	-7	0	7
Y	5	6	7

 

For  x−3y=6   or     x=6+3y

The solution table is

 

X	6	4	0
Y	0	-1	-2

 

The graphical representation is-

 

Question 2:

A cricket team coach purchases 3 bats and 3 balls for Rs 3900. Later on, he buys 1 bat and 2 more balls of the same kind for Rs 1200. Represent the situation algebraically and geometrically.

Solution:

Let us assume that the cost of a bat be ‘Rs x’

And,the cost of a ball be ‘Rs y’

According to the question, the algebraic representation is

 

3x+6y=3900 x+2y=1300

For 3x+6y=3900

x=3900−6y3

 

The solution table is

 

x	300	100	-100
y	500	600	700

 

For,

x+2y=1300 x=1300−2y

 

The solution table is

 

x	300	100	-100
y	500	600	700

 

The graphical representation is as follows.

 

Question 3:

The cost of 2 kg of apples and 1 kg of grapes on a day was found to be Rs 160. After a month, the cost of 4 kg of apples and 2 kg of grapes is Rs 300. Represent the given situation algebraically and geometrically.

Solution:

Let the cost of 1 kg of apples be ‘Rs x’

And, cost of 1 kg of grapes be ‘Rs y’

According to the question, the algebraic representation is

2x+y=160 4x+2y=300

For,  2x+y=160

y=160−2x

 

The solution table is

 

X	50	60	70
Y	60	40	20

 

For 4x+2y=300,

y=300−4x2

 

The solution table is

 

x	70	80	75
y	10	-10	0

The graphical representation is as follows.

 

Exercise-2

 

Question 1: 

Find the graphical solution for the given problems.

 (a).In Class 10^th, 10 students participated in a Maths test. If there are 4 more girls than the total number of boys, find how many girls and boys participated in the test.

(b)The total cost of 5 erasers and 7 chocolates is Rs.50, but the total cost of 7 erasers and 5 chocolates is  Rs. 46. Now, calculate the cost of one eraser and the cost of one chocolate.

Solution:

(a)Let there are x number of girls and y number of boys. As per  the given question,  it is represented as follows.

x+y=10x−y=4Nowx+y=10,x=10−y

 

X	5	4	6
Y	5	6	4

 

Forx−y=4,x=4+y

X	4	5	3
Y	0	1	-1

 

Hence, the table is represented in graphical form as follows.

From the figure, it is seen that the given lines cross each other at point (7, 3). Therefore, there are 7 girls and 3 boys in the class .

(b)Let 1 pencil costs Rs.x and 1 pen costs Rs.y. According to the question, the algebraic representation is

5x+7y=507x+5y=46For5x+7y=50, x=50−7y5

x	3	10	-4
y	5	0	10

 

7x+5y=46

 

X	8	3	-2
Y	-2	5	12

 

Hence, the graphic representation is as follows.

From the figure, it is seen that the given  lines cross each other at point (3, 5).

So, the cost of a eraser is 3/- and cost of a chocolate is 5/-.

 

Question 2:

Comparing the given ratios, a1a2,b1b2andc1c2,Figure out whether the lines are parallel or coincident:

(a) 5x−4y+8=0

7x+6y−9=0

(b) 9x+3y+12=018x+6y+24=0

(c) 6x−3y+10=02x−y+9=0

Solution:

(a) 5x−4y+8=0

7x+6y−9=0

Comparing these equations with a1x+b1y+c1=0

and a2x+b2y+c2=0

We get,

a1=5,b1=−4,c1=8a2=7,b2=6,c2=−9 a1a2=57 b1b2=−46=−23

Since a1a2≠b1b2

So, the pairs of equations given in the question have a unique solution and the lines cross each other at exactly one point.

 

(b) 9x+3y+12=018x+6y+24=0

Comparing these equations with a1x+b1y+c1=0

and a2x+b2y+c2=0

We get,

a1=9,b1=3,c1=12a2=18,b2=6,c2=−24 a1a2=918=12 b1b2=36=12 c1c2=1224=12

 

Since a1a2=b1b2=c1c2

So, the pairs of equations given in the question have infinite possible solutions and the lines are coincident.

 

(c) 6x−3y+10=02x−y+9=0

Solution: Comparing these equations with a1x+b1y+c1=0

and a2x+b2y+c2=0

We get,

a1=6,b1=−3,c1=10a2=2,b2=−1,c2=9 a1a2=62=31 b1b2=−3−1=31 c1c2=109

 

 

Since a1a2=b1b2≠c1c2

So, the pairs of equations given in the question are parallel to each other and the lines never intersect each other at any point and there is no possible solution for the given pair of equations.

 

Question 3:

Compare the given ratios, a1a2,b1b2andc1c2 find out whether the following pair of linear equations are consistent, or inconsistent.

(a) 3x+2y=5;2x−3y=7

(b) 2x−3y=8;4x−6y=9

(c) 32x+53y=7;9x−10y=14

Solution:

(a) 3x+2y=5;2x−3y=7

a1a2=32 b1b2=−23 c1c2=57

 

Since a1a2≠b1b2

So, the given equations intersect each other at one point and they have only one possible solution. The equations are consistent.

 

(b) 2x−3y=8;4x−6y=9

a1a2=24 b1b2=−3−6 c1c2=89

 

Since a1a2=b1b2≠c1c2

So, the equations are parallel to each other  and they have no possible solution. So, the equations are inconsistent.

 

(c) 32x+53y=7;9x−10y=14

a1a2=329=16 b1b2= frac53−10=−16 c1c2=714

 

Since a1a2≠b1b2

So, the equations are intersecting  each other at one point and they have only one possible solution. So, the equations are consistent.

 

Question 4:

Find whether the following pairs of linear equations are consistent or  inconsistent. If consistent, find the solution graphically:

(a)x + y = 5, 2x + 2y = 10

(b)2x + y – 6 = 0,4x – 2y – 4 = 0

Solution:

(a)x + y = 5, 2x + 2y = 10

a1a2=12 b1b2=12 c1c2=510=12

 

Since a1a2=b1b2=c1c2

∴ the equations are coincident  and they have infinite number of possible solutions.

So, the equations are consistent.

x + y = 5, 2x + 2y = 10

x	4	3	2
y	1	2	3

 

And, 2x + 2y = 10

x=10−2y2

x	4	3	2
y	1	2	3

 

So, the equations are represented as follows:

From the figure, it is seen that the lines are overlapping each other.

Therefore, the equations have infinite possible solutions.

 

Question 5:

Consider the two equations x − y + 1 = 0 and 3x + 2y − 12 = 0. Draw a graph for both. Find out the coordinates of the vertices of the triangle formed by these lines and the x-axis, and shade the triangular region.

Solution:

x−y+1=0

Or

x=y−1

x	0	1	2
y	1	2	3

 

3x+2y−12=0x=12−2y3

Hence, the graphic representation is as follows.

From the figure, it can be observed that these lines are intersecting each other at point (2, 3) and x-axis at (−1, 0) and (4, 0). Therefore, the vertices of the triangle are (2, 3), (−1, 0), and (4, 0).

 

Exercise 3

 

Question 1:

Using substitution method, solve the given pair of linear equations.

1. i) x – y = 3

x3+y2=6

1. ii) a + b = 1

a – b = 4

iii)  0.2a + 0.3b = 1.3

0.4a+0.5b = 2.3

1. iv) 3a – b = 3

9a – 3b = 9

1. v) 3x2–5y3=−2

x3+y2=136

1. vi) 2–√x+3–√y=0

3–√x–8–√y=0

Solution:

i) x – y = 3- – – – – – – – – – – (I)

x3+y2=6– – – – – – – – (II)

From (I), we get

x = y + 3- – – – – — – – – (III)

Substituting the value of x in equation (II), we get

y+33+y2=6

2y + 6 + 3y = 36

5y = 30

y = 6- – – – – – – – – (IV)

Substituting the value of y in equation (III), we get x = 9

∴ x = 9, y = 6

 

ii) a + b = 14- – – – – – – – – (I)

a – b = 4  — – – – – – – – – (II)

From (I), we get a = 14 – b- – – – – – – – – –  (III)

Substituting the value of a in equation (II), we obtain

(14 – b) – b = 4

14 – 2b = 4

10 = 2b

b = 5- – – – – – – – –  (IV)

Substituting (IV) in (III), we get

a = 14 – b

= 14 – 5

= 9

∴ a = 9, b = 5

 

iii)  0.2a + 0.3b = 1.3- – – – – – – (I)

0.4a + 0.5b = 2.3- – – – – – – –  (II)

From equation (I), we get

a=1.3−0.3b0.2 – – – – – – – – (III)

Substituting the value of a in equation (II), we get

0.4(1.3−0.3b0.2)+0.5b=2.3

2.6 – 0.6b + 0.5b = 2.3

2.6 – 2.3 = 0.1b

0.3 = 0.1b

b = 3- – – – – – – – (IV)

Substituting the value of b in equation (III), we get

a=1.3−0.3∗30.2 =1.3−0.90.2=0.40.2=2

∴ a = 2, b = 3

 

iv) 3a – b = 3- – – – – – – – (I)

9a – 3b = 9  – – – – – – – – (II)

From (I), we get b = 3a – 3 – – – – – – – – (III)

Substituting the value of b in equation (II), we get

9a – 3(3a – 3) = 9

9a – 9a + 9 = 9

9 = 9

This is always true.

The given pair of equations has infinite solutions and the relation between these variables can be given by b = 3a – 3

Therefore, one of its possible solutions is a = 1, b = 0.

 

v) 3x2–5y3=−2 – – – – – – – – (I)

x3+y2=136 – – – – – – – – (II)

From equation (I), we get

9x – 10y = -12

x=−12+10y9 – – – – – – – – (III)

Substituting the value of x in equation (II), we get

−12+10y93+y2=136 −12+10y27+y2=136 −24+20y+27y54=136

47y = 117 + 24

47y = 141

y = 3 – – – – – – – –  (IV)

Substituting the value of y in equation (III), we get

x=−12+10∗39=189=2

Hence, x = 2, y = 3

 

vi) 2–√x+3–√y=0 – – – – – – – – (I)

3–√x–8–√y=0 – – – – – – – – (II)

From equation (I), we get

x=−3√y2√ – – – – – – – –  (III)

Substituting the value of x in equation (II), we get

3–√(−3√y2√)−8–√y=0 −3y2√−22–√y=0 y(−32√−22–√)=0

y = 0- – – – – – – – (IV)

Substituting the value of y in equation (III), we get

x = 0

∴ x = 0, y = 0

 

Question 2:

Solve 2a + 3b = 11 and 2a – 4b = -24 and calculate the value of m in b = ma + 3.

Solution:

2a + 3b = 11                    (I)

2a – 4b = -24                   (II)

From equation (II), we get

a=11−3b2                   (III)

Substituting the value of a in equation (II), we get

2(11−3b2)−4b=−24

11 – 3b – 4b = -24

-7b = -35

b = 5                  (IV)

Putting the value of b in equation (III), we get

a=11−3∗52=−42=−2

Hence, a = -2, b = 5

Also,

b = ma + 3

5 = -2m +3

-2m = 2

m = -1

 

Question 3:

Using substitution method, find the solution by forming the pair of linear equations for the given problems.

i) The coach of a cricket team buys 7 bats and 6 balls. It cost her Rs 3800. Later, she buys 3 bats and 5 balls. It cost her Rs 1750. Find the cost of single bat and single

Solution:

Let the cost a bat be x and cost of a ball be y.

According to the question,

7x + 6y = 3800 …………….. (I)

3x + 5y = 1750 ……………… (II)

From (I), we get

y=3800−7x6 ………………. (III)

Substituting (III) in (II). we get

3x+5(3800−7x6)=1750 3x+95003−35x6=1750 3x−35x6=1750−95003 18x−35x6=5250−95003 −17x6=−42503

-17x = -8500

x = 500 ……………….. (IV)

Substituting the value of x in (III), we get

y=3800−7∗5006=3006=50

Hence, the cost of a bat is Rs 500 and cost of a ball is Rs 50.

 

ii) The difference between two numbers is 26 and one number is three times the older. Find the two numbers.

Solution:

Let the two numbers be x and y respectively, such that y > x.

According to the question,

y = 3x ……………… (1)

y – x = 26 …………..(2)

Substituting the value of (1) into (2), we get

3x – x = 26

x = 13 ……………. (3)

Substituting (3) in (1), we get y = 39

Hence, the numbers are 13 and 39.

 

iii) Five years hence, the age of Rahul will be three times that of his son. Five years ago, Rahul’s age was seven times that of his son. Calculate their present ages.

Solution:

Let the age of Rahul and his son be x and y respectively.

According to the question,

(x + 5) = 3(y + 5)

x – 3y = 10 ………………… (1)

(x – 5) = 7(y – 5)

x – 7y = -30 ……………….. (2)

From (1), we get x = 3y + 10 ……………………. (3)

Substituting the value of x in (2), we get

3y + 10 – 7y = -30

-4y = -40

y = 10 ………………… (4)

Substituting the value of y in (3), we get

x = 3 x 10 + 10

= 40

Hence, the present age of Rahul and his son is 40 years and 10 years respectively.

 

iv) If 2 is added to both the numerator and the denominator a fraction becomes 8/11. If 3 is added to both the numerator and the denominator it becomes 5/6. Find the fraction.

Solution:

Let the fraction be x/y.

According to the question,

x+2y+2=911

11x + 22 = 9y + 18

11x – 9y = -4 …………….. (1)

x+3y+3=56

6x + 18 = 5y +15

6x – 5y = -3 ………………. (2)

From (1), we get x=−4+9y11 …………….. (3)

Substituting the value of x in (2), we get

6(−4+9y11)−5y=−3

-24 + 54y – 55y = -33

-y = -9

y = 9 ………………… (4)

Substituting the value of y in (3), we get

x=−4+8111=7

Hence the fraction is 7/9.

 

v) The larger of two supplementary angles exceeds the smaller by 18^o. Find the two angles.

Solution:

Let the larger angle by x^o and smaller angle be b^o.

We know that the sum of two supplementary pair of angles is always 180^o.

According to the question,

x + y = 180^o……………. (1)

x – y = 18^o ……………..(2)

From (1), we get x = 180^o – y …………. (3)

Substituting (3) in (2), we get

180^o – y – y =18^o

162^o = 2y

y = 81^o ………….. (4)

Using the value of y in (3), we get

x = 180^o – 81^o

= 99^o

Hence, the angles are 99^o and 81^o.

 

vi) A taxi charge consists of a fixed charge + charge for the distance covered. For a distance of 10 km and 15 km, the charge paid is Rs 105 and Rs 155 respectively. Calculate the fixed charge and the charge per km. Also, calculate travel cost for 25 km.

Solution:

Let the fixed charge be Rs x and per km charge be Rs y.

According to the question,

x + 10y = 105 …………….. (1)

x + 15y = 155 …………….. (2)

From (1), we get x = 105 – 10y ………………. (3)

Substituting the value of x in (2), we get

105 – 10y + 15y = 155

5y = 50

y = 10 …………….. (4)

Putting the value of y in (3), we get

x = 105 – 10 * 10 = 5

Hence, fixed charge is Rs 5 and per km charge = Rs 10

Charge for 25 km = x + 25y = 5 + 250 = Rs 255

 

 

Exercise 4

Question 1:

Using the method of elimination and method of substitution, solve the given liner equations.

(1) a + b = 5 and 2a – 3b = 4

(2) 3a + 4b = 10 and 2a – 2b = 2

(3) 3a = 5b – 4 = 0 and 9a = 2b + 7

(4) a2+2b3=−1 and a−b3=3

Solution:

(1) By the method of elimination.

A + B = 5   – – – – – – – – (i)

2A – 3B = 4 – – – – – – – – (ii)

When the equation (i) and is multiplied by (ii), we get

2A + 2B = 10 – – – – – – – – (iii)

When the equation (ii) is subtracted from (iii) we get,

5B = 6

B = 65 – – – – – – – (iv)

Substituting the values obtained in (i) we get,

A=5−65=195 ∴A=195,B=65

By the method of substitution:

From the equation (i), we get:

A = 5 – B – – – – – – – (v)

When the value is put in equation (ii) we get,

2(5 – B) – 3B = 4

-5B = -6

B = 65

When the values are substituted in equation (v), we get:

A=5−65=195 ∴A=195,B=65

 

(2) By the method of elimination:

3A + 4B = 10 – – – – – – -(i)

2A – 2B = 2 – – – – — – – (ii)

When the equation (i) and (ii) is multiplied by 2, we get:

4A – 4B = 4 – – – – – – – (iii)

When the Equation (i) and (iii) are added, we get:

7A = 14

A=2 – – – – – – – – – (iv)

Substituting equation (iv) in (i) we get,

6 + 4B = 10

4B = 4

B = 1

Hence, A = 2 and B = 1

By the method of Substitution

From equation (ii) we get,

A = 1 + B – – – – – – – – (v)

Substituting equation (v) in equation (i) we get,

3(1 + B) + 4B = 10

7B = 7

B= 1

When B= 1 is substituted in equation (v) we get,

A = 1 + 1 = 2

Therefore, A =2 and B = 1

(3) By the method of elimination:

3A – 5B – 4 = 0 – – – – – – – (i)

9A = 2B + 7

9A – 2B – 7 = 0 – – – – – – – (ii)

When the equation (i) and (iii) is multiplied we get,

9A – 15B – 12 = 0 – – – – – (iii)

When the equation (iii) is subtracted from equation (ii) we get,

13B = -5

B=−513 – – – – – – – – (iv)

When equation (iv) is substituted in equation (i) we get,

3A+2513−4=0 3A=2713 A=913 ∴A=913,B=−513

By the method of Substitution:

From the equation (i) we get,

A=5B+43 – – – – – – – – (v)

Putting the value (v) in equation (ii) we get,

9(5B+43)−2B−7=0

13B = -5

B=−513

Substituting this value in equation (v) we get,

A=5(−513)+43 A=913 ∴A=913,B=−513

(4) By the method of Elimination

A2=2B3=−1

3A + 4B = -6 – – – – – – – (i)

A−B3=3

3A – B = 9 – – – – – – – (ii)

When the equation (ii) is Subtracted from equation (i) we get,

5B = -15

B = 3 – – – – – – (iii)

When the equation (iii) is substituted in (i) we get,

3A – 12 = -6

3A = 6

A = 2

Hence, A = 2 , B = -3

By the method of Substitution:

From the equation (ii) we get,

A=B+93 – – – – – – – – – (v)

Putting the value obtained from equation (v) in equation (i) we get,

3(B+93)+4B=−6

5B = -15

B= -3

When B= -3 is substituted in equation (v) we get,

A=−3+93=2

Therefore, A = 2 and B = -3

 

Question 2:

Find the solutions for the given pair of linear equation by the method of elimination for the following questions:

(1) If 1 is added in the numerator and 1 subtracted from that of the denominator, a fraction is then reduced to 1. It becomes ½ if 1 is added only to the denominator. What is the fraction?

(2) Reuben was thrice as old as Alex, 5 years back. And Reuben will be twice as old as Alex, 10 years from now. What are the ages of Reuben and Alex?

(3) The sum of digits of a two digit number is 9. Also when this number is multiplied nine times, it is two times the number when obtained by reversing the order of the digits. Find the number.

(4) Manna went to the bank to withdraw a sum of Rs 2000. She had asked the cashier to give her Rs 50 and Rs 100 notes only. Manna got 25 notes in total. Find how many Rs 50 notes and Rs 100 notes she had received.

(5) A lending library has a fixed charge for the first three days and an additional charge for each day thereafter. Sara paid a sum of Rs 27 for a book to keep it for seven days, while Rosy paid a sum of Rs 21 for the book that she had kept for five days. Find the fixed charge and the charge that will cost for each extra day.

Solution:

(1) Let the fraction be a/b

According to that of the given information,

A+1B−1=1 => A – B = -2 – – – – – – – – – – – (i)

AB+1=12 => 2A – B = 1 – – – – – – – – (ii)

When equation (i) is Subtracted from equation (ii) we get A = 3 – – – – – – (iii)

When A = 3 is substituted in equation (i) we get,

3 – B = -2

-B = -5

B = 5

Hence, the fraction is 3/5

 

(2) Let the present age of Reuben = a

And the present age of Alex = b

According to the information that is given,

(A – 5 ) = 3 ( B – 5 )

A – 3B = -10 – – – – – – – – – (i)

(A + 10) = 2(B + 10)

A – 2B = 10 – – – – – – – – – – – (ii)

When the equation (i) is subtracted from equation (ii) we get,

B = 20 – – – – – – – – – (iii)

Substituting B = 20 in equation (i), we get:

A – 60 = -10

A = 50

Hence, the present age of Reuben is 50 yrs

And the present age of Alex is 20 yrs

 

(3) Let the unit digit and tens digit of a number be A and B respectively.

Then, Number (n) = 10B + A

N after reversing the digits = 10A + B

According to the given information, A + B = 9 – – – – – – – – – (i)

9(10B + A) = 2(10A + B)

88 B – 11 A = 0

-A + 8B = 0 – – – – – – – – (ii)

Adding the equations (i) and (ii) we get,

9B = 9B

= 1 (3)

Substituting this value in the equation (i) we get A= 8

Hence the number (N) is 10B + A = 10 x 1 +8 = 18

 

(4) Let the number of Rs 50 notes be A and the number of Rs 100 notes be B

According to the given information,

A + B = 25 – – – – – – – – – – – (i)

50A + 100B = 2000 – – – – – – – (ii)

When equation (i) is multiplied with (ii) we get,

50A + 50B = 1250 – – – – – – – – – (iii)

Subtracting the equation (iii) from the equation (ii) we get,

50B = 750

B = 15

Substituting in the equation (i) we get,

A = 10

Hence, Manna has 10 notes of Rs 50 and 15 notes of Rs 100.

 

(5) Let the fixed charge for the first three days be Rs A and the charge for each day extra be Rs B.

According to the information given,

A + 4B = 27 – – – – – (i)

A + 2B = 21 – – – – – – (ii)

When equation (ii) is subtracted from equation (i) we get,

2B = 6

B = 3 – – – – – – (iii)

Substituting B = 3 in equation (i) we get,

A + 12 = 27

A = 15

Hence, the fixed charge is Rs 15

And the Charge per day is Rs 3

 

  Exercise-5

Question 1:

 From the given pairs of linear equations find the ones with unique solution, no solution or infinitely many solutions. If there is a unique solution, find it by using cross multiplication method.

(i) x-3y-3 =0 and  3x-9y-2=0               (ii) 2x+y =5 and 3x +2y =8

(iii)6x – 10y =40 and 3x-5y = 20    (iv)x -3y – 7 =0  and 3x -3y -15=0

 

Solution:

(i)      3x – 9y -2 =0

x – 3y – 3 =0

a₁/a₁=1/3 ,         b1/b2  = -3/-9 =1/3,     c₁/c₂=-3/-2 = 3/2

a1a2=b1b2≠c1c2

Since, the given set of lines are parallel to each other they will not intersect each other and     therefore there is no solution for these equation.

 

(ii) 2x + y = 5

3x +2y = 8

a1a2=23,b1b2=12,c1c2=−5−8 a1a2≠b1b2

Since they intersect at a  unique point these equations will have a unique solution by cross multiplication method:

xb1c2−c1b2=yc1a2−c2a1=1a1b2−b1a2 x−8+10=y−15+16=14−3

x/ 2 = y/1 = 1

x =2 , y =1.

 

(iii) 6x -10y = 40

3x – 5y = 20

a₁ / a₂ = 3/6 = 1/2 ,               b₁/b₂ = -5/-10 =1/2,     c₁/c₂ =-20/-40 = ½

a₁/a₂ = b₁/b₂ = c₁/c₂

Since the given sets of lines are overlapping each other there will be infinite number of solutions for this pair of equation.

 

(iv) 3x – 3y – 15 =0

x – 3y – 7 = 0

a₁/a₂ = 1/3,     b1/b2 = 1 ,   c₁/c₂ = -7/-15

a1a2≠b1b2

Since this pair of lines are intersecting each other at a unique point, there will be  a unique solution.

By cross multiplication,

x/(45-21) = y/(-21+15) = 1/(-3+9))

x/24 = y/-6 =1/6

x/24 = 1/6 and y/-6 = 1/6

∴  x = 4 and y =1.

 

Question 2:

(i) Find the values of a and b at which the following pair of linear equations will have infinite solution.

3y + 2x = 7

(a+b)y +(a-b)x = 3a + b -2

(ii) Find the value of k at which the following set of linear equations won’t have a solution.

3x + y =1

(2k – 1)x + (k-1)y = 2k + 1

Solution:

(i) 3y + 2x -7 =0

(a + b) y + (a-b)y – (3a + b -2) = 0

a₁/a₂=2/a-b ,               b₁/b₂=3/a+b ,               c₁/c₂=-7/-(3a + b -2)

For infinitely many solutions,

a₁/a₂ = b₁/b₂ = c₁/c₂

2/a-b = 7/3a + b – 2

6a + 2b – 4 = 7a – 7b

a – 9b = -4  ……(i)

2/a-b = 3/a+b

2a + 2b = 3a – 3b

a -5b = 0 …….(ii)

Subtracting (i) from (ii), we get

4b = 4

b =1

Substituting this eqn in (ii), we get

a -5 x 1= 0

a = 5

Thus at a = 5 and b = 1 the given equations will have infinite solutions.

 

(ii) 3x + y -1 = 0

(2k -1)x  +  (k-1)y – 2k -1 = 0

a₁/a₂ = 3/2k -1 ,           b₁/b₂=1/k-1 ,c₁/c₂ = -1/-2k -1 = 1/ 2k +1

For no solutions

a₁/a_{2  =} b₁/b₂ ≠ c₁/c₂

3/2k-1 = 1/k -1   ≠ 1/2k +1

3/2k – 1 = 1/k -1

3k -3 = 2k -1

k =2

Therefore, for k = 2 the given pair of linear equations will have no solution.

 

Question 3:

Solve the given pair of linear equations using cross multiplication method and substitution method:

3x + 2y = 4

8x + 5y =9

Solution:

1. 8x + 5y = 9 ….(1)

3x   +  2y = 4 ….(2)

From equation (2) we get

x = 4 – 2y / 3  …. (3)

Using this value in equation 1 we get

8(4-2y)/3 + 5y = 9

32 – 16y +15y = 27

-y = -5

y = 5 ……(4)

Using this value in equation (2), we get

3x + 10 = 4

x = -2

Thus , x = -2 and y = 5.

 

Now, Using Cross Multiplication method:

8x +5y – 9 = 0

3x + 2y – 4 = 0

x/(-20+18) = y/(-27 + 32 ) = 1/(16-15)

-x/2 = y/5 =1/1

Thus , x = -2 and y =5.

 

Question 4:

For the problems given below, form a pair of linear equations and also solve them (if a solution exists).

(i) When 1 is subtracted from the numerator of a fraction it becomes 1/3 and it becomes 1/4 when 8 is added to the denominator. Find the fraction.

(ii) Gah and Hag are 100kms apart on a highway. A car starts from Gah and another from Hag at the same time. If the cars move towards each other, they meet in one hour and if they move in the same direction, they meet after 5 hours. Find the speed of the two cars.

(iii) Monthly hostel charges are fixed, except for the mess charge which is included in it. Dom a eats for 20 days in the mess and pays Rs1000 as her monthly hostel charge. Nimi eats for 26 days and pays Rs.1180 as hostel charges. What is the fixed charge and the cost of food per day?

(iv) Lama scored 40 in an exam, acquiring 3 marks higher for each right answer and losing 1 mark for every wrong answer. If 4 marks had been awarded for every correct answer and 2 marks reduced for every wrong answer, Lama would have scored 50 marks. Find the number of questions in the exam.

Solution:

(i)    let the fraction be x/y

So, according to question :

(x-1)/y = 1/3 ­­­ =>  3x – y = 3…..(1)

x/(y + 8) = 1/4  => 4x –y =8 ….(2)

Subtracting equation (1) from (2) , we get

x = 5 ……(3)

Using this value in equation (2) we get

4×5 – y = 8

y= 12.

Therefore, the fraction is 5/12.

 

(ii)Let the speed of the first and second cars be x km/h and y km/h respectively.

Respective speed of the two cars when they are moving in the same direction = (x -y) km/h

Respective speed of the two cars when they are headed towards each other = (x + y)km/h

According to the question;

5(x-y) =100

    x – y =20 ….. (1)

Also,

1(x +y )=100 …..(2)

Adding equations (1) and (2) we get

2x =120

x = 60 km/h ……(3)

Using this in equation (1) we get

60-20= y

y= 40 km/h.

 

(iii) let x be the fixed charge and y be the charge of food per day.

According to the question

x + 20y = 1000…. (i)

x +  26y = 1180….(ii)

Subtracting (i) from  (ii) we get

6y=180

y= Rs.30

Using this value in equation (ii) we get

x = 1180 -26 x 30

= Rs.400.

 

(iv) Let x be the number of correct numbers and y be the number of incorrect answers.

According to the question,

3x – y = 40 …..(i)

4x – 2y = 50

    2x-y = 25….(ii)

Subtracting equation (ii) from (i), we get

x=15…………(iii)

Using this in equation (i) we get

3(15) – 40 =y

y= 5

Therefore, the number of correct answers = 15

the number of incorrect answers= 5

the number of questions              =20

 

  EXERCISE – 6

 

Solve the following pairs of equations by reducing them to a pair of linear equations:

Question 1:

 12a+13b=2

 13a+12b=136

 Solution:

 Let us assume 1a=mand1b=n, then the equation will change as follows.

m2+n3=2⇒3m+2n−12=0   …….(1)

m3+n2=136⇒2m+3n−13=0   …….(2)

Now, Using cross-multiplication method, we get

m−26−(−36)=n−24−(−39)=19−4 m10=q15=15 m10=15andq15=15

m = 2 and n = 3

1a=2and1b=3 a=12andb=13

 

Question 2:

4a+3b=14

4a−4b=23

 

Solution:

 Putting 1a=m in the given equation we get,

So, 4m + 3b = 14     => 4m + 3b – 14 = 0  ………..(1)

3m – 4b = 23     => 3m – 4b – 23 = 0  ………..(2)

By cross-multiplication, we get,

m−69−56=b−42−(−92)=1−16−9 m−125=b50=−125 m−125=−125andb50=−125

m = 5 and b = -2

m = 1a = 5

a = 15

b = -2

 

Question 3:

7a−2bab=5

7b−2a=5 …..(i)

8a+7bab=15

8b+7a=15 …..(ii)

Solution:

 Substituting 1a=m in the given equation we get,

– 2m + 7n = 5     => -2 + 7n – 5 = 0  ……..(iii)

7m + 8n = 15     => 7m + 8n – 15 = 0 ……(iv)

By cross-multiplication method, we get,

m−105−(−40)=n−35−30=1−16−49 m−65=n−65=1−65 m−65=1−65andb−65=1−65

m = 1 and n = 1

m = 1a = 1        n = 1a = 1

a = 1    b = 1

 

Question 4:

10a+b+2a−b=4

15a+b−5a−b=−2

Solution:

Substituting 1a+b=m and 1a−b=n in the given equations, we get,

10m + 2n = 4      =>  10m + 2n – 4 = 0      …..(i)

15m – 5n = -2     =>   15m – 5n + 2 = 0    …..(ii)

Using cross-multiplication method, we get,

m4−20=n−60−(−20)=1−50−30 m−16=n−80=1−80 m−16=1−80andb−80=1−80

m = 15 and n = 1

m = 1a+b=15 and n = 1a−b=1

a + b = 5  …..(iii)

and a – b = 1 …..(iv)

Adding equation (iii) and (iv), we get

2a = 6   => a = 3 …….(v)

Putting the value of a = 3 in equation (3), we get

b = 2

Hence, a = 3 and b = 2

 

Question 5:

2a√+3b√=2

4a√−9b√=−1

 

Solution:

 Substituting 1a√=m and 1b√=n in the given equations, we get

2m + 3n = 2 …….(i)

4m – 9n = -1 ……(ii)

Multiplying equation (i) by 3, we get

6m + 9n = 6 ………..(iii)

Adding equation (ii) and (iii), we get

10m = 5

m = 12 ……(iv)

Now by putting the value of ‘m’ in equation (i), we get

2×12+2n=2

3n = 1

n = 13

m = 1a√=12

a−−√=2

a = 4

n = 1b√=13

b√=3

b = 9

Hence, a = 4 and b = 9

 

Question 6:

5a−1+1b−2=2

     6a−1−3b−2=1

 

Solution:

 Substituting 1a−1=m and 1b−2=n in the given equations, we get,

5m + n = 2 ……(i)

6m – 3n = 1 …..(ii)

Multiplying equation (i) by 3, we get

15m + 3n = 6 ……..(iii)

Adding (ii) and (iii) we get

21m = 7

m = 13

Putting this value in equation (i), we get

5×13+n=2 n=2−53=13 m=1a−1=13⇒a−1=3⇒a=4 n=1b−2=13⇒b−2=3⇒b=5

Hence, a = 4 and b = 5

 

Question 7:

6a + 3b = 6ab

=> 6b+3a=6 …..(i)

    2a + 4b = 5ab

=> 2b+4a=5 …….(ii)

Substituting 1a=m and 1b=n
3m + 6n – 6 = 0

4m + 2n – 5 = 0

 

By cross-multiplication method, we get

m−30−(−12)=n−24−(−15)=16−24 m−18=n−9=1−18 m−18=1−18andn−9=1−18

m = 1 and n = 1/2

m = 1/a = 1 and n = 1/b = 1/2

a = 1                              b = 2

Hence, a = 1 and b = 2

 

Question 8:

13a+b+13a−b=34

     12(3a+b)−12(3a−b)=−18

 

Solution:

 substituting 13a+b=mand13a−b=n in the given equations, we get

m + n = 3/4  ……(1)

m/2 – n/2 = -1/8

m – n = -1/4  ……(2)

Adding (1) and (2), we get

2m = 3/4 – 1/4

2m = 1/2

Putting in (2), we get

1/4 – n = -1/4

n = 1/4 + 1/4 = 1/2

 

m = 13a+b=14

3a + b = 4  …….(3)

n = 13a−b=12

3a – b = 2 ………(4)

Adding equations (3) and (4), we get

6a = 6

a = 1 ……..(5)

Putting in (3), we get

3(1) + b = 4

b = 1

Hence, a = 1 and b = 1

 

Exercise 7

 

Question 1:

The ages of Reuben and Alex are at a difference of 3 yrs. Reuben’s father Thomas is twice as old as Reuben and Alex is twice as old as his sister Ann. The ages of Ann and Thomas are at a difference of 30 yrs. What is the present age of Reuben and Alex?

Solution:

The age difference between Reuben and Alex is 3 yrs.

Either Alex is 3 yrs older than that of Reuben or Reuben is 3 yrs older than Alex. From both the cases we find out that Reuben’s father’s age is 30 yrs more than that of Ann’s age.

Let the ages of Reuben and Alex be A and B respectively.

Therefore, the age of Thomas = 2 x A = 2A yrs.

And the age of Alex’s sister Ann B/2 yrs

By using the information that is given,

Case (i)

When Reuben is older than that of Alex by 3 yrs then A – B = 3 – – – – – – – – (1)

2A−B2=30

4A – B = 60 – – – – – – – – – – – (2)

By subtracting the equations (1) and (2) we get,

3A = 60 – 3 = 57

A=573=19

Therefore, the age of Reuben = 19 yrs

And the age of Alex is 19 – 3 = 16 yrs.

Case (ii)

When Alex is older than Reuben,

B – A = 3 – – – – – – – – – (1)

2A−Y2=30

4A – B = 60 – – – – – – – – – (2)

Adding the equation (1) and (2) we get,

3A = 63

A = 21

Therefore, the age of Reuben is 21 yrs

And the age of Alex is 21 + 3 = 24 yrs.

 

Question 2:

Sangam says, “Give me Rs100, bro! I’ll become two times richer than you” Reuben replies, “If you give me Rs 10, I’ll become six times richer than you” What is the capital amount of Sangam and Reuben.

[Hint: A + 100 = 2 ( B – 100) , B + 10 =6(A- 10)]

Solution: 
Let Sangam have Rs A with him and Reuben have Rs B with him.

Using the information that is given we get,

A + 100 = 2(B – 100) A + 100 = 2B – 200

Or A – 2B = -300 – – – – – – – (1)

And

6(A – 10) = ( B + 10 )

Or 6A – 60 = B + 10

Or 6A – B = 70 – – – – – – (2)

When equation (2) is multiplied by 2 we get,

12A – 2B = 140 – – – – – – – (3)

When equation (1) is subtracted from equation (3) we get,

11A = 140 + 300

11A = 440A

=> 40

Using A =40 in equation (1) we get,

40 – 2B = -300

40 + 300 = 2B

2B = 340

B = 170

Therefore, Sangam had Rs 40 and Reuben had Rs 170 with them.

 

Question 3:

A train travelling at a uniform speed covers a certain distance. If the train would have travelled 10km/hr faster, then it would have reached 2 hours prior to the scheduled time. And if the train were slower by 10km/hr, it would have taken 3 hours more than the scheduled time. Find the distance covered by the train.

Solution:

Let the speed of the train be A km/hr and the time taken by the train to travel a distance be N hours and the distance to travel be X hours.

Speed of the train: DistancetravelledbythetrainTimetakentotravelthatdistance

A=N(distance)X(time)

Or, N = AX – – – – – – – – – – – (1)

Using the information that is given, we get:

(A+10)=X(N−2)

(A + 10) (N – 2) = X

DN + 10N – 2A – 20 = X

By using the equation (1) we get,

– 2A + 10N = 20 – – – – – – – – – – (2)

(A−10)=X(N+3)

(A – 10) (N + 3) = X

AN – 10N  + 3A – 30 = X

By using the equation (1) we get,

3A – 10N = 30 – – – – – – – – – (3)

Adding equation (2) and equation (3) we get,

A = 50

Using the equation (2) we get,

( -2) x (50) + 10N = 20

-100 +10N = 20

=> 10N = 120

N = 12hours

From the equation (1) we get,

Distance travelled by the train, X = AN

=> 50 x 12

=> 600 km

Hence, the distance covered by the train is 600km.

 

Question 4:

The students of a class are made to stand in rows and if there are 3 students that are extra in a row, there would be 1 row lesser. And if 3 students are lesser in a row, there would be 2 more rows. Find the number of students in the class.

Solution:

Let the number of rows be A and the number of students in a row be B.

Total number of students:

= Number of rows x Number of students in a row

=AB

Using the information that is given,

First Condition:

Total number of students = (A – 1) ( B + 3)

Or AB = ( A – 1 )(B + 3) = AB – B + 3A – 3

Or 3A – B – 3 = 0

Or 3A – Y = 3 – – – – – – – – – – – – – (1)

Second condition:

Total Number of students = (A + 2 ) ( B – 3 )

Or AB = AB + 2B – 3A – 6

Or 3A – 2B = -6 – – – – – – – – – (2)

When equation (2) is subtracted from (1)

(3A – B) – (3A – 2B) = 3 – (-6)

-B + 2B = 3 + 6B = 9

By using the equation (1) we get,

3A – 9 =3

3A = 9+3 = 12

A = 4

Number of rows, A = 4

Number of students in a row, B = 9

Number of total students in a class => AB => 4 x 9 = 36

 

Question 5:

 In a ΔZXC, ∠B=2(∠A + ∠B) and ∠C=3. What are the three angles?

Solution:

Given,

∠C = 3 ∠B = 2(∠B + ∠A)

∠B = 2 ∠A+2 ∠B

∠B=2 ∠A

∠A – ∠B= 0- – – – – – – – – – – –  (i)

We know, the sum of all the interior angles of a triangle is 180^O.

Thus, ∠ A +∠B+ ∠C = 180^O

∠A + ∠B +3 ∠B = 180^O

∠A + 4 ∠B = 180^O– – – – – – – – – – – – – – -(ii)

Multiplying 4 to  equation (i) , we get

8 ∠A – 4 ∠B = 0- – – – – – – – – – – – (iii)

Adding equations (iii) and (ii) we get

9 ∠A = 180^O

∠A = 20^O

Using this in equation (ii), we get

20^O+ 4∠B = 180^O

∠B = 40^O

3∠B =∠C

∠C = 3 x 40 = 120^O

Therefore, ∠A = 20^O

∠B=40^O

∠C = 120^O

 

Question 6:

 Represent the equations 5x–y = 5 and 3x – y =3 in a graph. What are the coordinates of the vertices of the triangles formed by the y-axis and these lines?

5x – y = 5

=> y =5x – 5

Its solution table will be.

X	2	1	0
Y	5	0	-5

 

Also given,3x – y = 3

y = 3x – 3

Its solution table will be.

X	2	1	0
Y	3	0	-3

 

The graphical representation of these lines will be as follows:

From the above graph we  can see that the triangle formed is ∆ABC by the lines and the y axis. Also the coordinates of the vertices are A(1,0) ,  C(0,-5) and B(0,-3)

 

Question 7:

 Find the solutions of the following set of linear equations.

(i) x/a –y/b = 0 , ax + by = a²+b²

(ii) by + ax= c,  ay + bx = 1+ c

(iii) px + qy = p-q, qx – py = p+q

(iv) 152x -378y =-74

 -378x + 152y = -604

(v) (a + b) y+(a-b)x = a ²− 2ab – b²

       (x + y)(a+b) = a ²+ b²

Solution:

(i) x/a – y/b = 0

=>  bx − ay = 0 ……. (i)

ax + by = a 2 + b 2 …….. (ii)

Multiplying a and b to equation (i) and (ii) respectively, we get

b²x − aby = 0 …………… (iii)

a²x + aby = a ³ + ab³ …… (iv)

Adding equations (iii) and (iv), we get

b²x + a ²x = a ³ + ab²

x (b² + a² ) = a (a² + b2 ) x = a

Using equation (i), we get

b(a) − ay = 0

ab − ay = 0

ay = ab,

y = b

 

(ii)Given,

ax + by= c…………………(i)

bx + ay = 1+ c………… ..(ii)

Multiplying a to equation (i) and  b to equation (ii), we obtain

a²x + aby = ac ………………… (iii)

b²x + aby = b + bc …………… (iv)

Subtracting equation (iv) from equation (iii),

(a 2 − b 2 ) x = ac − bc– b

x=c(a−b)−ba2−b2

From equation (i), we obtain

ax +by = c

a{c(a−b)−b)a2−b2}+by=c ac(a−b)−aba2−b2+by=c by=c–ac(a−b)−aba2−b2 by=a2c−b2c−a2c+abc+aba2−b2 by=abc−b2c+aba2−b2 y=c(a−b)+aa2−b2

 

(iii)

px + qy = p − q ……………… (i)

qx − py = p + q ……………… .. (ii)

Multiplying p to equation (1)  and q to equation (2), we get

p²x + pqy = p² − pq ………… (iii)

q²x − pqy = pq + q² ………… (iv)

 

Adding equation (iii) and equation (iv),we get

p²x + q² x = p²  + q²

(p² + q² ) x = p² + q²

x=p2+q2p2+q2=1

From equation (i), we get

p(1) + qy = p – q

qy = − q y = − 1

 

(iv) 152x − 378y = − 74

76x − 189y = − 37

x=(189y-137)/76………(i)

− 378x + 152y = − 604

− 189x + 76y = − 302 ………….. (ii)

Using the value of x in equation (ii), we get

−189(189y−3776)+76y=−302

− (189) ² y + 189 × 37 + (76) ² y = − 302 × 76

189 × 37 + 302 × 76 = (189) ² y − (76) ²y

6993 + 22952 = (189 − 76) (189 + 76) y

29945 = (113) (265) y

y = 1

Using equation (i), we get

x =(189-37)/76

x=152/76 =2

 

(v)

(a + b) y + (a – b) x = a²− 2ab − b² …………… (i)

(x + y)(a + b)  = a ² + b²

(a + b) y + (a + b) x  = a 2 + b 2 ………………… (ii)

Subtracting equation (ii) from equation (i), we get

(a − b) x − (a + b) x = (a ² − 2ab − b ² ) − (a² + b² )

x(a − b − a − b) = − 2ab − 2b²

− 2bx = − 2b (b+a)

x =  b + a

Substituting this value in equation (i), we get

(a + b)(a − b)  +y (a + b)  = a²− 2ab – b²

a² − b² + y(a + b)  = a²− 2ab – b²

(a + b) y = − 2ab

y=-2ab/(a+b)

 

Question 8:

A cyclic quadrilateral ABDC is given below, find its angles.

Solution:

It is know that  the sum of the opposite angles of a cyclicquadrilateral is 180^o

Thus, we have

∠C +∠A = 180

4y + 20− 4x = 180

− 4x + 4y = 160

x − y = − 40 ……………(1)

And, ∠B + ∠D = 180

3y − 5 − 7x + 5 = 180

− 7x + 3y = 180 ………..(2)

Multiplying 3 to equation (1), we get

3x − 3y = − 120 ………(3)

Adding equation (2) to equation (3), we get

− 7x + 3x = 180 – 120

− 4x = 60

x = −15

Substituting this value in equation (i), we get

x − y = − 40

-y−15 = − 40

y = 40-15

= 25

∠A = 4y + 20 = 20+4(25)  = 120°

∠B = 3y − 5 = − 5+3(25)  = 70°

∠C = − 4x = − 4(− 15) = 60°

∠D =  5-7x

∠D=  5− 7(−15) = 110°

Related Links