General Form of an A.P:

Let us consider a series with n number of terms:

a₁ + a₂ + a₃ + a₄ + a₅ + a₆ + a₇ ……………………………..  = a_n

The above series of numbers is said to form an Arithmetic Progression (A.P) if,

a₂ – a₁ = a₃ – a₂ = a₄ – a₃ = …………………………. a_n – a_n-1 = d

Here, d = common difference of the A.P and it can be positive negative or zero.

Thus the general form of an A.P can be written as:

a, a+ d, a+ 2d, a+ 3d, a+ 4d, a+ 5d …………………………. a + (n – 1)d

Where a = First Term

And,    d = Common Difference

 

Exercise 5.1

Q-1: From the given A.P write the first term (a) and common difference (d). 

(i) 2, 4, 6, 8, 10…..

Sol.

Here, a = 2

Since d = a₂ – a₁

Therefore, d = 4 – 2 = 2

Q-2: Check whether the following list of numbers form an A.P? If they form an A.P then write next three terms.

(i) 5, 25, 45, 65………

Sol.

Here, a = 5

Since for an A.P:     a₂ – a₁ = a₃ – a₂ = a₄ – a₃

Therefore,      25 – 5 = 45 – 25 = 65 – 45 = 20

Hence the given number forms an A.P with d = 20

Therefore next three terms of this A.P are: 65 + 20 = 85

85 + 20 =105

105 + 20 = 125

(ii) 3–√,6–√,9–√,12−−√,…………..

Sol.

Here, a = 3–√

Since for an A.P:     a₂ – a₁ = a₃ – a₂ = a₄ – a₃

Therefore, 6–√−3–√=9–√−6–√=12−−√−9–√=3–√

Hence the given number forms an A.P with d = 3–√

Therefore, next three terms of this A.P are:                            12−−√+3–√=15−−√15−−√+3–√=18−−√18−−√+3–√=21−−√

(iii) −12,−14,−18……

Sol.

Here, a = −12

Since for an A.P:      a₂ – a₁ = a₃ – a₂ = a₄ – a₃

Therefore, −14−12≠−18−14

Hence the given numbers does not form an A.P

The n^th term of an A.P is given by:

           a_n = a + (n – 1)×d

Here,  a = first term of an A.P and d = common difference

Example-1: Let us consider an A.P 2, 7, 12, 17……. Find its 9^th term.

Sol.

Here,                   a = 2

d = 7 – 2 = 17 – 12 = 5

n = 9

Therefore,   a₉ = 2+ (9 – 1)5

a₉ = 42

Therefore, 9^th term of this A.P = 4

Example-2 Fourth term of an A.P is 12 and Sixth term of an A.P is 18. Determine the A.P

Sol.

Given, a₄ = 12 and a₆ = 18

Let a be the first term and d be the common difference of an A.P

Then               12 = a + (4 – 1)d

Therefore,     12 = a + 3d . .  . . (1)

And                 18 = a + (6 – 1)d

Therefore      18 = a + 5d . . . . . . . (2)

Now, equation (2) – equation (1)

18 – 12 = 5d – 3d    Therefore d = 3

Putting d = 3 in equation (2) we get

18 = a + 5×(3)

Therefore a = 3

Hence, the required A.P is 3, 6, 9, 12, 15, 18, 21………

 

Example -3 Determine which term of the following A.P : 14, 9, 4 . . . . . . is -96 ?

Sol.

From the given A.P,

a = 14,

d = -5,

a_n = -96,

n =??

Since,            a_n = a + (n – 1)d

Therefore   -96 = 14 + (n – 1)×-5

(or)                5n = -96 -14 -5

Therefore       n = -23 (neglecting –ve sign)

Therefore, 23^rd term of this A.P is -96.

 

Example-4 Check whether 328 is the term in the following A.P: 76, 97, 118, 139………

Sol.

From the given A.P:

a = 76,

d = 97 – 76 = 21,

a_n = 328

Since,                                       a_n = a + (n – 1)d

Therefore                            328 = 76 + (n – 1)×21

(or)                                       21n = 328 + 21 – 76

(or)                                           n = 13

Since n is a +ve integer

Therefore 328 is the 13^th term of this given A.P

 

Example -5 Find how many two digit numbers are divisible by 5.?

Sol.

Two digit numbers that are divisible by 5 are: 10, 15, 20, 25…………………95

Now, from the given A.P

a = 10

d = 5

a_n = 95

n = ??

Since,         a_n = a + (n – 1)d

Therefore 95 = 10 + (n – 1)×5

(or)         5n = 95 – 10 + 5

n = 18

Therefore there are 18 two digit numbers that are divisible by 5.

Example-6 From the given A.P: 15, 8, 1, -6………….. -111. Find 8^th term from last term.

Sol.

On reversing the given A.P:

a = -111 (in this case, last term will now become its first term)

d = 7 (If we go from first term to last term then d = -7 (8 – 15), so if we reverse this A.P d will become +7)

n = 8

a₈ = ??

Since,          a_n = a + (n – 1)d

Therefore, a₈  = -111 + (8 – 1)×7 = -111 + 49

a₈ = -62

Therefore, 8^th term from last term = -62

Exercise – 5.2

Q.1 In the given A.P: 26, 40, 54, 68 ………………. which term is 208?

Sol.

From the given A.P:

a = 26,

d = 40 – 26 = 14,

a_n  = 208,

n = ??

Since,             a_n = a + (n – 1)d

Therefore, 208 = 26 + (n – 1)×14

14n = 208 + 14 – 26

Hence,            n = 14

14^th term of this given A.P is 208

 

Q2. Find how many total numbers of terms are there in each of the following A.P

(i) 17, 26, 35, 44,……………………….179

Sol.

From the given A.P :

a = 17,

d  = (26 – 17) = 9,

a_n = 179

n = ??

since,         a_n = a + (n – 1)d

Therefore, 179 = 17 + (n – 1)×9

9n = 179 – 17 + 9

Therefore n = 19

Hence, there are 19 terms in this given A.P

(ii)13,223,433,…………………….3373

From the given A.P :

a = 13,

d = 223–13=7,

a_n = 3373,

n = ??

Since, a_n = a + (n – 1)d

Therefore, 3373=13+(n−1)7

21n=337+21-1

Therefore n= 17

Hence, there are 17 terms in this given A.P

 

Q3. Seventh term of an A.P is -1 and fourth term is 41. Determine the A.P and hence find its 17^th term.

Sol.

Given, a₇ = -1 and a₄ = 41

Let a be the first term and d be the common difference of an A.P

-1 = a + (7 – 1)d

-1 = a + 6d . . . . . . .  . . (1)

And   41 = a + (4 – 1)d

41 = a + 3d . . . . . . . (2)

Now, equation (1) – equation (2)

-1 – 41 = 6d – 3d    Therefore,   d = -14

Putting d = -14 in equation (2) we get

41 = a + 3×(-14)

Therefore, a = 83

Therefore the required A.P is 83, 69, 55, 12, 41, 27, 13………

Now, from the given A.P:

a = 83,

d = 69 – 83 = -14

n = 17

a₁₇ = ??

Since,                   a_n = a + (n – 1)d

Therefore          a₁₇ = 83 + (17 – 1)× -14

a₁₇ = 208 + 14 – 26

a₁₇ = -141

Therefore 17^th term of this given A.P is -141

 

Q.4 There are 26 terms in an A.P of which fifth term is 61 and the last term is 292. Find 12^th term

Sol.

Given,

a₅ = a + (5-1)d = 61

61 = a + 4d…………………………………(1)

a₂₆ = a + (26 – 1)d = 292

292 = a + 25d……………………………(2)

Equation (2) – Equation (1)

292 – 61 = 25d – 4d

Therefore, d = 11

Putting the value of d in equation (1) we get

61 = a + 4 × 11

Therefore, a = 17

Hence a₁₂ = 17 + (12 – 1)×11 = 138

Therefore 12^th term of this given A.P is 138

 

Q.5 Find which term of an A.P is zero, if fourth term and tenth of an A.P are -26 and 52 respectively.

Sol.

Given,

a_n = 0

a₄ = a + (4 – 1)d = -26

-26 = a + 3d………………………………(1)

a₁₀ = a + (10 – 1)d = 52

52 = a + 9d……………………………(2)

Equation (2) – Equation (1) we get:

52 + 26 = 9d – 3d

Therefore, d = 13

On putting the values of d in equation (1) we get:

-26 = a + 3×13

Therefore, a = -65

Now, let n^th term be 0

Therefore,  0 = a + (n – 1)d

0 = -65 + (n – 1)×13

0 = -65 + 13n -13

n = 6

Therefore 6^th term of the given A.P is zero.

 

Q.6 Find the common difference d, if 14^th term of an A.P exceeds its 7^th term by 49.

Sol.

Since,             a_n = a + (n – 1)d

Therefore,   a₁₄ = a + (14 – 1)d

Similarly,      a₇ = a + (7 – 1)d

Now, according to the given condition:

  a₁₄ – a₇  = 49

49 = {a + (14 – 1)d} – {a + (7 – 1)d}

49 = 13d – 6d

Therefore, d = 7 (Common Difference) 

 

Q.7 Consider a series : 3, 20, 37, 54,71 …………………………..,Which term of this given A.P will be 289 more than its 32^nd term.

Sol.

Given, a = 3

d = 20 – 3 = 17

Therefore,  a₃₂ = a + (32 – 1)×d

a₃₂ = 3 + 31×17

a₃₂ = 530

Now, according to the given condition:

  a_n – a₃₂ = 289    (Since, a_n > a₃₂)

(3+ (n – 1)17) – 530 = 289

819 = 3 + 17n – 17

n = 49

Thus 49^th term of this given A.P will be 289 more than its 32^nd term.

 

Q.8 Find the first three terms of an A.P, if sum of 12^th and 15^th term is 219 and sum of 5^th and 7^th term is 114.

Sol.

Since, a_n = a + (n-1)d

Therefore, according to the given conditions:

a₁₂+ a₁₅ = 219

[a + (12-1)d] + [a + (15-1)d] = 219

2a + 25d = 219 ……………………………………………. (1)

And, a₅ + a₇ = 114

[a +(5-1)d] + [a+(7-1)d] = 114

2a + 10d = 114 ……………………………………………………(2)

Subtracting equation (2) from equation (1) we get

25d – 10d = 105

Therefore, d = 7

Putting values of d in equation (2) we get

2a + 10×7 = 114

Therefore a = 22

Hence 1^st term of this A.P = a = 22

             2^nd term of this A.P = 22+(2-1)×7 = 29

             3^rd term of this A.P = 22+(3-1)×7 = 36

 

Q.9 Nikhil started his work in 2002 at an annual salary of Rs 9000 and each year he received an increment of Rs 300. Find in which year his income will reach Rs 12900?

Sol.

Since, each year Nikhil’s salary is increased by Rs 300 and his starting salary was Rs 9000.

Therefore, this forms an A.P with a = 9000 and d = 300

Given, a_n = 12900 and n = ??

Since, a_n = a +(n-1)d

Therefore, 12900 = 9000 + (n-1)×300

300n = 12900 + 300 – 9000

Therefore n = 14

Hence, the salary of Nikhil will be 12900 Rs after 14 years from 2002.

 

Q.10 Find the first three terms of an A.P, if sum of 11^th and 14^th term is 126 and sum of 4^th and 6^th term is 66.

Sol.

Since, a_n = a + (n-1)d

Therefore, according to the given conditions:

a₁₁+ a₁₄ = 126

[a + (11-1)d] + [a + (14-1)d] = 126

2a + 23d = 126 ……………………………………………. (1)

And, a₄ + a₆ = 66

[a +(4-1)d] + [a+(6-1)d] = 66

2a + 8d = 66 ……………………………………………………(2)

Subtracting equation (2) from equation (1) we get

23d – 8d = 60

Therefore, d = 4

Putting values of d in equation (2) we get

2a + 8×4 = 66

Therefore a = 17

Hence 1^st term of this A.P = a = 17

             2^nd term of this A.P = 17+(2-1)×4 = 21

             3^rd term of this A.P = 17+(3-1)×4 = 25

 

Q.11 In the first week of a particular year Deepak saved Rs 10 and then he increased his weekly savings by 2.25Rs. If Deepak was able to increase his weekly savings to Rs 46 in n^th week. Find n

Sol.

Money saved by Deepak in 1^st week = 10Rs

Money saved by Deepak in 2^nd week = 10 + 2.25 = 12.25Rs

Money saved by Deepak in 3^rd week = 12.25 + 2.25 =14.50Rs

Therefore, the above given situation forms an A.P: 10, 12.25, 14.50, 16.75…………..

Here, a = 10

d = 2.25

a_n = 46

Since,       a_n= a+(n-1)d

Therefore 46= 10+(n-1)2.25

2.25n=46-10+2.25

Therefore n= 17

Hence in 17^th week if that year Deepak was able to increase his weekly savings to Rs 46.

 

Q.12 Find 26^th term from the last term of A.P: 7, 12, 17,22………..187.

Sol.

Here, a = 187 (In this case, last term will be first term)

d= -5 (since the given A.P is reversed, therefore d = 12 -17 = 7 – 12 = -5)

And, n = 26

Since, a_n= a + (n – 1)d

Therefore a_n = 187 + (26 – 1)×-5

                   a_n= 62

Therefore 26^th term from the last term is 62.

 

Q.13 Consider two A.Ps :- 9, 15, 21, 27,…….. and 63,66, 69, 72,…….. Find the value of n, such that the n^th term of both the A.P’s is equal.

Sol.

For First A.P, a = 7 and d = 16-7 = 8

Since          a_n = a+(n-1)d

Therefore a_n= 9+(n-1)6

(Or)             a_n= 6n+3

For second A.P, a = 9 and d = 15-9 = 6

Since,          a_n = a + (n – 1)d

Therefore,  a_n = 63 + (n – 1)×3

(Or)              a_n = 3n +60

Now, according to the given condition:

3n+60=6n+3

Therefore n = 19

Therefore, 19^th term of both the A.P’s is equal.

Q.14 Find how many three digit numbers are divisible by 9

Sol.

First 3- digit number divisible by 9 = 108

Second 3- digit number divisible by 9 = 117

Similarly last 3-digit number divisible by 9 = 999

Hence it forms an A.P with d = 9,

108, 117, 126……………999

Now, from the above A.P:

a= 108, d= 9, a_n= 999

n=??

Since, a_n= a+ (n-1)×d

Therefore 999= 108+ (n-1)×9

9n=999-108+9

Therefore n= 900

Hence, there are 900 three digit numbers that are divisible by 9.

 

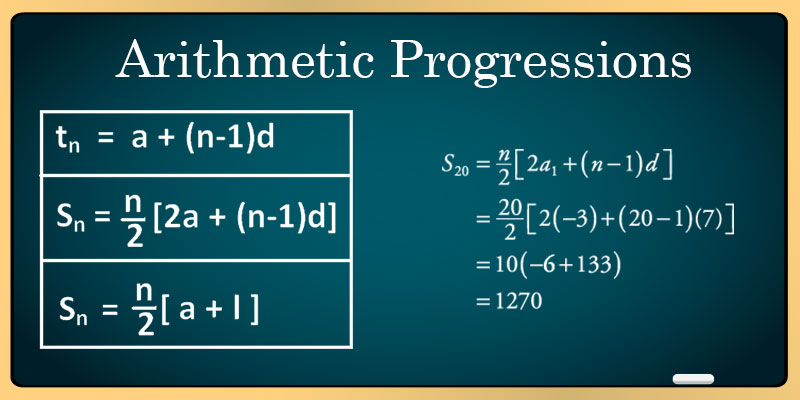
 Formula for Sum of first n terms:

Let us suppose that there are n terms in an A.P, therefore sum of first n terms of an A.P is given by S_n = n2 [2a+(n-1)d]

Here, a = first term, d = common difference and n = number of terms whose sum is to be determined.

REMEMBER:     a_n = S_n – S_n–1

Example-1: In an A.P -2, 5, 12, 19,……… Find the sum of first 8 terms.

Sol.

Here, a = -2 and   d = (12-5) = 7 and n = 8

Since,        S_n = n2 [2a+(n-1)d]

Therefore S_n = 82 [2×-2+(8-1)7]

= 4 × [45]

Therefore sum of first 8 terms = 180.

 

Example – 2 How many terms of an A.P 34, 43, 52, 61,………………… must be taken so that there sum is equal to 1002.

Sol.

Given,  a = 34, d = 9 and S_n = 1002

Since, S_n = n2 [2a+(n-1)d]

Therefore, S_n = 1002 = n2 [2×34+(n-1)×9]

1002×2 = n×[59 + 9n]

9n² + 59n – 2004 = 0

Now, from the above quadratic equation:    a = 9, b = 59 and c = -2004

Substituting the values of a, b and c in quadratic formulae we get:

n=−(59)+(59)2−4(9×−2004)√2×9  and  n= −(59)−(59)2−4(9×−2004)√2×9

n=−59+3481+72144√18  and  n=−59−3481+72144√18

n=−59+75625√18  and  n=−59−75625√18

n = −59+27518  and  n= −59+27518

                  n=12     and     n= 12

Therefore, the sum of first 12 terms in the given A.P will be 2004.

 

Example-3 Find the sum of first 100 odd positive integers.

Sol.

Odd positive integers can be written as: 3, 5, 7, 9, 11……

Here a = 3, d = 2 and n = 100

Since          S_n = n2 [2a+(n-1)d]

Therefore, S_n = 1002 [2×3+(100-1)×2]

= 50[6+198]

= 10200

Therefore the sum of first 100 odd positive integers = 10200

 

Example – 4 The n^th term of any particular series in given by (3n – 5). Find sum of first 16 terms.

Sol.

Given,             a_n = 3n – 5

Therefore,      a₁ = 3×1 – 5 = -2

a₂ = 3×2 – 5 = 1

a₃ = 3×3 – 5 = 4

Therefore, the series becomes -2, 1, 4 . . . . .

Since   [1 – (-2)] = (4 – 1) = 3 = d

Hence, the above series forms an A.P with a = -2 and d = 3

Since, the sum of first n terms is given by: S_n = n2 [2a+(n-1)d]

S_n = 162 [2×-2+(16-1)×3]

= 8(-4 + 45)

= 328

Therefore the sum of first 16 terms is 328

                           Exercise 5.3

Q1. Find the sum of the following given AP’s:

(i) 3, 7, 11, 15 . . . . . . .  up to 12 terms.

Sol.

Here a = 3, d = (7 – 3) = 4 and n = 12

Since, the sum of first 12 terms is given by:  S_n = n2 [2a+(n-1)d]

Therefore,                                            S_n = 122 [2×3+(12-1)×4]

= 6×(6 + 44) = 300

Therefore, the sum of first 12 terms is 300.

(ii) –17, –30, –43. . . . . . . . up to 16 terms.

Sol.

Here a = -17, d = [-30 – (-17)] = -13 and n = 16

Since, the sum of first n terms is given by:  S_n = n2 [2a+(n-1)d]

Therefore,                                                          S_n = 162 [2×(-17) + (16-1)× -13]

= 8×(-34 – 195) = -1832

Therefore, the sum of first 16 terms is -1832

(iii) 0.8, 1.3, 1.8. . . . . . . up to 50 terms.

Sol.

Here a = 0.8, d = (1.3 – 0.8) = 0.5 and n = 50

Since, the sum of first n terms is given by: S_n = n2 [2a+(n-1)d]

Therefore,        S_n = 502 [2×0.8+(50 – 1)×0.5]

= 25×(1.6 + 24.5) = 652.5

Therefore, the sum of first 50 terms is 652.5

 

Q.2 The first term of an A.P is 11 and last term of an A.P is 91. If sum of this A.P is 867, find the total number of terms in that A.P and common difference.

Sol.

Given, a = 11, l = 91 (last term) and S_n = 867

Since,              S_n = n2 [2a+(n-1)d]

(or)                  S_n = n2 [a+a+(n-1)d]

(or)                  S_n = n2 [a + l]       (since a_n = a +(n – 1)d = l)

Therefore,  867 = n2 [11+91]

867×2 = 102n

Therefore,    n = 17

Since              l = a + (17 – 1)d        (since there are total 17 terms)

Therefore, 91 = 11 + 16d

                      d = 5

Therefore, total numbers of terms are 17 and common difference is 5

 

Q.3 First term of an A.P is 17 and last term of an A.P is 407. How many terms are there in an A.P also find its sum if common difference is 13.

Sol.

Given, a = 17, d = 13, l = 407 (last term) = a_n

Since,            a_n = a + (n – 1)d

Therefore, 407 = 17 + (n – 1)×13

13n = 407 + 13 – 17

Therefore, n = 31

Since               S_n = n2 [2a + (n – 1)d]

(or)                  S_n = 312 [a+a+(n – 1)d]

(or)                  S_n = 312 [a + l]       (since a_n = a +(n – 1)d = l)

Therefore,     S_n = 312 [17+407]

S_n = 6572

Therefore, total number of terms in the given A.P are 31 and there sum is 6572.

Q4. 2^nd and 4^th terms of an A.P are 30 and 44 respectively. Find the sum of first 45 terms.

Sol.

Given, a₂ = 30 and a₄ = 44

Since,  a_n = a + (n – 1)d

Therefore, a₂ = a + (2-1)d

(or)             30 = a + d . . . . . . . . . . . (1)

And,     a₄ = a + (4 – 1)d

44 = a + 3d . . . . . . . . . (2)

Subtracting equation (1) from equation (2) we get

14 = 2d

Therefore d = 7, on putting value of d in equation (2) we get

44 = a + 3 × 7

Therefore a = 23

Since, the sum of first n terms is given by: S_n = n2 [2a+(n-1)d]

Therefore,    for n = 45

S_n = 452[2×23+(45 – 1)×7]

S_n = 452[354]

Therefore, S_n = 7965

Hence, the sum of first 45 terms is 7965

 

Q.5 If the sum of first n terms of any particular A.P is given by 2n² – 6n. Find first five terms.

Sol.

Given,             S_n = 2n² – 6n

Therefore,     S₁ = -4     (2×1² – 6×1)

S₂ = -4     (2×2² – 6×2)

Similarly,       S₃ = 0, S₄ = 8, S₅ =20

Since             a_n = S_n – S_{n –1}

Therefore,   a₁ = S₁ = -4

a₂ = S₂ – S₁ = -4 – (-4) = 0

a₃ = S₃ – S₂ = 0 – (-4) = 4

a₄ = S₄ – S₃ = 8 – 0 = 8

a₅ = S₅ – S₆ = 20-8 = 12

Therefore, first five terms of an A.P are:  -4, 0, 4, 8, 12

 

Q.6 A total sum of Rs 7500 is to be used to give 6 cash prizes to students of Saint Marry School for their overall excellent academic performance. If cost of each prize is Rs 200 less than its preceding prize, find the value of each of the prizes.

Sol.

Let the cost of 1^st prize be = a

Given, d = -200 (since each prize is Rs 200 less than its preceding prize)

and    S_n = 7500

Since, the sum of first n terms is given by:  S_n = n2 [2a+(n-1)d]

Therefore,    for n = 6

7500 = 62[2×a + (6 – 1)×-200]

7500 = 3(2a – 1000)

2500 = 2a – 1000

a = 1750

Therefore, cost of prizes will be:

1^st prize = a = 1750Rs,

2^nd prize = (a – d) = 1550Rs,

Similarly, 3^rd prize = 1350Rs, 4^th prize = 1150Rs, 5^th prize = 950Rs and 5^th prize = 750Rs

Q.7 In a construction contract there is a penalty for delay in completion of a contract beyond a certain date. For the delay of one day it is Rs 500, for 2^nd day it is Rs 600, and Rs 700 for the 3rd day and so on, If the penalty for each succeeding day is increased by Rs 100 and the job was delayed for 28 days. Find how much money the contractor has to pay as penalty??

 

Sol.

Given, a = 500 (since, penalty on 1^st day is 500 Rs)

d = 100 (since, penalty increases by Rs 100 for each succeeding day.)

And     n = 28

Since, the sum of first n terms is given by:  S_n = n2 [2a+(n-1)d]

Therefore,    for n = 6

S_n = 282[2×500 + (28 – 1)×100]

= 14(3700)

= 51800 Rs

Therefore, the contractor paid Rs 51800 as penalty.

Q.8 Fauzia took part in a sports event; there was a race in which 8 balloons were placed in a straight line. A basket was placed at a distance of 5m from the first balloon and all 8 balloons are 2m apart. Fauzia started from basket, picks up the nearest balloon and runs back to the basket to drop it and she continues in the same way until all balloons are in the basket. Find the total distance Fauzia covered.  

Sol.

To pick up 1^st balloon and drop it into basket Fauzia covered distance = 2×5m = 10m

Similarly for 2^nd balloon, distance covered by Fauzia = 2(5+2) = 14m

Similarly for 3^rd balloon, distance covered by Fauzia = 2(5+2+2) = 18m

Since there are 8 balloons therefore, n = 8

Here, a = 10 and d = (18-14) = 4

Since, the sum of first n terms is given by: S_n = n2 [2a+(n-1)d]

Therefore,    for n = 8

S_n = 82[2×10 + (8 – 1)×4]

= 4(48)

= 192m

Therefore, total distance covered by Fauzia = 192m

 

Q.9 The product of three numbers in an A.P is -10 and their sum is 6. Find the numbers.

Sol.

Let the numbers be (a – d), a, (a + d)

Now, according to the given conditions:

(a – d) + a + (a + d) = 6

3a = 6, therefore a = 2

And,    (a – d)× a ×(a + d) = -10

(2 – d)(2)(2 + d) = -10

4 – d² = -5

d² = 9

Therefore d = 3 and d = -3

Now, if d = 3

Then the numbers are: [ -1, 2, 5 ]

And, if d = -3

Then the numbers are: [ 5, 2, -1]

 

Extra Questions

LAQ

QUESTIONS-:

1. In an A.P., given pn=4,q=2,An=−14,, find ‘n’ and p.

Ans.- Given-: pn=4,q=2,An=−14,

As we know that, pn = p + (n-1)q

∴ p + (n-1)2 + 4

⇒ p + 2n – 2 = 4

⇒ p + 2n = 4 + 2

⇒ p +2n = 6 ……(i)

Also, we know that  An = n2[2p+(n−1)q]

∴n2[2p+(n−1)2]=−14

 

⇒n[2p+(n−1)2]=−14×2

 

⇒n[2p+2n−2]=−28

 

⇒n[p+(p+2n)−2]=−28

 

⇒n[p+6−2]=−28…[Using(i)]

 

⇒n[p+4]=−28

 

⇒n=−28p+4…(ii)

Now, putting the value of n = n=−28p+4 in equation (i),

We get

p+2(−28p+4)=6

 

⇒p−56p+4=6

 

⇒p(p+4)−56p+4=6

 

⇒p2+4p−56=6(p+4)

 

⇒p2+4p−56=6p+24

 

⇒p2+4p−56−6p−24=0

 

⇒p2−2p−80=0

 

⇒p2−10p+8p−80=0

 

⇒p(p−10)+8(p−10)=0

 

⇒(p−10)(p+8)=0

 

∴p=10,orp=−8

Now, putting the value of p = 10 in (i), we have

10 + 2n = 6

⇒2n=−4

⇒n=−2, which is not possible

Now putting the value of p = -8 in (i), we get

-8 + 2n = 6

⇒2n=14 ⇒n=7

Hence, p = -8 and n = 7

 

2. A contract on construction job specifies a penalty for delay of completion beyond a certain date as follows -: Rs 100 for the first day, Rs 150 for the second day, Rs 400 for the third day, etc.; the penalty for each succeeding day being Rs 50 more than for the preceding day. How much does a delay of 30 days cost the contractor?

Ans.- Since the penalty for each succeeding day is Rs 50 more than for the preceding day. Therefore, the amount of penalty for different days form an A.P. with first term p (= 100) and the common difference q (= 50). We have to find how much a delay of 30 days costs the contractor. In other words, we have to find the sum of 30 terms of the A.P.

∴Requiredsum=3022×100+(30−1)×5 [ becauseAn=n2[2p+(n−1)q] ⇒Requiredsum=15(200+29×50)

 

⇒Requiredsum=15(200+1450) ⇒Requiredsum=15×1650=24750

Thus, a delay of 30 days will cost the contractor of Rs. 24750.

 

3. A sum of Rs. 560 is to be used to give seven cash prizes to students of a school for their overall academic performance. If each prize is Rs. 20 less than its preceding prize, find out the value of each of the prizes.

Ans.- Total amount of seven prizes = Rs.560

Let us assume the value of first prize be Rs.p

By the given condition, we have

P, p-20, p-40,…

Now, m2−m1=p−20−p=−20

Similarly,m3−m2=p−40−p+20=−20 Sincem2−m1=m3−m2=−20,whichisconstant.

Therefore, it is an A.P. with common difference (q) = -20

Let K = p + (p-20) + (p-40) + … upto 7 terms

Here, m = p, q =-20 and n = 7

Now,  Kn=n2[2m+(n−1)q]

∴K7=72[2p+(7−1)(−20)]

 

⇒K7=72[2p+(6)(−20)]

 

⇒560=72[2p−120]

 

[∴Totalamountof7prizes=Rs.560=K7]

 

⇒2p−120=560×27

 

⇒2(p−60)=160

 

⇒p−60=1602=80

 

⇒p=80+60=140

Hence, the amount of each prize (in rs.) respectively is-:

140, 140-20, 140-40, 140-60, 140-80, 140-100, 140-120

i.e, Rs.140, Rs.120, Rs.100, Rs.80, Rs.60, Rs.40, Rs.20 respectively.

 

4. A sum of Rs. 1000 is to be used to give ten cash prizes to students of a school for their overall academic performance. If each prize is Rs. 20 less than its preceding prize, then find out the value of each of the prizes.

Ans.- Here, the total amount of ten prizes is = Rs.1000

Now, Let us assume the value of first prize be RS. P

So, by the given condition, we have prizes of denomination (in Rs.) p, p – 20, p – 40, …

Now, m2−m1=p−20−p=−20

m3−m2=p−40−p+20=−20

Since, m2−m1=m3−m2=−20, which is constant.

Therefore, it is an A.P. with common difference (q) = -20

Hence, A10=p+(p−20)+(p−40)+….upto10terms

Here, m = p, q = -20 and n = 10

Now, An=n2[2m+(n−1)q][ becauseA10=1000]

⇒1000=5(2p−180) ⇒1000=10p−900 ⇒10p=1900 ⇒p=190

 

Hence, amount of each prize (in Rs.) respectively is 190, (190-20), (190-40), (190-60), (190-80), (190-100), (190-120), (190-140), (190-160) and  (190-180)

i.e., Rs.190, Rs.170, Rs.150, Rs.130, Rs.110, Rs.90, Rs.70, Rs.50, Rs.30 and Rs.10.

 

5. A spiral is made-up of successive semicircles, with centres alternately at P and Q, starting with centre at P, of radii 0.5 cm, 0.1 cm, 1.5 cm, 2.0 cm, … as shown in fig. What is the total length of such a spiral made-up of 13 consecutive semicircles ? (Takeπ=227)

Ans.- Since radii od semicircles are 0.5 cm, 1.0 cm, 1.5 cm, 2.0 cm …

Now, the length of semicircle (l1) = Perimeter  of first semicircle = πr=227×0.5cm

=227×510=117cm

So now, the length of the second semicircle(l2) = Perimeter of second semicircle

= πr=227×1.0cm=227cm

Similarly, we can get the length of other semicircle as,

l3=337cmandl4=447cm and so on upto 13 semicircles.

Let us assume ‘A’ be the total length of all semicircles.

i.e., p= l3=337cmandl4=447cm … upto 13 terms

Here, p=117,q=227−117=117andn=13

Since, An=n2[2p+(n−1)q]

∴A13=132[2×117+(13−1)×117] =132[227+1327] =132×1547=143cm

 

 

6. 200 logs are stacked in the following manner : 20 logs in the bottom row, 19 in the next row, 18 in the row next to it and so on. Find out in how many rows are 200 logs placed and how many logs are in the top row ?

Ans.- Suppose 200 logs are stacked in ‘s’ rows.

There are 20 logs in the first row and the number of logs in a row is one less than the number of logs in the preceding row. So, number of logs in various rows form an A.P. with first term p (=20) and the common difference q (= -1). As there are 200 logs in all rows.

∴ Sum of ‘s’ terms of an A.P. with p = 20 and q = -1 is 200

⇒s2{2p+(s−1)q}=200 ⇒s2{2×20+(s−1)×−1}=200 ⇒s2(40−s+1)=200 ⇒n(41−s)=400 ⇒s2−41s+400=0⇒(s−25)(s−16)=0 ⇒s=16ors=25

Now, If s =25, then number of logs in 25^th row is equal to 25^th terms of an A.P. with first term 20 and the common difference (-1).

∴ number of logs in 25^th row = p + 24q = 20 – 24 = -4

Clearly, this is not meaningful.

∴ s = 16

Thus, logs are placed in 16 rows.

Number of logs in top row = Number of logs in 16^th row

= 16^th term of an A.P. with p = 20 and q = -1

Hence, there are 5 logs in the top row.

 

7. The sum of 4^thand 8^th terms of an A.P. is 24 and the sum of 6^th and 10^th terms is 44. Calculate A.P.

Ans.Let us assume ‘p’  be the first term and ‘q’  be the common difference of the required A.P.

∴A4+A8=24

 

⇒42{2p+(4−1)q}+82{2p+(8−1)q}=24

 

⇒2{2p+3q}+4{2p+7q}=24

 

⇒4p+6q+8p+28q=24⇒12p+34q=24

Or, 6p + 17q = 12 ….(i)

And, A6+A10=44

⇒62{2p+(6−1)q}+102{2p+(10−1)q}=44

 

⇒3{2p+5q}+5{2p+9q}=44

 

⇒6p+15q+10p+45q=44⇒16p+60q=44

Or, 4p+15q = 11 ….(ii)

Now, multiplying (i) by 2 and (ii) by 3, we get

12p + 34q = 24 …..(iii)

12p + 45q = 33 ……(iv)

Now, Subracting (iii) from (iv), we have 11q = 9 or q = 911

From (ii), we have 4p+15×911=11

⇒4p=11−13511=121−13511=−1411 ⇒p=−1411×14=−722

Hence, the required A.P. is -:

−722,−722+911,−722+1811, ….

Or, −722,12,2922, ….

 

8. The 17^thterm of an A.P. is 5 more than twice its 8^th If the 11^th term of the A.P. is 43, then calculate its ‘nth’ term.

Ans.- Given, p11=43

P + 10q = 43 ….(i)

[Usingpn=p+(n−1)q]

And, p17=5+2p8

P + 16q = 5 + 2[p + 7q]

[Usingpn=p+(n−1)q]

P + 16q = 5 + 2p + 14q

⇒ p – 2q = -5     ….(ii)

Subtracting (ii) from (i), we get

p+10q – (p-2q) = 43 – (-5)

p+10q-p+2q = 43+5    ⇒12q=48⇒q=4 …(iii)

From (iii) and (i), we have

P + 40 = 43   ⇒p=3

We know that, pn=p+(n−1)q

pn=3+(n−1)4=3+4n−4=4n−1

Hence, the ‘nth’ term of given A.P. is 4n – 1.

 

9. Determine the common difference of an A.P. whose first term is 5 and the sum of it first four term is half the sum of the next four terms.

Ans.- Given, First term = p = 5

And, A4=12(thesumofp5,p6,p7,p8)

= 12(A8−A4)⇒2A4=A8−A4⇒3A4=A8

A4=42[2p+(4−1)q] [UsingAn=n2(2p+(n−1)q)]

= 2 (10 + 3q) = 20 + 6q

A8=82(10+7q)=4(10+7q)=40+28q

So, 3A4=3(20+6q)=60+18q

3A4=A8   (According to question)

60 + 18q = 40 + 28q

⇒10q=20⇒q=2

∴ The common difference (q) = 2.

 

10. The sum of first six terms of an A.P. is 42. The ratio of its 10^thterm to its 30^th term is 1 : 3. Determine the first and the 13^th term of the A.P.

Ans.- Let us assume ‘p’ be the first tern and ‘q’ be the common difference.

Here, A6=42

62{2p+(6−1)q}=42

2p+5q = 14 …..(i)

Also, p10p30=13

⇒p+9qp+29q=13 ⇒3p+27q=p+29q ⇒3p−p=29q−27q

⇒2p=2q …..(ii)

Subtracting (ii) from (i), we get

5q + 2q = 14

7q = 14

q = 2

Now, from (ii), we have -:

2p−2×2=0⇒p=2

So now,  p13=p+12q=2+12×2⇒2+24=26

Therefore, the first term of the given A.P. is 2 and its 13^th term is 26.

 

11. If the sum of first ‘s’ terms of an A.P. is ‘n’ and the sum of first n terms is ‘s’, then prove that the sum of its first (s+n) terms is –(s+n).

Ans.- Let us assume ‘p’ be the first term and ‘q’ be the common difference

∴As=n ⇒s2[2p+(s−1)q]=n

⇒2p+(s−1)q=2ns ….(i)

And, An=s

⇒n2[2p+(n−1)q]=s

⇒2p+(n−1)q=2sn ….(ii)

Subtracting (ii) from (i), we get,

(s – 1 – n + 1)q = 2ns−2sn

(s – n)q = 2(n2−s2)sn = 2(n+s)(n−s)sn

⇒q=−2(s+n)sn …..(iii)

Also, from (i), we get -: 2p=2ns−(s−1)q …..(iv)

And now, the sum of first (s + n) terms

= s+n2[2p+(s+n−1)q]

= s+n2[2ns−(s−1)q+(s+n−1)q] [Using (iv)]

= s+n2[2ns+nq]=n(s+n)2[2s+q]

= n(s+n2)[2s−2(s+n)sn] [Using(iii)]

= n(s+n2)[2n−2s−2nsn]

= n(s+n2)[−2ssn]=−(s+n)

 

12. In an A.P., the sum of first terms is given by -:

An=3n22+5n2. Determine the 25^th term of the A.P.

Ans.- Here, An=3n22+5n2=3n2+5n2

⇒An−1=3(n−1)2+5(n−1)2 ⇒3n2+3−6n+5n−52 ⇒3n2−n−22

Now,

pn=An−An−1

 

=3n2+5n2−3n2−n−22

 

=5n+n+22=6n+22=2(3n+1)2=3n+1

 

pn=3n+1

 

∴p1=3×1+1=4

 

∴p2=3×2+1=7

 

∴p3=3×3+1=10

Hence, the common difference (q) = 7 – 4 = 3

∴p2=3×2+1=7

 

13. A manufacturer of T.V. sets produced 600 sets in the third year and 700 sets in the seventh year. Assuming that the production increase uniformly by a fixed number of every year, calculate-:

(i) The production in the 1^st year.

(ii) The production in the 10^thyear.

(iii) The total production in first 7years.

Ans.- Let us assume the number of sets produced in 1^st year be ‘x’ and ‘y’ be the increase in population every year.

We are given, x + 2y = 600 …..(i)

And, x + 6y = 700 …..(ii)

Now subtracting equation (i) from (ii), we get

4y = 100 or y = 25

Now, Substituting y = 25 in equation (i), we get

x = 550

(1)        Production in the first year = x = 550

(2)        Production in 10th year = x+9y = 550+(9)(25) = 550 + 225 = 775

(3)        Total production in first 7years = x + (x+y) +(x+2y) + …..+ (x+6y)

We know that,

Sum = n2[2x+(n−1)y]

Here, x = 550, y = 25, n = 7

∴Sum=n2[2x+(n−1)y]

 

= 72[2×550+(7−1)(25)]

= 72[1100+(6)(25)]

= 72[1100+(6)(25)]

= 4375

∴ Total production in first 7 years = 4375

 

SAQ 2 MARK

QUESTIONS-:

 

1. The amount of money in the account every year, when rs 5000 is deposited at compound interest at 5% per annum.

Ans.-  Here, Principal = Rs.5000

Rate of interest = 5% per annum

Therefore, Annual (A) = 5000(1+5100)

Now, the sequence becomes  5000(1+5100),

5000(1+5100)2,5000(1+5100)3,…

Here, a2−a1≠a3−a2

Therefore, the given amount of money in the account every year does not form an A.P.

 

2. In which of the following situations, does the list of numbers involved make an arithmetic progression and why ?

(i) The amount of air present in a cylinder when a vaccum pump removes 16 of air remaining in the cylinder at a time.

(ii) The cost of digging a well after every metre of digging, when it costs Rs.250 for the first metre and rises by Rs. 100 for each subsequent metre.

Ans.- Let us assume, x1= p units

∴x2=p−16p=56punits ∴x3=56p−16×56p=56p−536p=2536punits

Similarly, ∴x4=2536p−16×2536p=2536p−25216p=125216punits

So, the list of numbers is p, 56p,2536p,125216p,…

Now here, x2−x1≠x3−x2

Hence, it is not an A.P.

 

3. Write first four terms of the A.P. whose first term is 20 and common difference is 20.

Ans.- In this case we have, p= 20, q= 20

∴Firstterm(p1)=p=20

 

Secondterm(p2)=p1+q=20+20=40

 

Similarly,Thirdterm(p3)=p2+q=40+20=60

 

Now,Fourthterm(p4)=p3+q=60+20=80

Hence, the required four terms are 20, 40, 60, 80.

 

4.Write the first four terms of the A.P. whose first term is -1 and the common difference is 12.

Ans.-  Here we have, p=-1, q=12

So, Firstterm(p1)=p=−1

Secondterm(p2)=p1+q=−1+12=−12

 

Similarly,Thirdterm(p3)=p2+q=−12+12=0

 

Similarly,Fourthterm(p4)=p3+q=0+12=12

Hence, the required four terms are −1,−12,0,12.

 

5. Write four terms of the A.P. whose first term is -1.25 and the common difference is -0.25.

Ans.- Here we have, p=-1.25, q=-0.25

So, Firstterm(p1)=p=−1.25

Secondterm(p2)=p1+q=−1.25=(−0.25)=−1.25−0.25=−1.50

 

Similarly,Thirdterm(p3)=p2+q=−1.50+(−0.25)=−1.50−0.25=−1.75

 

Similarly,Fourthterm(p4)=p3+q=−1.75+(−0.25)=−1.75−0.25=−2.0

Hence, the required four terms are -1.25, -1.50, -1.75, -2.0

 

6. Write first four terms of the A.P. when the first term ‘p’ and the common difference ‘q’ are given as follows-:

(i) p = -2, q = 0   (ii) p = 4, q = -3

Ans.- (i)Here,  First term = p = -2

Second term = p+q = -2+0 = -2

Third term = p+2q = -2+2(0) =-2

Similarly, Fourth term = p+3q = -2+3(0) =-2

Hence, the required four terms are -2, -2, -2, -2.

(ii) We have, First term = p = 4

Second term =p+q =4+(-3)=1

Third term = p+2q = 4+2(-3)= -2

Similarly, Fourth term = p+3q= 4+3(-3)= -5

Hence, the required four terms are 4, 1, -2, -5.

 

7. For the following A.P., write the first term and the common difference of the following 3, 1, -1, -3, …

Ans.- Here A.P. is 3, 1, -1, -3, …

So, the first term p1= 3

Now, p2−p1=1−3=−2,

p3−p2=−1−1=−2, p4−p3=−3−(−1)=−3+1=−2

i.e., pn+1−pnissameeverytime.

So therefore, for the given A.P. common difference (q) = -2

 

8. For the following A.P., write the first term (p1) and the common difference (q) 13,53,93,133,…

Ans.- Here the given A.P. is  13,53,93,133,…

So, First term ((p1))= 13

Now, p2−p1=53−13=5−13=43

p3−p2=93−53=9−53=43 p4−p3=133−93=13−93=43

i.e. , pn+1−pnissameeverytime.

So therefore, for the given A.P. common difference(q)=43

 

9. For the following A.P.s, write the first term (p) and the common difference (q)-:

(i) -5, -1, 3, 7, …  (ii) 0.6, 1.7, 2.8, 3.9, ….

Ans.- Given here, First term (p) = -5

Now the common difference (q) = -1-(-5) = 3-(-1) = 7-3 = 4

∴ Thus, for the given A.P., first term (p) is -5 and the common difference (q) is 4.

(ii) Given here, First term (p) = 0.6

Similarly, the common difference (q) = 1.7 – 0.6 = 2.8 – 1.7 = 3.9 – 2.8 = 1.1

∴ Thus, for the given A.P., first term (p) is 0.6 and the common difference (q) is 1.1.

 

10. Find whether the following list of numbers form an A.P If they form an A.P., find the common difference (q) and write three more terms p,p2,p3,p4,…

Ans.-  Here the given list of numbers is p,p2,p3,p4,…

So, first term (p1) = p

Now, p2−p1=p2−p=p(p−1)

p3−p2=p3−p2=p2(p−1) p4−p3=p4−p3=p3(p−1)

As, p2−p1≠p3−p2

Hence,  therefore the given list of numbers does not form an A.P.

 

11. Find whether the following list of numbers form an A.P. If they forman A.P., then find the common difference (q) and write three more terms 3, 3+2–√, 3+22–√, 3+32–√,…

Ans.- Here the given list of numbers is -: 3, 3+2–√, 3+22–√, 3+32–√,…

Now, first term (p1) = 3

So, p2−p1=3+2–√−3=2–√

p3−p2=3+22–√−(3+2–√)

= =3+22–√−3−2–√=2–√

Similarly,

p4−p3=3+32–√−(3+22–√)

= p4−p3=3+32–√−3−22–√=22–√

So here it is concluded that, pn+1−pnissameeverytime.

Hence, the given list of numbers form an A.P. in which, q = 2–√

Now the next three more terms are -:

3+32–√+2–√=3+42–√ 3+42–√+2–√=3+52–√

And , 3+52–√+2–√=3+62–√

∴ Hence, the three more terms are

3+42–√,3+52–√and3+62–√

 

12. Find whether the following list of numbers form an A.P. If they form an A.P., find the common difference (q) and write three more terms 2–√,8–√,18−−√,32−−√,…

Ans.- Here the given list of numbers is -:  2–√,8–√,18−−√,32−−√,…

Now here, the first term (p1)=2–√

So, p2−p1=8–√−2–√=4×2−−−−√−2–√

= 22–√−2–√=2–√

 

p3−p2=18−−√−8–√=9×2−−−−√−4×2−−−−√

=  32–√−22–√=2–√

p4−p3=32−−√−18−−√=16×2−−−−−√−9×2−−−−√

= 42–√−32–√=2–√

So here it is concluded that, pn+1−pnissameeverytime.

Hence, the given list of numbers form an A.P. in which, q = 2–√

Now the next three more terms are -:

32−−√+2–√=16×2−−−−−√+2–√=42–√+2–√

= 52–√=25×2−−−−−√=50−−√

50−−√+2–√=25×2−−−−−√+2–√=52–√+2–√

= 62–√=36×2−−−−−√=72−−√

72−−√+2–√=36×2−−−−−√+2–√=62–√+2–√

= 72–√=49×2−−−−−√=98−−√

∴ Hence, the three more terms are -:

50−−√,72−−√and98−−√

 

13. Find whether the following list of numbers form an A.P. If they form an A.P., find the common difference(q) and write three more terms -: 12,52,72,73,…

Ans.- Given list of numbers is-: 1,25,49,73,…

Here, the first term (p1)=1

Now, p2−p1=25−1=24

p3−p2=49−25=24

Similarly,  p4−p3=73−49=24

So here it is concluded that, pn+1−pnissameeverytime.

Hence, the given list of numbers form an A.P. in which, q = 24

Now the next three more terms are -: 73+24 = 97

97+24 = 121

And , 121+24 = 145

Hence, the three more terms are 97, 121 and 145.

 

14. Fill in the blanks p = 15, q = 9, n = 12, pn = …

Here, ‘p’ is the first term, ‘q’ is the common difference and pn  = nnt term of an A.P.

Ans.- Given, p = 15, q = 9, n = 12, pn = ?

As we know that,

pn = p+(n−1)q

p12 = 15 + (12-1)9

= 15 + 99 = 114

Hence, p12 =114

 

15. Fill in the blanks p = 20, q = 4, n = …, pn =12

Ans.- Here, ‘p’ is the first term,’ q’ is the common difference and pn is the nth term of an A.P.

Given, p = 20, q = 4, pn = 12, n = …..

As we know that-: pn = p + (n – 1)q

⇒ 12 = 20 + (n – 1)4

⇒ 12 = 20 + 4n-4

⇒ 4n = 12 – 20 + 4 = -4

⇒ n = -1

∴Hence,n=−1

 

16. In the following A.P., find the missing term-: 4, ?, 32.

Ans.- Here given A.P. is 4, ?, 32.

Let us assume the missing number be ‘p’

i.e., 4, p, 32 is an A.P.

∴ p – 4 =  32 – p

⇒ p + p = 32 + 4

⇒ 2p = 36

⇒ p = 18

Hence, the missing term is 18.

 

17. How many terms are there in A.P. 7, 16, 25, …, 542 ?

Ans.- Given here, pn=540

P + (n+1)q = 542

⇒7+(n−1)9=542 ⇒9n–2=542 ⇒9n=540 ⇒n=60

Hence, the number of terms is 60.

18. Find the value of s, if the numbers q, 2q + s, 3q +6 are three consecutive terms of an A.P.

Ans.- Here, q, 2q + s, 3q +6 are in A.P.

∴  2q+s-q = 3q+6-2q-s

⇒ q+s = q+6-s

⇒ 2s = 6

⇒ s = 3

 

19. Find the common difference (q) of an A.P. whose first term is 12 and the 8^thterm is 176. Also mention its 4^th

Ans.- Let us assume p8=176

⇒p+7q=176

 

⇒12+7q=176

 

⇒7q=176−12

 

⇒7q=176−12

 

So now, p4=p+3q=12+3(13)=12+1=32

 

20. The angles of a triangle are in A.P. The greatest angle is twice the least. Find all the angles of the triangle.

Ans.- Let us assume the three angles of the triangle be p, q and r, where p is the smallest angle and r is the largest angle.

According to the given condition-: r = 2p ….(i)

Also, p + q + r =180∘ ….(ii)

[ becausesumofanglesofthe:triangleis180∘]

Here, 2q = p + r ….(iii)

⇒ = 2q = p + 2p ….[using (i)]

⇒ = 2q = 3p

⇒q=32p

Now, from (ii), we have -: p+32p+2p=180∘

⇒92p=180∘ ⇒p=180∘×29=40∘ ∴q=32×40∘=60∘ andr=2(40∘)=80∘

Hence, angle of the triangle are 40∘,60∘,80∘respectively.

 

21. Determine the 2^ndterm of an A.P. whose 6^th term is 12 and 8^th term is 22.

Ans.- Here, p6=12⇒p+5q=12…(i)

And,  p8=22⇒p+7q=22…(ii)

Now, subtracting (i) from (ii), we have

2q = 10

⇒ q = 5

Now, from (i), we have

P + 5(5) = 12

⇒ p = 12 – 25

⇒ p = -13

∴p2=p+q=−13+5=−8

 

22. Find the number of all three-digit natural numbers which are divisible by 9.

       108, 117, 126, 135, ….., 999

Ans.- Here, p = 108, q = 9 and pn=999

P + (n – 1)q = pn

108 + (n-1)9 = 999

⇒ (n – 1)9 = 999 – 108

⇒ (n – 1) = 8919=99

⇒ n = 99 + 1 = 100

 

23. The 8^thterm of an A.P. is equal to three times its third term. If its6th term is 22, then find the A.P.

Ans.- Let us assume ‘p’ and ‘q’ be the first term and the common difference of the required A.P.

∴ p6 = p + 5d = 22 ….(i)

And,  p8=3(p2)

⇒p+7q=3(p+2q) ⇒p+7q=3p+6q

⇒q=2p …..(ii)

From the equations (i) and (ii), we have

P + 5(2p) = 22

⇒11p=22 ⇒p=2

Similarly from (ii), we have q = 2(2) = 4

Hence, the required A.P. is 2, 6, 10, 14,….

 

24. Find the 20^thterm from the last term (end) of the A.P. -: 3, 8, 13, ….,253.

Ans.- We have an A.P. -:3, 8, 13, …, 253.

Here, p = 3, last term x = 253 and the common difference q = 5

Now, ‘nth’ term from end = x + (-n + 1)q

We have 20^th term from end as

= 253 + (-20+1)5 = 253 – 95 = 158.

OR

20^TH term from the last term of the given A.P.

= 20^th term of the A.P. 253, 248, 243, ….

= p + 19q

= 253 + 19 (-5)

253 – 95 = 158

 

25. The 9^thterm of an A.P. is equal to 6 times its second term. If its 5^th term is 22, then find the A.P.

Ans.- Let us assume ‘p’ and ‘q’ be the first term and common difference of the required A.P.

Here, p5=22

⇒p+4q=22 …(i)

And,  p9=6p2

⇒p+8q=6(p+q) ⇒p+8q=6p+6q

⇒5p=2q  ….(ii)

From (i) and (ii), we have -:

p + 2(5)p = 22

⇒11p=22 ⇒p=2

From (ii), we have 5(2) = 2q ⇒q=5

Hence, the required A.P. is 2, 7, 12, 17, …

 

26. The sum of the first 15 terms of an A.P. is 750 and its first term is 15. So find its 20^th

Ans.- Here, first term p = 15 and A15=750

An=n2[2p+(n−1)q] ⇒750=152[2×15+(15−1)q] 750=152(30+14q)

750 = 225 + 105q

⇒105q=750–225 ⇒q=525105=5

We have to find 20^th term

So as we know, pn=p+(n−1)q

∴p20=15+(19)5=15+95=110

 

27. The 16^thterm of an A.P. is more than twice its 8^th If the 12^th term of the A.P. is 47, then find out its ‘nth’ term.

Ans.- Here, p12=47…(given)

⇒p+11q=47…(i)

And, p16=1+2p8

⇒p+15q=1+2(p+7q)

So we got, P – q =-1     ……..(ii)

Subracting (ii) from (i), we get

⇒12q=48⇒q=4

Hence, p – 4 = -1  ⇒p=3 ….[using(ii)]

So, pn=3+(n−1)4=4n−1…[Usingpn=p+(n−1)q]

 

28. Sum of the first 20 terms of an A.P. is -240, and its first term is 7. Find out its 24^th

Ans.- Here, p = 7 …..(given)

A20=−240

⇒202(2×7+19q)=−240  ………..UsingAn=n2(2p+(n−1)q)

⇒10(14+19q)=−240 ⇒19q=−24−14⇒q=−2

So now, we have to find the 24^th term of A.P. i.e -:

p24=7+23×−2[Usingpn=p+(n−1)q]

= 7 – 46

= -39

 

Arithmetic progression VSAQ

 

1. Find next term of the A.P 3–√,12−−√,7–√,…

 

Ans.     Here p=3–√,q=12−−√−3–√=23–√−3–√=3–√

 

Next term to

27−−√=27−−√+3–√=33–√+3–√=43–√

=3×16−−−−−√=48−−√

2. In an A.P., 6 times the 6th term is equal to 11 times the 11 term, then find its 17th term

 

Ans.       6x6=11x11

6(x+5y)=11(x+10y)

⇒      6x+30y=11x+11y

⇒   11x−6x=−11y+30y

⇒         11x=−80y

⇒   x=−16y

 

⇒x+16y=0 ⇒x17=0

 

3. If the nth term of an A.P. is (2n =1), then find the sum of its first three terms.

 

Ans.      Here, we have     pn=2n+1

p1=2×1+1=3i p2=2×2+1=5i p3=2×3+1=7i

Adding p1+p2+p3=3+5+7=15

= sum of first three terms

s3=32(3−7)=30215

[ Using Sn=n2(p+l) ]

 

4. Find the sum of first 20 odd natural numbers.

 

Ans.   We know that sum of n odd natural number = n2

 

∴ sum of odd natural numbers =202=400

 

5. If a+1 ,2a+1, 4a+1 are in A.P. , then find the value of a.

  

Ans.   2a + 1 – a – 1 = 4a- 1 – 2a – 1

⇒                 a= 2a -2   ⇒ a=2

6. If p-1 ,p+3 ,3p-1 are in A.P. , then find the value of p.

 

Sol.  p + 3  – p + 1 = 3p – 1 – p – 3

⇒  4 = 2 p – 4

⇒       p=4

 

7. Which term of the A.P. 24,21, 28,…. Is the first negative terms ?

 

Ans.    a_n< 0

a+(n-1 )d < 0

24 +(n-1)(-3)< 0

24-3n +3 < 0

-3n<-27

n>9

 

8. Which term of the A.P. 1,4,7,….is 88?

 

Ans. .let   a_n =88

a+(n-1)d=88

1+(n−1)3=88 ⇒3n−2=88 ⇒3n=90 ⇒n=30

 

9. Which term of the A.P. 92, 88, 84, 80,… is zero.

 

Ans.   let

an=0 a+(n−1)d=0

 

⇒a+(n−1)d=0

 

⇒92+(n−1)(−4)=0

 

⇒96−4n=0

 

⇒4n=96 ⇒n=24

 

10. Whichterm of the A.P. 100, 90, 80,… is zero.

 

Ans. let

an=0 a+(n−1)d=0 ⇒100+(n−1)(−10)=0 ⇒−10n+110=0 ⇒n=0 ⇒n=11

 

11. Find the 15th term of the A.P. x-7,x-2, x-3,….

 

Sol.

a15=a+14d

=x−7+14(5)

=x−7+70

=x+63

 

12. what is the sum of all natural number from 1 to 1oo ?

 

Sol.

sn=n(n+1)2 s100=100(100+1)2

 

=100(101)2

=5050

 

Arithmetic Progressions Long Answer Questions

 

1. Jassi saves $32 during the first month, $36 in the second month and $40 in the second month and $40 in the third month. If she continues to save in this manner, in how many months will she save $2000 ?

Ans.  According to the question we have

32 + 36 + 40 + …n terms = 2000

n2[2×32+(n−1)4]=2000

[ because Here a= 32 ,d= 36 – 32 = 4  and no. of terms be n]

⇒32n+2n2−2n−2000=0 ⇒2n2+30n−2000=0 ⇒n2+15n−1000=0 ⇒n2+40n−25n−1000=0 ⇒(n−25)(n+40)=0

⇒ Either n – 25 =0 or n + 40 = 0

⇒ n =25 or n= -40

n cannot be =-40 ,because no. of terms cannot be –ve

Hence ,Jassi saves $2000 in 25 months.

2. Solve the Equation -4 + (-1) + 2 +… + x= 437

Ans.  In the LHS of the given equation, we have

a = -4 and d = -1 – (-4) = -1+4 =3 and l=x

∴ -4+(-1)+2+… +x =437

⇒n2(−4+x)=437  [ becauseSn=n2(a+l)]

⇒ n(-4+x) =874               …(i)

Also ,     x = -4 +(n-1)d                                               …(ii)

[ becausean=a+(n−1)d]

From (i) and (ii) we have,

n( – 4 – 4 + (n-1)d) = 874

⇒ -8n +n(n-1)3 = 874

⇒ −8n+3n2−3n−874=0

⇒ 3n2−11n−874=0

n=11±(−11)2−4×3×(−874)√2×3 n=11±121+10488√6 =11±1036=11+1036,11−1036

=19,−926 (rejecting)

n = 19

From (ii) , we obtain

x= -4 +(19-1)3

x= -4 + 54

x= 50

3. Yashraj Pal is repaying his total loan of Rs 118000 by paying every month starting with the first installment of Rs 1000.If he increases the installment by Rs 100 every month, what amount will be paid by him in the 30^thinstallment? What will be the amount of loan does he have to pay after 30^th installment?

Ans.  Since Yashraj Pal is repaying his loan of Rs 118000 with first installment of Rs. 1000 and increases each installment by Rs. 100.

∴ his installments are Rs 1000, Rs1100 ,Rs 1200, Rs 1300,… which forms an A.P.

Here, first term is Rs. 1000 and common difference is Rs. 100

∴ 30^th installment = a30=a+29d

=Rs( 1000 + 29 x 100)

=Rs (1000 +2900) = Rs. 3900

Amount paid in 30 installments = S30

⇒S30=Rs.302(2×1000+29×100)

= Rs. 15(2000 + 2900)

= Rs. 15(4900)

= Rs. 73500

Amount of loan that still have to be paid

= Rs. (118000 – 73500)

= Rs. 44500

4. In an A.P., given pn=4,q=2,An=−14,, find ‘n’ and p.

Ans.- Given-: pn=4,q=2,An=−14,

As we know that, pn = p + (n-1)q

∴ p + (n-1)2 + 4

⇒ p + 2n – 2 = 4

⇒ p + 2n = 4 + 2

⇒ p +2n = 6 ……(i)

Also, we know that  An = n2[2p+(n−1)q]

∴n2[2p+(n−1)2]=−14

 

⇒n[2p+(n−1)2]=−14×2

 

⇒n[2p+2n−2]=−28

 

⇒n[p+(p+2n)−2]=−28

 

⇒n[p+6−2]=−28…[Using(i)]

 

⇒n[p+4]=−28

 

⇒n=−28p+4…(ii)

Now, putting the value of n = n=−28p+4 in equation (i),

We get

p+2(−28p+4)=6

 

⇒p−56p+4=6

 

⇒p(p+4)−56p+4=6

 

⇒p2+4p−56=6(p+4)

 

 

⇒p2+4p−56=6p+24

 

⇒p2+4p−56−6p−24=0

 

⇒p2−2p−80=0

 

⇒p2−10p+8p−80=0

 

⇒p(p−10)+8(p−10)=0

 

⇒(p−10)(p+8)=0

 

∴p=10,orp=−8

Now, putting the value of p = 10 in (i), we have

10 + 2n = 6

⇒2n=−4

⇒n=−2, which is not possible

Now putting the value of p = -8 in (i), we get

-8 + 2n = 6

⇒2n=14 ⇒n=7

Hence, p = -8 and n = 7

 

Arithmetic Progressions SAQ 2 Marks

 

1. Two Arithmetic Progressions (AP) have the same common difference. The first term of one AP is two and that of the other is seven. The difference their 10^th terms is the same as the difference between their 21^stterms, which is the same as the difference between any two corresponding terms? Why?

Ans.  In this question, first terms of the AP’s are 2 and 7.

Let Common Difference of the 2 AP’s be d.

∴ Difference between their 10^th terms

= a10−A10

= 7+9d-2-20d

= 7-2 = 5

The difference between their 21^st terms

= a21−A21

= 7+20d-2-20d

= 7-2 = 5

The difference between any two corresponding terms of the AP’s is the same as the difference between their first terms.

2. Find the value of the middle most term(s) of the AP -11, -7, -3,… ,49

Ans. Here , a = -11 , d = -7-(-11) = 4 and an =49

∴ a + (n-1)d = an

-11 + (n-1)4 = 49

⇒ (n-1)4 = 60

⇒ (n-1) = 15

⇒ n=16

As n is even number, there will be two middle terms which are (162)th and (162+1)th i.e. 8^th  and 9^th terms.

∴ a8 =a + 7d = -11 + 7(4) = -11 + 28 = 17

a9 = a + 8d = -11 + 8(4) = -11 + 32 = 21

Hence required two middle most terms are 17 and 21.

3. Determine the AP whose 5^thterm is 19 and the difference of the 8^th term from the 13^th term is 20.

Ans.  Let a be the first term and d be the common difference of given AP

a5 = a + 4d = 19        …(i)

And a13−a8=20

⇒ a + 12d – a – 7d = 20

⇒ 5d = 20 ⇒ d = 4

From(i) we have

a + 4(4) =19

a=19-16 =3

Hence required AP is 3, 7, 11, 15, …  .

 

4. Find whether 55 is a term of the AP 7, 10, 13,… or not. If not find which term it is.

Ans.  Here, a=7 ,d = 13-10 = 3 and an = 55

∴ a + (n-1)d = 55

7 + (n-1)3 =55

(n-1)3 = 48

(n-1) =16

n = 17

Hence ,55 is the 17^th term of the given AP

 

5. Split 207 into three parts such that these are in AP and the product of the two smaller part is 4623.

Ans. Let the 3 parts of 207 be a-d ,a and a+d

∴ a – d + a + a + d = 207

3a = 207

a = 2073

a = 69

Also , (a-d)a =4623

⇒ (69-d)69 =4623

⇒ 69-d =67

⇒ d = 69-67 = 2

Therefore ,the 3 parts of 207 are 69-2, 69, 69+2

i.e. 67 , 69 and 71.

Related Links