Polynomials with degree 2 are called quadratic polynomials. When this polynomial is equated to zero, we get a quadratic equation.
Its general form is a2 + bx + c = 0, where a, b and c are real numbers and a ≠ 0.
In many real life situations we deal with quadratic equations.
Suppose, we have to make a table of 50m2 area with its length twice as its breadth then,

Let x be the breadth of the table Therefore, its length will be 2x
Since, length × breadth = area
Therefore, x.2x = 50
So, 2x2 = 50 (quadratic equation)
x2 =25 that gives, x = 5
Thus the length of that table will be = 2x = 10m and breadth will be 5m.
EXERCISE – 4.1
Represent the following statements mathematically:
Q.1 Rahul and Nikhil together have 50 chocolates. Both of them lost 5 chocolates each and the product of number of chocolates they have now is 300.Find how many chocolates they actually had?
Sol.
Let, x be the number of chocolates Rahul had Then, the total number of chocolates Nikhil had = (50 – x) chocolates.
After loosing 5 chocolates Rahul had (x – 5) chocolates and Nikhil had (50 – x – 5) chocolates.
Now, according to the given condition:
(x – 5) (45 – x) = 300
45x – x2 – 225 + 5x=300
x2 – 50x + 525 = 0
This is the required quadratic equation.
Q2. Check whether (x – 7)2 +4 = 2x – 9 is a quadratic equation.
Sol.
On, simplifying the above equation we get:
x2 + 49 – 14x + 4 = 2x – 9
x2 – 16x + 62=0
General form of quadratic equation is ax2 + bx + c = 0
Therefore, the given equation is a quadratic equation.
Q.3 Check whether x(x-7) + 3 = (x + 6) (x – 6) is a quadratic equation.
Sol.
On, simplifying the above equation we get:
x2 – 7x + 3 = x2 – 36
7x – 33 = 0
This is not a quadratic equation because general form of a quadratic equation is ax2+bx+c=0 with a ≠ 0 and in this equation a = 0.
Q.4 Check whether (x – 2)3 = 0 is a quadratic equation.
Sol.
On, simplifying the above equation we get:
x3 – 8 – 6x2+12x = 0.
Degree of this polynomial equation is 3,
Thus, it is a cubic polynomial equation not a quadratic equation.
Q.5 Rahul’s father is 27 years older than him. 3 years from now the product of their ages will be 1224. We would like to find Rohan’s present age.
Sol.
Let the present age of Rahul be x years
Then, present age of Rahul’s father will be = (x + 27) years
Now, their ages after 3 years:
Rahul’s age = (x + 3) years
Rahul’s father age = x + 27 + 3 = (x + 30) years
According to given condition:
(x+3) (x+30) = 1224
x2 + 30x +3x +90 –1224 = 0
x2 + 33x – 1134 = 0. (Where x is the present age of Rahul in years)
This is the required quadratic equation.
Q.6 There is a rectangular park of area 860m 2. The length (meters) of the park is three more than twice its breadth (meters). Form a quadratic equation to solve this problem.
Sol.
Let, x be length (meters) and y be breadth (meter) of the rectangular plot
Now, according to given condition:-
x = 3 + 2y ……… (1)
And
x.y = 860m2…… (2)
Now, substituting equation (1) in (2) we get
(3 + 2y) y = 860
2y2 + 3y = 860
2y2 + 3y – 860 = 0 where y = breadth (in meters)
This is the required quadratic equation.
Q.7 A train is covering a distance of 540 km from one city to another city at a uniform speed. If the speed of train had been reduced by 6 km/h, then to cover the same distance the train would have taken 1 hour more. Form a quadratic equation of this situation.
Sol.
Let the train travels at the uniform speed of x km/hr.
Therefore,
Time taken to cover 540km = 540xhours
Now,
When speed is reduced by 6km/h then time taken to cover the same distance = 540x−6hours
Now, according to given condition:
540x−6−540x=1
540x – 540 ( x – 6 ) = x ( x – 6 )
x2 – 6x – 3240 = 0. Where x= speed of train in km/h
This is the required quadratic equation.
Finding roots of quadratic equation by factorization
Exercise 4.2
Q1. Find the roots of quadratic equation by factorization:
x2−(3–√+2–√)x+6–√ = 0
Sol.
x2−3–√x−2–√x+6–√=0
x(x−3–√)−2–√(x−3–√)=0
(x−3–√)(x−2–√)=0
Therefore x=2–√ or x=3–√
Q2. Find the roots of quadratic equation:
10x2−73–√x+3=0
Sol.
10x2−73–√x+3=0
x2−73√x10+310=0
x2−(3√2+3√5)x+310=0
x2−3√2x−3√5x+310=0
x(x−3√2)−3√5(x−3√2)=0
(x−3√2)(x−3√5)=0
Therefore x=3√2 or x=3√5
Q3. Find the roots of quadratic equation:
400x2 – 40x + 1 = 0
Sol.
Since, 400x2 – 40x + 1 = 0
Therefore, x2−110x+1400=0
x2−110x+1400=0
x2−(120+120)x+1400=0
x2−120x−120x+1400=0
x(x−120)−120(x−120)
(x−120)(x−120)
Therefore x=120 or x=120
Q4. Find the roots of quadratic equation:
x2– 16x + 63 = 0
Sol.
Since, x2– 16x + 63 = 0
Therefore, x2– (9+7)x + 63 = 0
x2– 9x-7x + 63 = 0
x(x – 9) – 7( x – 9 ) = 0
(x -9) ( x – 7 ) = 0
Therefore, x = 9 or x = 7
Q5. Find the roots of quadratic equation:
3–√x2+7x+23–√=0
Sol.
3–√x2+7x+23–√=0
x2+73√x+2=0
x2+63√x+13√x+2=0
x(x+63√)+13√(x+23–√)=0
(x+13√)(x+23–√)=0
Therefore x=−13√ or x=−23–√
Q.6 Product of two numbers x and y is 144 and their sum is 25. Find x and y.
Sol.
According to given condition:
x . y = 144 ……………………….. (1)
And,
x + y = 25 ……………………………. (2)
Substituting equation (2) in (1) we get:
x(25 – x) = 144
(or)
x2 – 25x + 144= 0
x2 – (16 + 9)x + 144= 0
x2 – 16x – 9x + 144= 0
x(x – 16) – 9(x – 16) = 0
Therefore, x = 16 or x = 9
So, if first number (x) is 16 then second number (y) will be (25 -16) = 9
(or)
If first number is(x) 9 then second number(y) will be (25-9) = 16.
Q7. Sum of square of two consecutive positive integers is 481, find the numbers.
Sol.
Let, 1st number be x
Therefore, second number will be (x+1)
According to the given condition:
x2 + (x + 1)2 = 481
x2 + x2 + 2x + 1 = 481
2x2 + 2x – 480 = 0
x2 + x – 240 = 0
x2 + (16 – 15) x – 240 = 0
x2 + 16x – 15x – 240 = 0
x(x + 16) – 15(x + 16) = 0
(x – 15) (x + 16) = 0
Therefore,
x= 15 (or) x= -16
Since, the positive integers can’t be -16
Hence two consecutive positive integers are 15 and 16.
Q.8 The base of a right angled triangle is 3cm more than its altitude. If the hypotenuse is 15cm. Find the length of its base and altitude.
Sol.
In right angled triangle ABC:

Let, length of altitude = x cm
Therefore base = (x + 3) cm
Now, according to the given condition:
(AB)2 + (AC)2 = (BC)2 (From Pythagoras theorem)
(x)2 + (x+3)2 = (15)2
x2 + x2 +6x + 9 = 225
2x2 + 6x – 216 = 0
x2 + 3x – 108 = 0
This is required quadratic equation, on further solving this equation:
x2 + 3x – 108 = 0
x2 + (12 – 9)x – 108 = 0
x2 + 12x – 9x – 108 = 0
x(x + 12) – 9(x + 12) = 0
(x + 12) (x – 9) = 0
Therefore, x = -12 (or) x = 9
Since length cannot be negative. Therefore, neglecting -12cm
Hence the length of altitude = 9 cm and length of the base = 12cm
Finding roots by method of completing the square
Equations like x2 – 4x – 8 = 0, cannot be solved by method of factorization because it doesn’t factor.
These types of equations can be solved by method of completing the square.
i.e x2 – 4x – 8 = 0 or x2 – 4x = 8
Our main target is to convert x2 – 4x in form (x – a)2 which is equal to x2 – 2ax + a2.
So, we will try to break the middle term in such a way, that 4x can be written in form of 2ax.
i.e 4x= 2(2.x) thus, here a = 2.
Therefore, if we will add 22 on both LHS and RHS, we get the following equation:-
x2 – 2(2x) + 22 = 8 +22
(or), (x – 2)2 = 12
(x – 2)2 = 12−−√
(x – 2)2 – (12−−√)2= 0
Since, a2−b2=(a+b)(a–b)
Therefore, (x−2−12−−√) (x−2+12−−√)
x=2+12−−√ or x=2−12−−√
This is how we find roots of a given equation by method of completing the square.
Exercise – 4.3
Q.1 Find the roots of the equation 25x2 + 20x – 13 = 0 by method of completing the square.
Sol.
(5x)2 + 20x = 13
(5x)2 + 2(5x.2)+ 22 = 13+22
(5x+2)2 – 17 = 0
Therefore (5x+2)2−(17−−√)2=0
(5x+2+17−−√) (5x+2−17−−√)=0
Therefore x=−2+17√5 or x=−2+17√5
Q.2 Find the roots of the equation 9x2 + 12x – 5 = 0 by method of completing the square.
Sol.
9x2 + 12x = 5
(3x)2 + 2(3x.2) = 5
(3x)2 + 2(3x.2) + 22 = 5 + 22
(3x+2)2 = 9
(3x+2)2 – 32 = 0
(3x + 2 + 3) (3x + 2 – 3) = 0
(3x + 5) (3x – 1) = 0
Therefore x=−53 or x=13
Q.3 Find the roots of the equation 3x2 – 16x + 5 = 0 by method of completing the square.
Sol.
To solve the above equation either divide the entire equation by 3 to make 3x2 a perfect square but it will be much easier to solve the equation if we multiply the above equation by 3 to make 3x2 a perfect square.
Therefore, 9x2 – 48x = -15
(3x)2 – 2(3x.8) =-15
(3x)2 – 2( 3x.8 ) + 82 = -15 + 82
(3x – 8)2 – 49 = 0
(3x-8)2 – 72 = 0
(3x-8+7)(3x-8-7)=0
(3x-1)(3x-15)=0
Therefore x=13 or x=5
Q.4 Find the roots of the equation 2x2 + x – 4 = 0 by method of completing the square.
Sol.
Now, for making 2x2 a perfect square, multiplying the above equation by 2
Therefore,
4x2 + 2x = 8
Now,
(2x)2+2(2x.12)=8
(2x)2+2(2x.12)+14=8+14
(2x+12)2−(334−−√)2=0
(2x+12+33√2)(2x+12−33√2)=0
Therefore x=−1+33√4 or x=−1+33√4
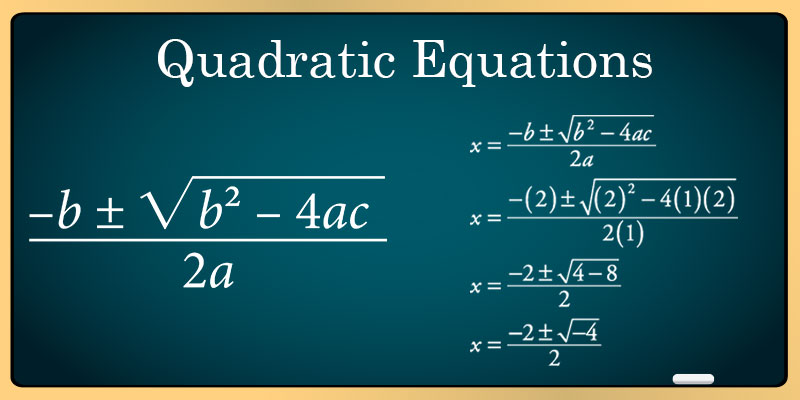
Consider a quadratic equation:
ax2 + bx+ c = 0 with a ≠ 0
The roots of the following quadratic equation are given by:
x=−b+b2−4ac√2a and x=−b−b2−4ac√2a
(or) x=−b±b2−4ac√2a
This is known as quadratic formulae for finding roots of quadratic equation.
Nature of roots:
The term (b2 – 4ac) is known as discriminant and it determines the nature of roots of the quadratic equation.
If b2 – 4ac > 0, then the equation will have two distant real roots.
If b2 – 4ac = 0, then the equation will have two equal real roots.
If b2 – 4ac < 0, then the equation will have unreal roots.
Exercise 4.4
Q.1 Find the roots of following equation:
x+1x=5, with x ≠ 0.
Sol.
On simplifying the above equation we get:
x2 – 5x + 1 = 0
Here, a=1, b = – 5 and c = 1.
On putting the values of a, b and c in quadratic formulae we get:
x=−(−5)+(−5)2−4(1×1)√2×1 and x=−(−5)−(−5)2−4(1×1)√2×1
x=5+25−4√2 and x=5−25−4√2
x=5+21√2 and x=5−21√2
Q.2 Find the roots of following equation:
1x−1x−3=7, where x≠0,3
Sol.
On simplifying the above equation we get:
7x2 – 21x + 3 = 0
Here, a = 7, b = -21 and c = 3
On putting the values of a, b and c in quadratic formulae we get:
x=−(−21)+(−21)2−4(7×3)√2×7 and x=−(−21)−(−21)2−4(7×3)√2×7
x=21+441−84√14 and x=21−441−84√14
x=21+357√14 and x=21−357√14
Q.3 Find the nature of roots of the following quadratic equation:-
7x2 + x + 2 = 0
Sol.
Here, a = 7, b = 1 and c=2
Since, discriminant (D) = b2 – 4ac ………….. (1)
On putting the values of a, b and c in equation (1) we get: –
D = 12 – 4(7×2)
Therefore D = -55
Since, D < 0. This equation will have no real roots.
Q.4 Find the values of k in the following quadratic equation so that they have two equal real roots 3x2 + kx + 5 = 0
Sol.
Given, 3x2 + kx + 5 = 0
Here a = 3, b = k and c = 5
Now, If b2 – 4ac = 0, then we will get two equal real roots.
Therefore,
k2 – 4×3×5 = 0
k2 = 60
Therefore for equal roots k= 215−−√
Q.5 Find the values of k in the following quadratic equation so that they have two equal real roots.
kx(x – 9) + 3 = 0
Sol.
On simplifying the given equation:
kx2 – 9kx +3 = 0
Here a = k, b = -9k and c = 3
Now, If b2 – 4ac = 0, then we will get two equal real roots.
Therefore,
(-9k)2 – 4(k×3) = 0
81k2 – 12k = 0
(or)
k(81k – 12) = 0
Since value of k cannot be 0.
Therefore for equal roots k= 1281
Q.6 Find the nature of roots of the following quadratic equation, if real roots exist, Find them.
2x2 + x – 4 = 0
Sol.
Here a = 2, b = 1 and c = – 4
Since,
D = b2 – 4ac
Therefore,
D= 12 – 4(- 4 × 2)
D= 1 + 32
Therefore, D= 33
Since, D > o. The given quadratic equation will have real roots.
On putting the values of a, b and c in the quadratic formulae we get:
x=−(1)+(1)2−4(2×−4)√2×2 and
x=−(1)−(1)2−4(2×−4)√2×2
x=−1+1+32√4 and x=−1−1+32√4
x=−1+33√4 and x=−1−33√4
Q.7 Deepak’s father is 27 years older than him. 3 years from now the product of their ages will be 1224. Find the present age of Deepak.
Sol.
Let the present age of Deepak be x years
Then, present age of Deepak’s father will be = (x + 27) years
Now, their ages after 3 years: –
Deepak’s age = (x + 3) years
Deepak’s father age = x + 27 + 3 = (x + 30) years
According to given condition,
(x+3) (x+30) = 1224
x2 + 30x +3x +90 –1224 = 0
x2 + 33x – 1134 = 0 (Where x is the present age of Deepak in years)
This is the required quadratic equation.
Here, a = 1, b = 33 and c = -1134
On putting the values of a, b and c in the quadratic formulae we get:
x=−(33)+(33)2−4(1×−1134)√2×1 and x=−(33)−(33)2−4(1×−1134)√2×1
x=−33+1089+4536√2 and x=−33−1089+4536√2
x=−33+5625√2 and x=−33−5625√2
x=−33+752 and x=−33−752
Therefore x=21 and x= -54
Since, age cannot be negative. Therefore neglecting x = -54
Thus the present age of Deepak = x = 21 years
And the present age of Deepak’s father will be = (x + 27) = 48 years
Q.8 There is a rectangular park of area 860m 2. The length (meters) of the park is three more than twice its breadth (meters). Find the length and breadth of this rectangular park.
Sol.
Let, x be length (meters) and y be breadth (meter) of the rectangular plot
Now, according to given condition:
x = 3 + 2y ……… (1)
And
x.y = 860m2…… (2)
Now, substituting equation (1) in (2) we get
(3 + 2y) y = 860
2y2 + 3y = 860
2y2 + 3y – 860 = 0 where y = breadth (in meters)
This is the required quadratic equation.
Here, a = 2, b = 3 and c = -860
On putting the values of a, b and c in the quadratic formulae we get:
y=−(3)+(3)2−4(2×−860)√2×2 and y=−(3)−(3)2−4(2×−860)√2×2
y=−3+9+6880√4 and y=−3−9+6880√4
y=−3+6889√4 and y=−3−6889√4
y=−3+834 and y=−3−834
Therefore y=20 and y= -21.5
Since, length cannot be negative. Therefore neglecting y = -21.5
Thus breadth of the rectangular park = y = 20m
And length of the rectangular park, x = (3 + 2y) = 43m
Q.9 A train is covering a distance of 540km from one city to another city at a uniform speed. If the speed of train had been reduced by 6km/h, then to cover the same distance the train would have taken 1hour more. Find the uniform speed of train.
Sol.
Let the train travels at the uniform speed of x km/hr
Therefore,
Time taken to cover 540km = 540x hrs
Now,
When speed is reduced to 6km/h then time taken to cover the same distance = 540x−6 hrs
Now, according to given condition:
540x−6−540x=1
540x – 540 ( x – 6 ) = x ( x – 6 )
x2 – 6x – 3240 = 0 Where x= speed of train in km/h
This is the required quadratic equation.
Here, a = 1, b = -6 and c = -3240
On putting the values of a, b and c in the quadratic formulae we get:
x=−(−6)+(−6)2−4(1×−3240)√2×1 and x=−(−6)−(−6)2−4(1×−3240)√2×1
x=6+36+12960√2 and x=6−36+12960√2
x=6+12996√2 and x=6−12996√2
x=6+1142 and x=6−1142
Therefore x=60 and x= -54
Since, speed cannot be negative. Therefore neglecting x = -54
Thus, the uniform speed of train = x = 60km/h.
Q.10 A chocolate factory produces a certain number of chocolates in a day. It was observed that on a particular day the cost of production of each chocolate (in rupees) was 5 more than thrice the number of chocolates produced on that day. If total cost of production on that day was 750 rupees. Find the number of chocolates produced and the cost of each chocolate.
Sol.
Let x be number of chocolates produced and y be the cost of each chocolate.
Now, According to given conditions:
y = 5 + 3x…………………. (1)
And
x.y = 750 ……………. (2)
Substituting values of equation (1) in equation (2) we get:
x(3x + 5) = 750
3x2 + 5x – 750 = 0
This is the required quadratic equation.
Here, a = 3, b = -5 and c = -750
On putting the values of a, b and c in the quadratic formulae we get:
x=−(−5)+(−5)2−4(3×−750)√2×3 and x=−(−5)−(−5)2−4(3×−750)√2×3
x=5+25+9000√6 and x=5−25+9000√6
x=5+9025√6 and x= 5−9025√6
x=5+956 and x=5−956
Therefore x=503 and x= -15
Since, number of chocolates cannot be negative. Therefore neglecting x = -15
Hence number of chocolates produced = x = 503 chocolates
And cost of each chocolate = y = (5 + 3x) = 55 Rs
Q.11 Find two consecutive even positive integers, whose product is 168.
Sol.
Let x be the first number, then second number will be (x + 2).
Now, according to the given condition:
x(x + 2) = 168
(or) x2 + 2x -168 = 0
Here a =1, b = 2 and c = -168
Now, substituting the values of a, b and c in quadratic formulae we get:
x=−(2)+(2)2−4(1×−168)√2×1 and x=−(2)−(2)2−4(1×−168)√2×1
x=−2+4+672√2 and x=−2−4+672√2
x=−2+262 and x=−2−262
Therefore x=12 and x= -14
Since x can’t be negative, therefore neglecting x = -14.
Therefore first number= x = 12 and second number = (x + 2) = 14
Q.12 Find two consecutive even positive integers, sum of whose squares is 100.
Sol.
Let x be the first number, then second number will be (x + 2)
Now, According to the given condition:
x2 + (x+2)2 = 100
x2 + x2 + 4 + 4x = 100
2x2 + 4x – 96 = 0
x2 + 2x – 48 = 0
Here, a =1, b = 2 and c = -48
Now, substituting the values of a, b and c in quadratic formulae we get:
x=−(2)+(2)2−4(1×−48)√2×1 and x=−(2)−(2)2−4(1×−48)√2×1
x=−2+4+192√2 and x=−2−4+192√2
x=−2+142 and x=−2−142
Therefore x=6 and x= -8
Since x can’t be negative, therefore neglecting x = -8
Therefore first number = x = 6 and second number = (x + 2) = 8
Q.13 It takes 105mints more for a motor boat to go 35km upstream than to return downstream at the same spot. If speed of a motor boat in still water is 21 km/h. Find speed of the stream.
Sol.
Since 60mints = 1 hour
Therefore 105mints = 10560 hours
Let the speed of stream be x km/h
Therefore, speed of boat in upstream = (21-x) km/h
And speed of boat in downstream = (21+x) km/h
Since time=distancespeed
Therefore time taken by motorboat to go upstream = 3521−xhours
And time taken by motorboat to go downstream = 3521+xhours
Now, according to the given condition:
3521−x−3521+x=10560
35(21+x−21+x212−x2)=74
2x441−x2=74×35
40x=441−x2
x2+40x−441=0
Now, from the above quadratic equation:- a = 1, b = 40 and c = -441
Substituting the values of a, b and c in quadratic formulae we get:
x=−(40)+(40)2−4(1×−441)√2×1 and x=−(40)−(40)2−4(1×−48)√2×1
x=−40+1600+1764√2 and x=−40−1600+1764√2
x=−40+3364√2 and x=−40−3364√2
x=−40+582 and x=−40−582
Therefore x =9 and x = -49
Since distance can’t be negative, therefore neglecting x = -49.
Therefore, the speed of stream = 9 km/h.
Q.14 If the difference in the parameter of two squares is 32m and sum of the areas of both squares is 544m2. Find the sides of both the squares.
Sol.
Let x be the side of 1st square and y be the side of 2nd square.
Now, according to the given conditions: –
4x – 4y = 32
Or, x – y = 8 …..………………………….. (1)
And
x2 + y2 = 544 …………………………… (2)
Now, substituting equation (1) in equation (2) we get:
(8 – y)2 + y2 = 544
y2 + 64 – 16y + y2 = 544
2y2 -16y – 480 = 0
Or, y2 – 8y – 240 = 0
Now, from the above quadratic equation:- a = 1, b = -8 and c = -240
Substituting the values of a, b and c in quadratic formulae we get:
y=−(−8)+(−8)2−4(1×−240)√2×1 and y=−(−8)−(−8)2−4(1×−240)√2×1
y=+8+64+960√2 and y=+8−64+960√2
y=8+1024√2 and y=8−1024√2
y=8+322 and y=8−322
Therefore y=20 and y= -12
Since distance can’t be negative, therefore neglecting y = -12.
Therefore, The side of 2nd square = y = 20m
And the side of 1st square = (8 + y) = 28m
Q.15 If the average speed of an express train is 15km/h more than that of a passenger train and for covering a distance of 150km between two stations A and B an express train takes 97hours less than a passenger train. Find the average speed of train A and train B.
Sol.
Let x km/h be the average speed of a passenger train.
Therefore, Average speed of an express train will be (x + 15) km/h.
Now, time taken by an express train to cover 150km = 150x+15 hours
And, time taken by a passenger train to cover the same distance = 150x hours
Now, according to the given condition:
150x – 150x+15= 97
150(x+15−x)x(x+15)=97
150×15×7=9(x2+15x)
50×5×7=x2+15x
Therefore x2+15x−1750=0
Now, from the above quadratic equation:- a = 1, b = 15 and c = -1750
Substituting the values of a, b and c in quadratic formulae we get:
x=−(15)+(15)2−4(1×−1750)√2×1 and x=−(15)−(15)2−4(1×−1750)√2×1
x=−15+225+7000√2 and x=−15−225+7000√2
x=−15+7225√2 and x=−15−7225√2
x=−15+852 and x=−15−852
Therefore x=35 and x= -50
Since speed can’t be negative, therefore neglecting x = -50.
Therefore, speed of passenger train= x = 35km\h.
Andspeed of express train= (x + 15) = 50km\h.
Q.16 Two water taps with different diameters are used to fill a particular tank, both the taps together can fill a tank in 245hours.
However, if they are operated separately, tap with larger diameter can fill the tank 4 hours early as compared to the tap with smaller diameter. Find the time in which each tap can separately fill the tank.
Sol.
Let, x hours be the time taken by the tap with smaller diameter to fill a tank separately.
Thus, in 1 hour it can fill 1x part of the tank.
Let, the time taken by the tap with larger diameter to fill a tank separately is (x-4) hours.
Thus, in 1 hour it can fill 1x−4 part of the tank.
Both the taps together can fill tank in 245hours.
Therefore in 1hour both the taps can fill 524 part of the tank.
Now, according to the given condition:
1x+1x−4=524 x−4+xx(x−4)=524 48x−965=x2−4x
5x2−68x+96=0
Now, from the above quadratic equation:- a = 5, b = -68 and c = 96
Substituting the values of a, b and c in quadratic formulae we get:
x=−(−68)+(−68)2−4(5×96)√2×5 and x= −(−68)−(−68)2−4(5×96)√2×5
x=68+4624−1920√10 and x=68−4624−1920√10
x=68+2704√10 and x=68−2704√10
x= 68+5210 and x=68−5210
Therefore x=12 and x= 1.6
When x = 1.6, It doesn’t satisfy the given conditions, therefore neglecting x = 1.6
Therefore the time taken by the tap with smaller diameter to fill the tank separately = x = 12 hours
And the time taken by the tap with larger diameter to fill the tank separately = (x – 4) = 8 hours
Q.17 There is a requirement of a rectangular park of area 575m2 and perimeter 100m. Check whether it’s possible or not? If possible then find length and breadth of that park.
Sol.
Let x be length of the park and y be breadth of the park in meters.
Now, according to the given conditions:
2(x + y) = 100
(or)
x + y = 50 ……………………… (1)
And
x.y = 575 ……………………. (2)
Now, substituting equation (1) in equation (2) we get:
(50 – y)y = 575
y2 -50y +575=0
Now, from the above quadratic equation: a = 1, b = -50 and c = 575
Since, D=b2−4(ac)
Therefore, D=32−4(1×−108)
Therefore D=441
Since, D≥0, Therefore the above quadratic equation will have real roots and hence the given situation is practically possible.
Now, from the quadratic formulae:
x=−(3)+(3)2−4(1×−108)√2×1 and x=−(3)−(3)2−4(1×−108)√2×1
x=−3+9+432√2 and x=−3−9+432√2
x=−3+441√2 and x=−3−441√2
x=−3+212 and x=−3−212
D=b2−4ac
Therefore D = (−50)2−4(1×575)
Therefore D= 200
Since, D≥0 Therefore The above quadratic will have real roots and hence it is possible to design a park with given conditions.
Now,
y=−(−50)+(−50)2−4(1×575)√2×1 and y=−(−50)−(−50)2−4(1×575)√2×1
y=50+2500−2300√2 and y=50−2500−2300√2
y=50+200√2 and y= 50−200√2
y=50+102√2 and y=50−102√2
Therefore y=25+52–√ and y=25−52–√
Now,
If breadth of park = y=25+52–√m
Then, Length of park = (50 – y) =x=25–52–√m
And If breadth of park = y=25−52–√m
Then Length of park = (50 – y) = x=25+52–√m
Q.18 There is a circular park with diameter equal to 15m having two gates one for entry and other for exit fixed at diametrically opposite ends. A plant has to be planted on the boundary of a circular park in such a way that the difference of its distance from two opposite fixed gates P and Q from boundary is 3 metres.
Check whether the above give situation is possible or not?
And if it is possible to do so, then find the distance of plant from both the gates P and Q.
Sol.

Let P be the location of the plant, A and C represents the location of gates of the park.
Now, According to the given condition:
PC – AP = 3
PC = 3 + AP
= 3 + x
Therefore, AC2 = AP2 + PC2 (Pythagoras Theorem)
152 = x2 + (x + 3)2
225 = x2+x2+9+6x
2x2 + 6x – 216 = 0
(or) x2 + 3x – 108 = 0
Now, from the above quadratic equation a = 1, b =3 and c = -108
Since,D=b2−4(ac)
Therefore D=32−4(1×−108)
Therefore D=441
Since, D≥0, Therefore the above quadratic equation will have real roots and hence the given situation is practically possible.
Now, from the quadratic formulae:
x=−(3)+(3)2−4(1×−108)√2×1 and x=−(3)−(3)2−4(1×−108)√2×1
x=−3+9+432√2 and x=−3−9+432√2
x=−3+441√2 and x=−3−441√2
x=−3+212 and x=−3−212
Therefore x=9 and x= -12
Since, the distance cannot be negative therefore neglecting x= -12
Therefore, AP = x =9m and PC = (x+3) = 12m.
Q.19 Find the value of k, if one root of the quadratic equation k2x2 – 21x + k + 9 = 0 is 1.
Sol.
From the given quadratic equation: – a= k2, b= -21 and c= (k + 9)
Let the other root of this quadratic equation be β
Therefore, sum of their roots = 1 + β = −ba =21k2
1+β=21k2
Therefore β=21−k2k2…………(1)
And product of their roots = 1 × β = ca = k+9k2
Therefore β=k+9k2…………(2)
Now, from equation (1) and equation (2) we get:
k+9k2=21−k2k2
Therefore k2+k−12=0
Now, from the above quadratic equation: a = 1, b = 1 and c = -12
Substituting the values of a, b and c in quadratic formulae we get:
k=−(1)+(1)2−4(1×−12)√2×1 and k=−(1)−(1)2−4(1×−12)√2×1
k=−1+1+48√2 and k=−1−1+48√2
k=−1+49√2 and k=−1−49√2
k=−1+72 and k=−1−72
k=3 and k= -4.
Therefore, the value of k is 3 or -4
Q.20 Determine the nature of roots of equation: 2x2 + 3x + 9 = 0
Sol.
From the given equation a = 2, b = 3 and c = 9.
Since, D = b2 – 4ac
Therefore, D = 33 – 4(9×2)= -63
Since D < 0, the given equation has no real roots.
Q.21 If the ratio of roots of quadratic equation kx2 + 18x + 15 = 0 is 1:5. Find the possible values of k.
Sol.
From the given quadratic equation: a = k, b = 18 and c = 15
Since, roots of the given quadratic equation are in ratio 1:5
Therefore 1st root will be α and 2nd root will be 5α.
Now, sum of roots = α + 5α = −ba= −18k
Therefore, 6α=−18k
Therefore α=−3k ……………….(1)
And product of roots = α × 5α = ca=15k
Therefore α2=3k………… (2)
Now, on substituting equation (1) in equation (2) we get:
(−3k)2=3k
Therefore, k = 2